All in one.
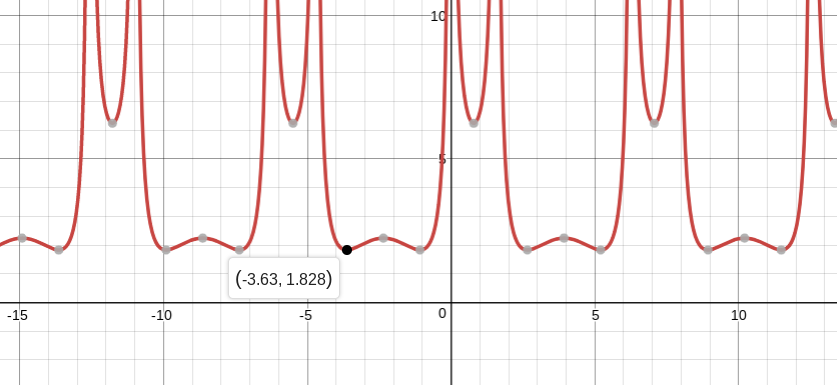
Find the absolute minimum value of the following function:
∣ sin x + cos x + tan x + cot x + sec x + csc x ∣
The answer is 1.828.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
I have absolutely no idea how to solve this algebraically...
Log in to reply
You take sin x + cos x = t . Then, tan + cot becomes t 2 − 1 2 and sec + csc becomes t 2 − 1 2 t . It gets reduced to a simple function whose range can be calculated, the only condition is that − √ 2 < t < √ 2