Almost Isoceles
A B = B C = C D , m ∠ A B C = 7 5 ∘ , m ∠ B C D = 1 6 5 ∘ . Find m ∠ E , to the nearest tenth of a degree.
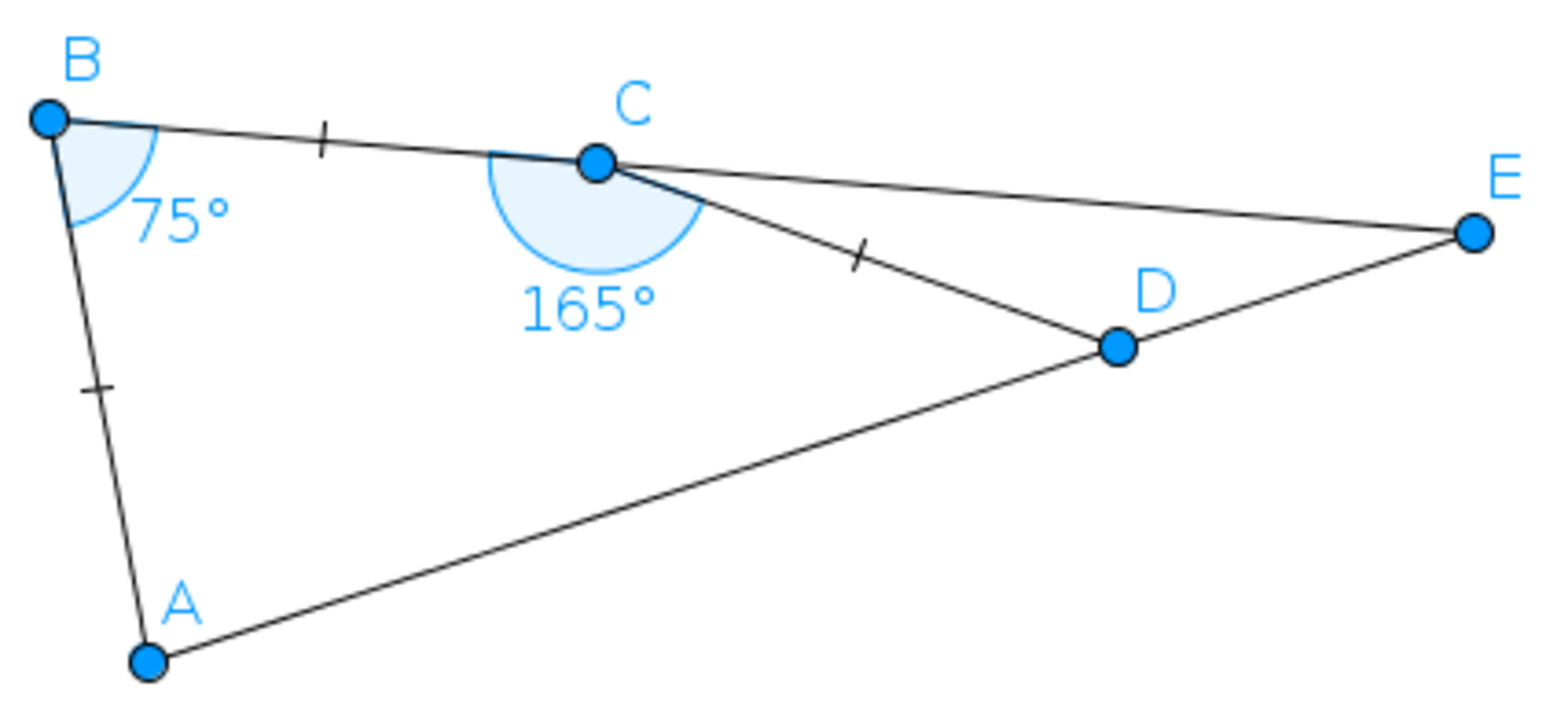
(Illustration not necessary to scale)
The answer is 22.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Connect A C and observe that △ A B C is isosceles, which implies that ∠ B C A = ∠ B A C = 5 2 . 5 ∘ . Applying the cosine rule to △ A B C , we find that A C = 2 − 2 cos 7 5 ∘
Now, note that ∠ A C D = 1 6 0 ∘ − 5 2 . 5 ∘ = 1 1 2 . 5 ∘ . Applying the cosine rule to △ A C D , we find that A D = A C 2 + 1 − 2 A C cos 1 1 2 . 5 ∘ = 1 . 8 4 7 7 6 . . .
Apply the sine rule to △ A C D . sin 1 2 2 . 5 ∘ A D = ∠ C A D 1 ⇒ ∠ C A D = 3 0 ∘
Therefore, ∠ E = 1 8 0 ∘ − 1 1 2 . 5 ∘ − 3 0 ∘ − 1 5 ∘ = 1 2 . 5 ∘
The trick here is constructing equilateral △ A B F .
m ∠ F B C + m ∠ B C D = 1 5 ° + 1 6 5 ° = 1 8 0 ° and F B = B C = C D
⇒ B C D F is a rhombus
⇒ B C ∥ D F and △ A F D is isoceles
⇒ m ∠ E = m ∠ A D F = 9 0 ° − 2 1 m ∠ A F D = 9 0 ° − 2 1 ( 3 6 0 ° − 6 0 ° − 1 6 5 ° ) = 2 2 . 5 °