Amazing Trigonometry_4

Techniques used:

• Double angle identities for sine
• Product-to-sum trigonometry formulas for $\sin A\sin B$ and $\cos A\cos B$
• Introducing extra terms in a clever way

Since $\tan\frac{8\pi}{7}=\tan\frac{\pi}{7}$ , the expession is equivalent to $\tan\frac{\pi}{7}\tan\frac{2\pi}{7}+\tan\frac{2\pi}{7}\tan\frac{4\pi}{7}+\tan\frac{4\pi}{7}\tan\frac{\pi}{7}$

Consider $\tan\frac{\pi}{7}\tan\frac{2\pi}{7}$ , $\begin{aligned}\tan\frac{\pi}{7}\tan\frac{2\pi}{7}&=\frac{\sin\frac{\pi}{7}\sin\frac{2\pi}{7}}{\cos\frac{\pi}{7}\cos\frac{2\pi}{7}} \\ &=\frac{(\cos\frac{\pi}{7}-\cos\frac{3\pi}{7})\cos\frac{4\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{\cos\frac{4\pi}{7}\cos\frac{\pi}{7}-\cos\frac{4\pi}{7}\cos\frac{3\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{(\cos\frac{5\pi}{7}+\cos\frac{3\pi}{7})-(\cos\pi+\cos\frac{\pi}{7})}{4\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \end{aligned}$

Similarly: $\begin{aligned}\tan\frac{2\pi}{7}\tan\frac{4\pi}{7}&=\frac{\sin\frac{2\pi}{7}\sin\frac{4\pi}{7}}{\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{(\cos\frac{2\pi}{7}-\cos\frac{6\pi}{7})\cos\frac{\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{\cos\frac{\pi}{7}\cos\frac{2\pi}{7}-\cos\frac{\pi}{7}\cos\frac{6\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{(\cos\frac{3\pi}{7}+\cos\frac{\pi}{7})-(\cos\pi+\cos\frac{5\pi}{7})}{4\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \end{aligned}$ $\begin{aligned}\tan\frac{\pi}{7}\tan\frac{4\pi}{7}&=\frac{\sin\frac{\pi}{7}\sin\frac{4\pi}{7}}{\cos\frac{\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{(\cos\frac{3\pi}{7}-\cos\frac{5\pi}{7})\cos\frac{2\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{\cos\frac{3\pi}{7}\cos\frac{2\pi}{7}-\cos\frac{5\pi}{7}\cos\frac{2\pi}{7}}{2\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{(\cos\frac{5\pi}{7}+\cos\frac{\pi}{7})-(\cos\pi+\cos\frac{3\pi}{7})}{4\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \end{aligned}$ All them all up, some terms cancel, we are left with $\begin{aligned} \tan\frac{\pi}{7}\tan\frac{2\pi}{7}+\tan\frac{2\pi}{7}\tan\frac{4\pi}{7}+\tan\frac{4\pi}{7}\tan\frac{\pi}{7}&=\frac{\cos\frac{\pi}{7}+\cos\frac{3\pi}{7}+\cos\frac{5\pi}{7}+3}{4\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{2(\cos\frac{\pi}{7}+\cos\frac{3\pi}{7}+\cos\frac{5\pi}{7}+3)\sin\frac{\pi}{7}}{8\sin\frac{\pi}{7}\cos\frac{\pi}{7}\cos\frac{2\pi}{7}\cos\frac{4\pi}{7}} \\ &=\frac{2\sin\frac{\pi}{7}\cos\frac{\pi}{7}+2\cos\frac{3\pi}{7}\sin\frac{\pi}{7}+2\cos\frac{5\pi}{7}\sin\frac{\pi}{7}+6\sin\frac{\pi}{7}}{\sin\frac{8\pi}{7}} \\ &=\frac{\sin\frac{2\pi}{7}+(\sin\frac{4\pi}{7}-\sin\frac{2\pi}{7})+(\sin\frac{6\pi}{7}-\sin\frac{4\pi}{7})+6\sin\frac{\pi}{7}}{\sin\frac{8\pi}{7}} \\ &=\frac{\sin\frac{6\pi}{7}+6\sin\frac{\pi}{7}}{\sin\frac{8\pi}{7}} \\ &=\frac{\sin\frac{\pi}{7}+6\sin\frac{\pi}{7}}{-\sin\frac{\pi}{7}} \\ &=-\frac{7\sin\frac{\pi}{7}}{\sin\frac{\pi}{7}} \\ &=-7 \end{aligned}$

There is a very neat way to do this problem almost mentally. (If you are comfortable with evaluating trig products in your head.)

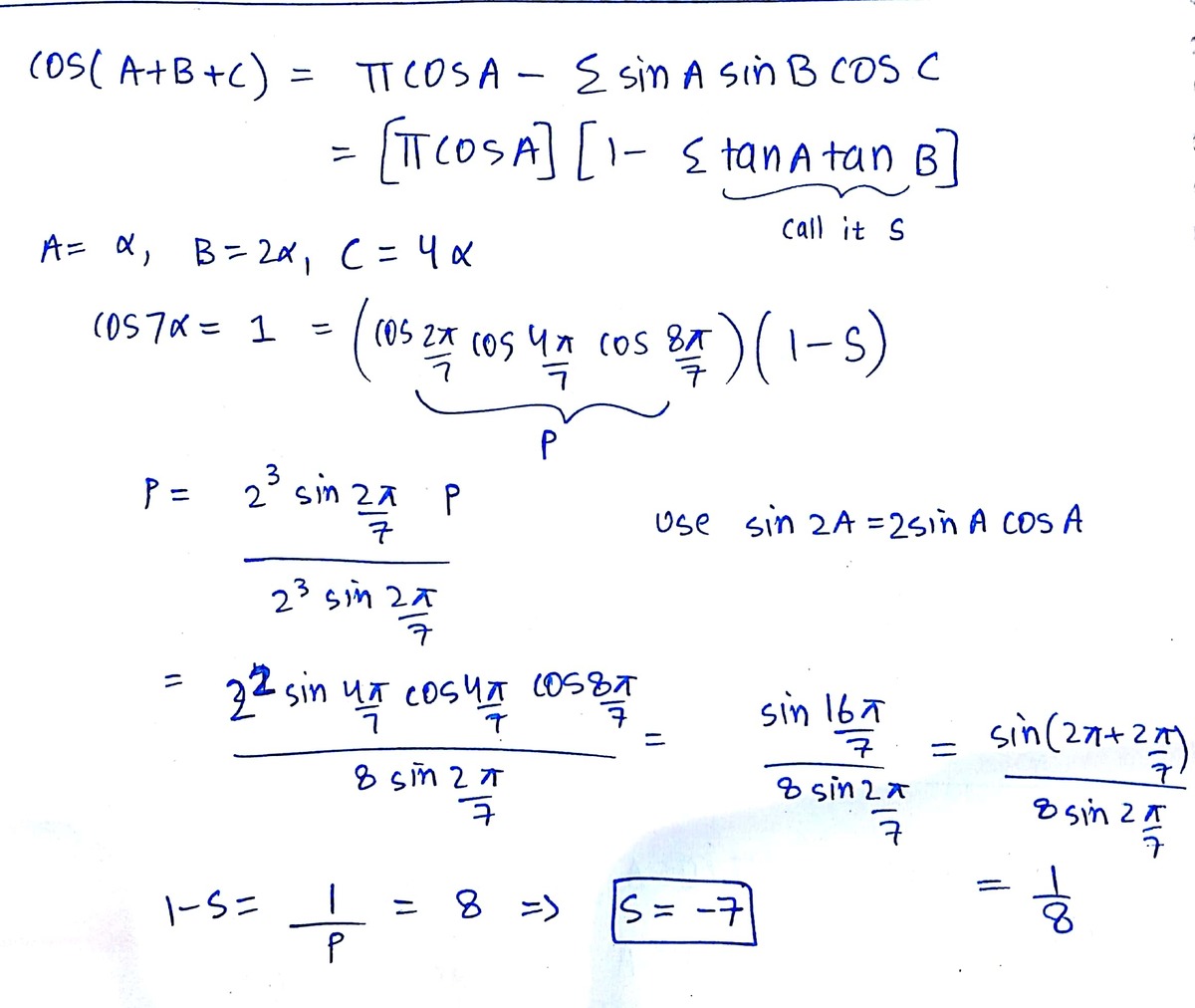
Let's consider $(cis\,x)^7= cis\,7x$

Expand the left side, we have $cis\,7x=(cos^7x-21cos^5x\,sin^2x+35cos^3x\,sin^4x-7cos\,x\,sin^6x)+i(7cos^6x\,sinx-35cos^4x\,sin^3x+21cos^2x\,sin^5x-sin^7x)$

Compare the real and imaginary parts of both side, we have $cos\,7x=cos^7x-21cos^5x\,sin^2x+35cos^3x\,sin^4x-7cos\,x\,sin^6x$ $sin\,7x=7cos^6x\,sinx-35cos^4x\,sin^3x+21cos^2x\,sin^5x-sin^7x$

Therefore, $tan\,7x=\frac{sin\,7x}{cos\,7x}=\frac{7cos^6x\,sinx-35cos^4x\,sin^3x+21cos^2x\,sin^5x-sin^7x}{cos^7x-21cos^5x\,sin^2x+35cos^3x\,sin^4x-7cos\,x\,sin^6x}$

Divide both top and bottom by $cos^7x$ : $tan\,7x=\frac{7tan\,x-35tan^3x+21tan^5x-tan^7x}{1-21tan^2x+35tan^4x-7tan^6x}$

Set $tan\,7x=0$ , we have $x=\frac{k\pi}{7},\,k=0, 1, 2, ..., 6$

Therefore the equation $7tan\,x-35tan^3x+21tan^5x-tan^7x=0$ has those 6 roots also, plus the one $x=0$

Assume $x\ne0$ , divide the equation both side by $-tan\,x$ , we have $tan^6x-21tan^4x+35tan^2x-7=0$

Thus, the equation $x^6-21x^4+35x^2-7=0$ has roots $tan\,\frac{\pi}{7}, tan\,\frac{2\pi}{7}, ..., tan\,\frac{6\pi}{7}$

Notice that $x^6-21x^4+35x^2-7=(x^3+\sqrt{7}x^2-7x+\sqrt{7})(x^3-\sqrt{7}x^2-7x-\sqrt{7})$

So 3 of the roots go to the first factor and the last 3 remaining roots go for the second factor.

Looking at the sign of the 2 factors, it is quite certain that $tan\,\frac{\pi}{7}$ and $tan\,\frac{2\pi}{7}$ are for the first factor (since that are not so large), and $tan\,\frac{5\pi}{7}$ and $tan\,\frac{6\pi}{7}$ are for the second factor (since they are negative)

Applying the famous formula $tan\,\frac{\pi}{7}tan\,\frac{2\pi}{7}tan\,\frac{3\pi}{7}=\sqrt{7}$ , $tan\,\frac{3\pi}{7}$ is not for the first factor (otherwise $tan\,\frac{\pi}{7}tan\,\frac{2\pi}{7}tan\,\frac{3\pi}{7}=-\sqrt{7}$ ), so it is for the second factor, and $tan\,\frac{4\pi}{7}$ is for the first factor

(Thus, this observation is correct)

The first factor $x^3+\sqrt{7}x^2-7x+\sqrt{7}$ has root $tan\,\frac{\pi}{7}, tan\,\frac{2\pi}{7}, tan\,\frac{4\pi}{7}$ . Therefore $tan\,\frac{\pi}{7}tan\,\frac{2\pi}{7}+tan\,\frac{2\pi}{7}tan\,\frac{4\pi}{7}+tan\,\frac{4\pi}{7}tan\,\frac{\pi}{7}=-7$

Applying $tan\,(\pi +y)=tan\,y$ , we can see $tan\,\frac{\pi}{7}=tan\,\frac{8\pi}{7}$ . So finally $tan\,\frac{2\pi}{7}tan\,\frac{4\pi}{7}+tan\,\frac{4\pi}{7}tan\,\frac{8\pi}{7}+tan\,\frac{8\pi}{7}tan\,\frac{2\pi}{7}=\boxed{-7}$

Finally Note To lead to the final answer, this is the most convenient factorization, others can have problem with the sign or even lead to dead end.