A logic problem by A Former Brilliant Member

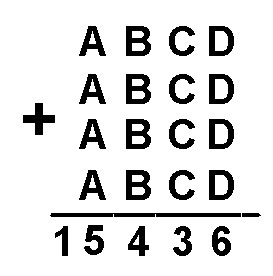(1/4)x(15436) = 3859. 3 + 8 + 5 + 9 = 25. Ed Gray

Since there are $4$ of $\overline{ABCD}$ 's, we can express the summation as the following product $\begin{array}{cccccc} & A & B & C & D\\ \times& & & & 4\\ \hline\hline 1 & 5 & 4 & 3 & 6 \end{array}$ In this case, $15436 \div 4 = 3859$ . Therefore, since $A = 3$ , $B = 8$ , $C = 5$ and $D = 9$ , $A + B + C + D = \boxed{25}$ .

$ABCD=3859$

$3859+3859+3859+3859=15436$

$A+B+C+D=3+8+5+9=25$