An AMC 10 Geometry Problem

Along the line, all points with distance 3 from the line form a cylinder with radius $3$ and height $AB$ . At each endpoint, the points of distance three from the endpoint form a sphere of radius $3$ (half of which is inside the cylinder):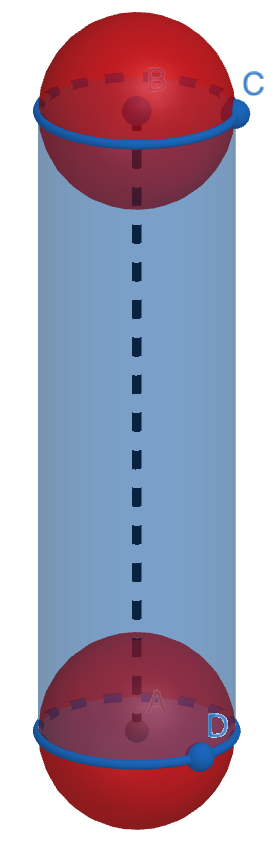 Thus, the volume enclosed is the volume of the cylinder plus the volume of the two hemispheres (which is just a sphere): $\begin{aligned} \pi r^2 \times h + \frac{4}{3}\pi r^3 &= \text{Volume enclosed} \\ 9\pi \times AC + \frac{4}{3}\times 27\pi &= 216\pi \\ 9\pi(AC + 4) &= 216\pi \\ AC + 4 &= 24 \\ AC = \boxed{20} \end{aligned}$