An electricity and magnetism problem by Sriram Radhakrishnan

Electricity and Magnetism Level 2

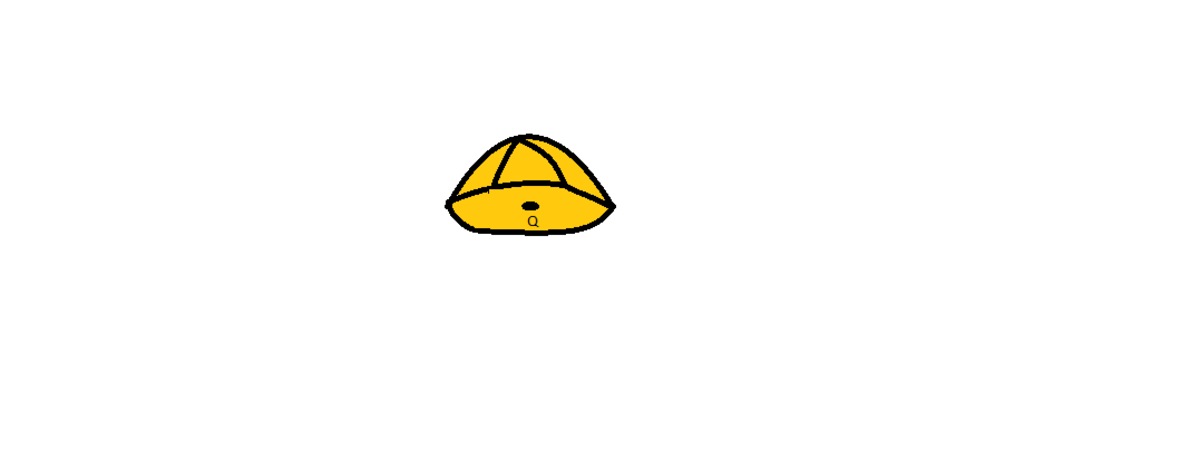
Looking at the figure above, what is the net electric flux through the curved surface of the hemisphere?

Take $Q = 44\times 10^{11} \text{ C}$ .

And let $E_0$ denote the electrical permittivity, such that $\dfrac1{E_0} = 1.1 \times 10^{11}$ .

40 \text{ Vm}

10 \text{ Vm}

20 \text{ Vm}

30 \text{ Vm}

1 solution

Ayon Ghosh
Sep 28, 2017

Very easy approach to this problem using Gauss Law,

Also $q_{enc} = Q$ . We will not directly apply the Law.

First let us construct a Gaussian Sphere (G-Sphere) with point charge at the center.

The flux through the sphere is $Q / e_0$ $=$ $40 N/C$ but since we are considering the hemisphere the flux through it is half i.e. $20 N/C$ .