A geometry problem by Aman thegreat

Geometry Level 2

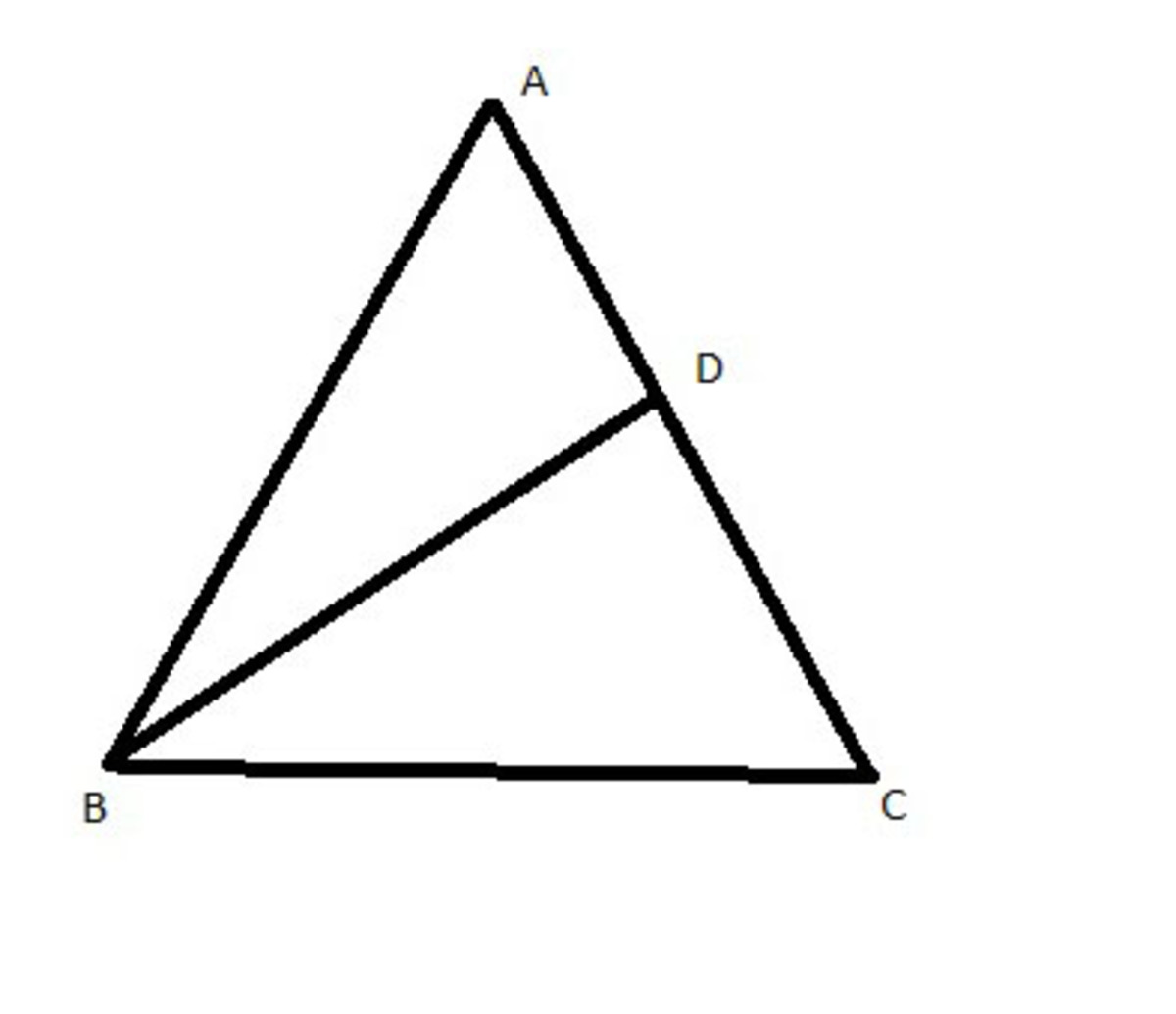
In the given figure, $D$ is a point lies on the side $AC$ of triangle $ABC$ such that $\angle BDC$ $=$ $\angle ABC$ . Then $BC^2$ - $DC^2$ always equals?

Note: Image is not to scale

AD \times DC

BC \times DC

AB \times AD

AB \times DC

1 solution

David Vreken
Dec 3, 2017

$\triangle ABC$ ~ $\triangle BDC$ because they have two pairs of congruent angles ( $\angle BDC$ = $\angle ABC$ and $\angle ACB$ = $\angle BCD$ ), and so $\frac{BC}{DC}$ = $\frac{AC}{BC}$ or $BC^2 = AC \cdot DC$ . Subtracting $DC^2$ on each side of the equation gives $BC^2 - DC^2 = AC \cdot DC - DC^2$ , and factoring $DC$ on the right side of the equation gives $BC^2 - DC^2 = (AC - DC)DC$ . Since $AC - DC = AD$ by segment subtraction, substituting this gives $BC^2 - DC^2 = AD \cdot DC$

A geometry problem by Aman thegreat

1 solution

0 pending reports