An isosceles obtuse triangle
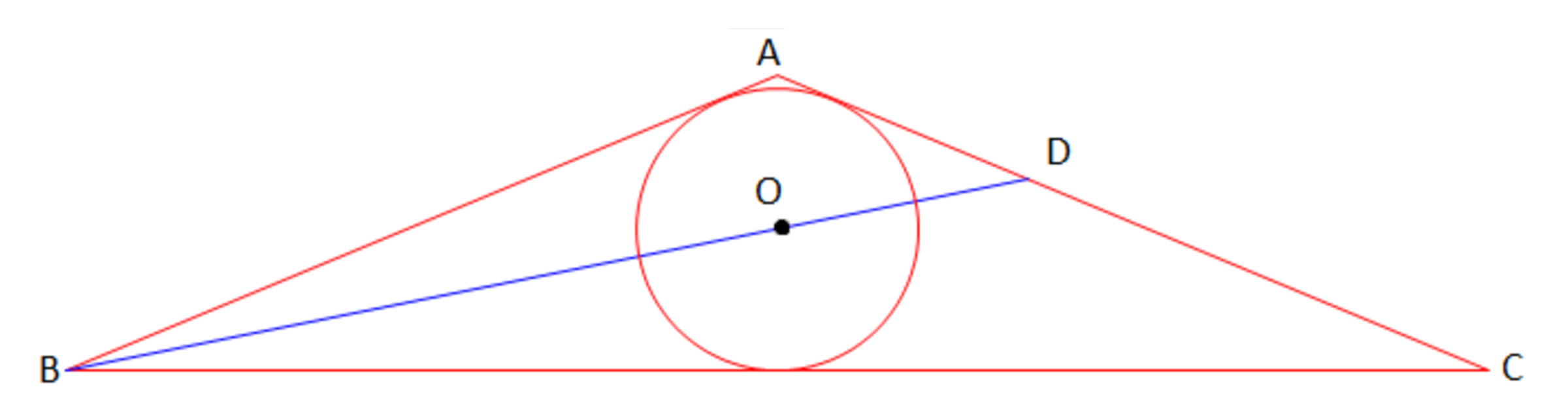
is an isosceles obtuse triangle with and . Its incircle, circle has a radius of . Line segment is extended to meet at . Find .
Figure drawn to scale
The answer is 88.366.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Since △ A B C is isosceles with ∠ A = 1 3 5 ∘ , then ∠ B = ∠ C = 2 2 . 5 ∘ . Let A E be the altitude of △ A B C which passes through center O of the incircle. Then O B bisects ∠ B and ∠ O B E = ∠ O B A = 1 1 . 2 5 ∘ .
We have B E = tan 1 1 . 2 5 ∘ 1 0 and A B = sin 2 2 . 5 ∘ B E = tan 1 1 . 2 5 ∘ sin 2 2 . 5 ∘ 1 0 ≈ 5 4 . 4 1 5 5 3 0 5 4 .
By sine rule we have sin ∠ A D B A B = sin ∠ A B D A D = sin ∠ B A D B D , then
A D B D ⟹ A D + B D = sin 3 3 . 7 5 ∘ A B ⋅ sin 1 1 . 2 5 ∘ ≈ 1 9 . 1 0 8 1 9 3 2 5 = sin 3 3 . 7 5 ∘ A B ⋅ sin 1 3 5 ∘ ≈ 6 9 . 2 5 7 8 3 3 4 2 ≈ 8 8 . 4