An Old Coin In China
This is an old coin in ancient China. There is a square hole in the middle and the length of it is 1 unit ( B C = 1 ). And A B is 2 unit long. Q: what is the area of the coin? (π is 3)
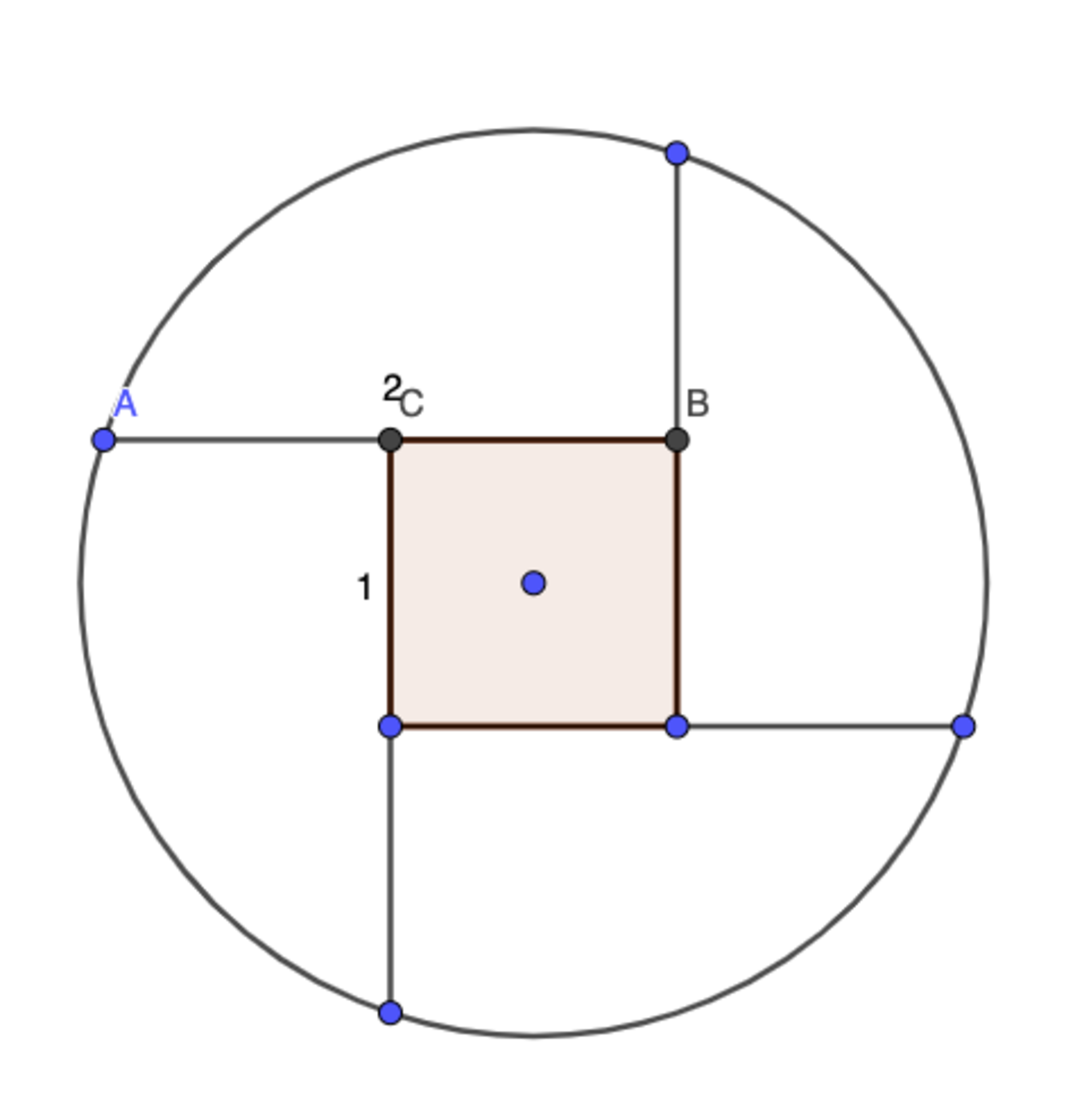
The answer is 6.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
This explanation does not take π = 3 because it is not!
Let the radius of the circle be r . Then
r 2 = ( 2 1 ) 2 + ( 1 + 2 1 ) 2 = 2 5 ⟹ π r 2 ≈ 2 1 5 = 7 . 5
⟹ area of the coin ≈ 7 . 5 − 1 2 = 6 . 5 .

We draw a line A E (the circle's diameter) , and know that A D 2 + D E 2 = A E 2 , A D = 3 , D E = 1 . So the radius should be 2 1 0 . The area is( 2 1 0 ) 2 * π - 1 2 = 6.5
Let the midpoint of C B = D
r 2 π r 2 = ( A D ) 2 + ( D O ) 2 = ( 1 . 5 ) 2 + ( 0 . 5 ) 2 = 2 . 5 = π ( 2 . 5 ) ≈ 7 . 8 5