Angles in isosceles triangle

Let $K$ be the center of the circumcircle $c$ of $\triangle ADC$ . Since $\angle BAD=\alpha=\angle DCA$ , $AB$ is tangent to circle $c$ , thus $AK\bot AB \ \ \ \ \ (1)$

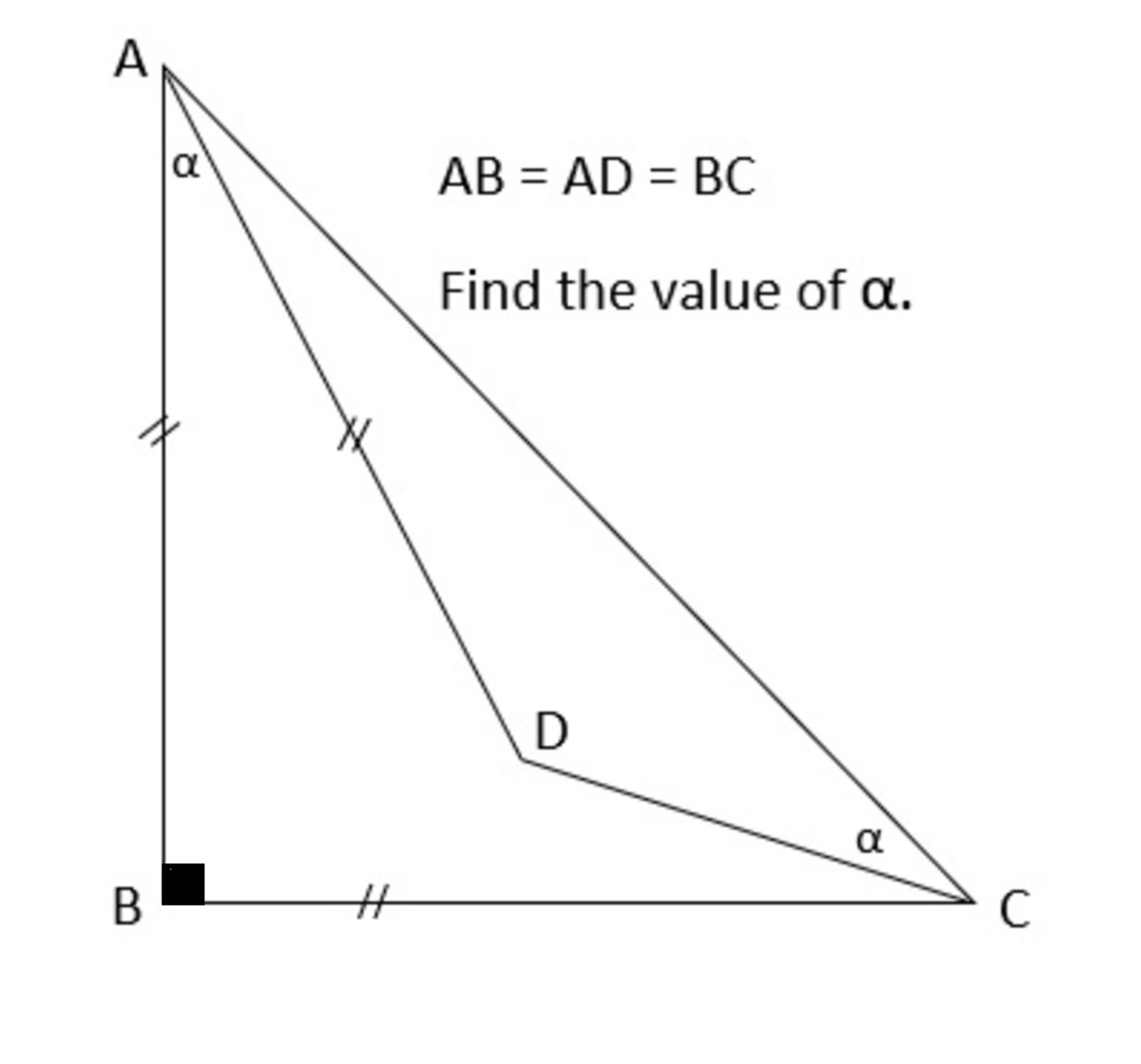
$\triangle ABC$ is isosceles right triangle, hence $\angle BAC=\angle BCA=45{}^\circ$ .

Consequently, $\angle DAC=\angle BAC-\angle BAD=45{}^\circ -\angle \alpha =\angle BCA-\angle DCA=\angle BCD$ . This means that $BC$ is tangent to circle $c$ , thus, $CK\bot BC \ \ \ \ \ (2)$

$\left( 1 \right), \ \left( 2 \right)$ , combined with the fact that $\angle ABC=90{}^\circ$ and $AB=BC$ give us that $ABCK$ is a square.

Since $AB=AD=AK$ , $D$ lies on the intersection of circle $c$ and the quadrant $KB$ of center $A$ . This implies that $\triangle ADK$ is equilateral, hence, $\angle KAD=60{}^\circ$ .

Finally, $\alpha=\angle BAD=\angle KAB-\angle KAD=90{}^\circ-60{}^\circ=\boxed{30{}^\circ}$ .

Let $x = AB = BC = AD$ . Since $\triangle ABC$ is a right isosceles triangle, $\angle BAC = 45°$ and $AC = \sqrt{2}x$ .

$\angle DAC = \angle BAC - \angle BAD = 45° - \alpha$ .

By the sum of the angles in $\triangle DAC$ , $\angle ADC = 180° - \angle DAC - \angle DCA = 180° - (45° - \alpha) - \alpha = 135°$ .

By the law of sines on $\triangle ADC$ , $\cfrac{\sin \angle DCA}{AD} = \cfrac{\sin ADC}{AC}$ , or $\cfrac{\sin \alpha}{x} = \cfrac{\sin 135°}{\sqrt{2}x}$ , which solves to $\sin \alpha = \cfrac{1}{2}$ , so $\alpha = \boxed{30°}$ .