Annoying conics are annoying
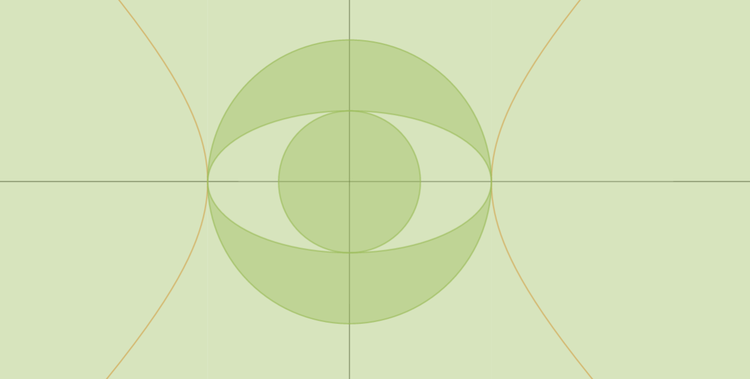 The smallest possible circle tangent to both sides of a hyperbola
x
2
−
y
2
=
1
circumscribes an ellipse with minor axis half the length of the major axis. The largest possible circle is inscribed in this ellipse. The area of the region within both circles but not containing the area within the ellipse can be expressed as
Z pi
. Find Z in decimal.
The smallest possible circle tangent to both sides of a hyperbola
x
2
−
y
2
=
1
circumscribes an ellipse with minor axis half the length of the major axis. The largest possible circle is inscribed in this ellipse. The area of the region within both circles but not containing the area within the ellipse can be expressed as
Z pi
. Find Z in decimal.
The answer is 0.75.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Can I request you for a detailed explanation
Here you go @Anany Prakhar .
Let's first start by finding the coordinates of the vertices of the two parabolas that make up the whole hyperbola. Seeing how this has an axis of symmetry at y = 0 , we plug in to the equation given, which produces ( ± 1 , 0 ) . Thus, the diameter of the circle inscribed is 2 . From here, we can deduce that the area of this circle is simply π .
Now, let's examine the ellipse. Its major axis is the diameter of the larger circle that we just looked at. From the information we've been given, the minor axis is half of the major axis, which is thus 1 . Now, we can plug into our formula for area A B π where A and B are the maximum and minimum distance from the perimeter to the center of the ellipse. Thus, we have 0 . 5 π as the area of the ellipse.
To solve for the area of the smallest circle, we see that its diameter is the minor axis of the ellipse described above. Thus, its area is 0 . 2 5 π .
With our areas in hand, we can first find the area of non-ellipse section of the largest circle, which is π − 0 . 5 π ⟹ 0 . 5 π . Then, we'll add the area of the smallest circle, 0 . 2 5 π to get 0 . 7 5 π . From here, our desired answer is simply 0 . 7 5 .
The area of the ellipse is phi/2, so the unshaded area is phi/4. The shaded area is phi - phi/4 = 0.75 phi