Another Challenging Circle Problem
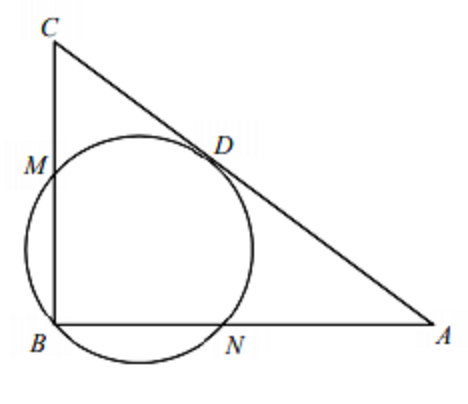
In the figure, A C is a tangent to the circle at D . B is a point on the circle. B C cuts the circle at M and B A cuts the circle at N . It is given that A B = 8 , B C = 6 and A C = 1 0 .
Find the minimum value of M N .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
thank you!
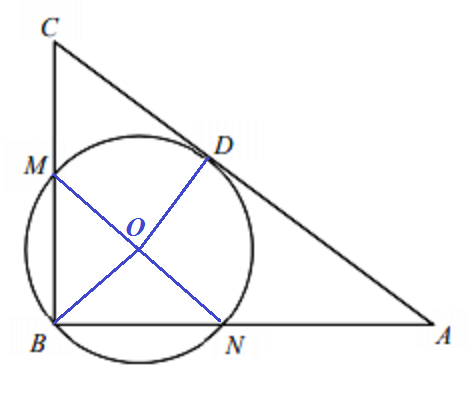
Note that △ A B C is a right triangle because 6 2 + 8 2 = 1 0 2 , where ∠ B = 9 0 ∘ . Since B , M , and N are points on the circle, and ∠ B = 9 0 ∘ , M N must be the diameter of the circle. The circle with the smallest diameter and hence the shortest M N is when B D is a straight line. Then B D = M N , the diameter of the circle. And that △ B C D is similar to △ A B C , therefore B C B D = A C A B = 5 4 ⟹ B D = M N = 5 4 B C = 5 4 × 6 = 4 . 8 .
BD is minimum when it is perpendicular to AC.
B D m i n = 4 . 8
Also, as AC is tangent, and BD is perpendicular to it ⇒ BD is diameter of circle.
According to given sides of triangle, angle B = 90°
So, MN is also diameter of circle (diameter subtends 90° angle at circumference)
So, M N m i n = B D m i n = 4 . 8