Another covering problem - 2

Geometry Level 2

Consider the figure below. The central circle has diameter 1, and each of the eight evenly spaced rays is length 1 (despite my somewhat dubious drawing skills.)

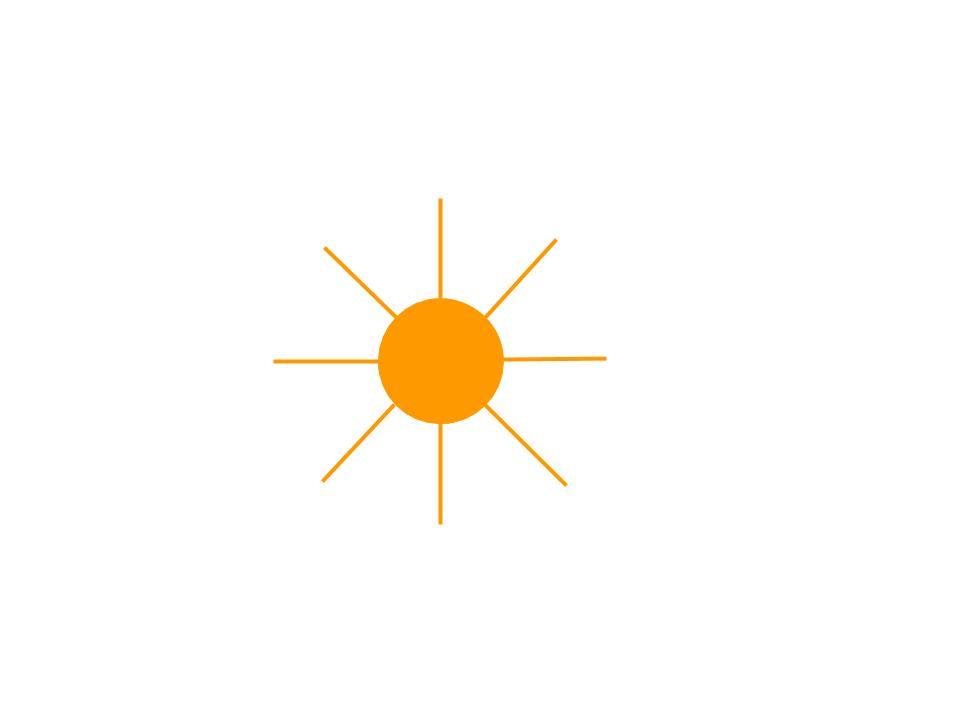
Now consider another copy of the figure which is scaled up in size by 0.1%. How many copies of the original figure are needed to cover it?

Inspiration

Confusion


The answer is 8.

Varsha Dani by Varsha Dani , 47, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Jacob Byker
Oct 22, 2018

If you were to connect the rays it would create an octagon. If you were to increase the size of an octagon you would need at least 8 octagons to fill the space.

2 Helpful 0 Interesting 0 Brilliant 0 Confused

8 is correct for this figure, but your reasoning is not. You don't need eight octagons to cove a slightly increased octagon. See the problem that inspired this.

Varsha Dani - 2 years, 7 months ago
David Vreken
Nov 5, 2018

Using trigonometry, we find that the tip of each adjacent ray is separated by more than 1 1 unit, so there is no hope of covering two tips at once with a unit circle. Therefore, at least 8 8 copies of the original figure are needed to cover the 8 8 ray tips.

If the 8 8 copies are placed so that each center is slightly off-center of the new copy (but just enough to cover a ray tip), the central circles will overlap to cover the new copy's central circle as well.

Therefore, 8 \boxed{8} copies of the original figure are sufficient to cover the new bigger copy.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...