Another Cyclic Trapezoid
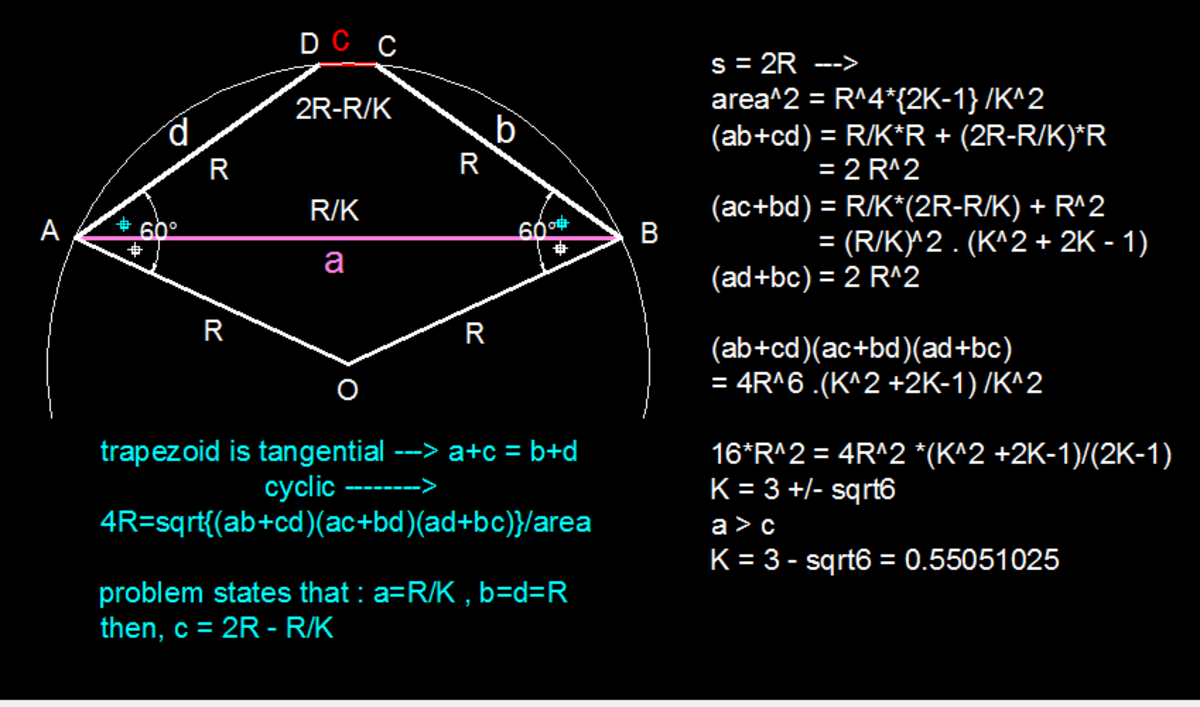
A trapezoid A B C D is circumscribed around a circle with radius r and inscribed in a circle with radius R , with bases A B and C D , such that A B > C D and B C = A D = R . If R = k ⋅ A B , find k to the nearest 3 decimal places.
The answer is 0.55051025.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Call the center of the larger circle Z and call the smaller circle X . Furthermore, let E , F , G , H be the points on A B , B C , C D , A D , respectively, where circle X is tangent. We know that G C = F C and E B = F B . Furthermore, by symmetry, we see that E is the midpoint of A B and G is the midpoint of C D . If we say A B = b and C D = a , then we have R = B C = B F + C F = 2 a + 2 b , so a + b = 2 R , and the semi-perimeter can be expressed as S = 2 R + R + a + b = 2 R + R + ( 2 R ) = 2 R .
Next, we calculate the area of A B C D : A = ( S − a ) ( S − R ) ( S − b ) ( S − R ) = R ( 2 R − a ) ( 2 R − b ) = R a b . Using the area of a trapezoid formula,
R a b = A = h 2 b 1 + b 2
R a b = ( E Z + G Z ) 2 a + b
R a b = ( B Z 2 − B E 2 + C Z 2 − C G 2 ) ⋅ R
a b = R 2 − ( 2 a ) 2 + R 2 − ( 2 b ) 2
a b = ( R 2 − ( 2 a ) 2 ) + 2 ( R 2 − ( 2 a ) 2 ) ( R 2 − ( 2 b ) 2 ) + ( R 2 − ( 2 b ) 2 )
4 ( a + b ) 2 + 2 a b − 2 R 2 = 2 ( R 2 − ( 2 a ) 2 ) ( R 2 − ( 2 b ) 2 )
a b − 2 R 2 = ( 4 R 2 − a 2 ) ( 4 R 2 − b 2 )
a 2 b 2 − 4 R 2 a b + 4 R 4 = 1 6 R 4 − 4 ( a 2 + b 2 ) R 2 + a 2 b 2
4 ( a 2 + 2 a b + b 2 − 3 a b ) R 2 = 1 2 R 4
4 R 2 − 3 a b = 3 R 2
R 2 = 3 a b = 3 b ( 2 R − b )
R 2 − 6 b R + 3 b 2 = 0 .
And using the quadratic formula, we get R = ( 3 ± 6 ) b . Using these values to solve for a , we get R = ( 3 ∓ 6 ) a . Since we know that b > a , we take the larger value of b as the only valid solution. Thus R = ( 3 − 6 ) b is the valid solution.