Archimedean Siblings

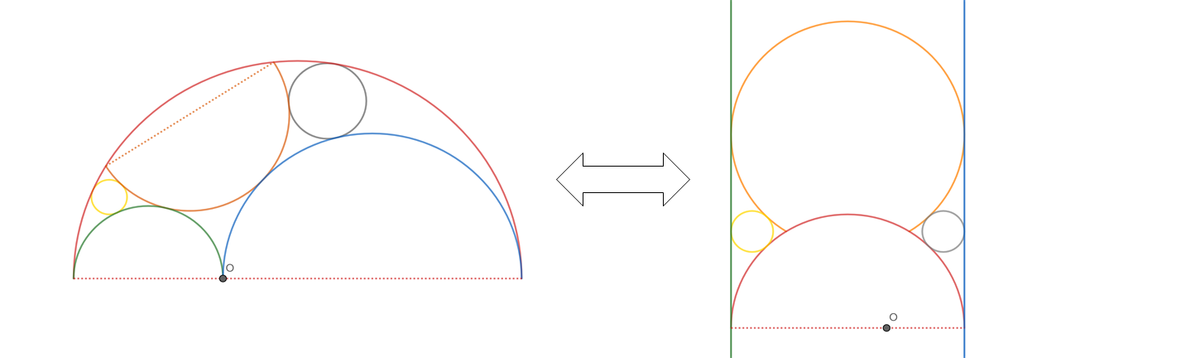
Label the diagram as follows:

Let $OD = 2$ and $OC = 4$ , so that the radius of the left semi-circle is $1$ , the radius of the right semi-circle is $2$ , and the radius of the large semi-circle is $3$ , and $DA = AO = OB = BE = 1$ and $EC = 2$ .

---

Let the radius of the upside-down semi-circle be $R$ . Then by the Pythagorean Theorem on $\triangle KHB$ , $KB = \sqrt{HB^2 - KH^2} = \sqrt{9 - R^2}$ .

By the law of cosines on $\triangle AKB$ , $\cos \angle KAB = \cfrac{AK^2 + AB^2 - KB^2}{2 \cdot AK \cdot AB} = \cfrac{(R + 1)^2 + 2^2 - (9 - R^2)}{2 \cdot (R + 1) \cdot 2} = \cfrac{R^2 + R - 2}{2(R + 1)}$ .

By the law of cosines on $\triangle AKE$ , $\cos \angle KAB = \cfrac{AK^2 + AE^2 - KE^2}{2 \cdot AK \cdot AE} = \cfrac{(R + 1)^2 + 3^2 - (R + 2)^2}{2 \cdot (R + 1) \cdot 3} = \cfrac{3 - R}{3(R + 1)}$ .

Therefore, $\cos \angle KAB = \cfrac{R^2 + R - 2}{2(R + 1)} = \cfrac{3 - R}{3(R + 1)}$ , which solves to $R = \cfrac{4}{3}$ for $R > 0$ .

---

Since $R = \cfrac{4}{3}$ , $AK = 1 + \cfrac{4}{3} = \cfrac{7}{3}$ , $KE = 2 + \cfrac{4}{3} = \cfrac{10}{3}$ , and $\cos \angle KAB = \cfrac{3 - \frac{4}{3}}{3(\frac{4}{3} + 1)} = \cfrac{5}{21}$ .

That means $\sin \angle KAB = \sqrt{1 - \cos^2 \angle KAB} = \sqrt{1 - \bigg(\cfrac{5}{21}\bigg)^2} = \cfrac{4\sqrt{26}}{21}$ .

By the law of cosines on $\triangle AKE$ , $\cos \angle KEA = \cfrac{KE^2 + AE^2 - AK^2}{2 \cdot KE \cdot AE} = \cfrac{(\frac{10}{3})^2 + 3^2 - (\frac{7}{3})^2}{2 \cdot \frac{10}{3} \cdot 3} = \cfrac{11}{15}$ .

That means $\sin \angle KEA = \sqrt{1 - \cos^2 \angle KEA} = \sqrt{1 - \bigg(\cfrac{11}{15}\bigg)^2} = \cfrac{2\sqrt{26}}{15}$ .

---

Let the smaller circle have a radius of $S$ . By the law of cosines on $\triangle AFK$ , $\cos \angle FAK = \cfrac{AF^2 + AK^2 - FK^2}{2 \cdot AF \cdot AK} = \cfrac{(S + 1)^2 + (\frac{7}{3})^2 - (S + \frac{4}{3})^2}{2 \cdot (S + 1) \cdot \frac{7}{3}} = \cfrac{7 - S}{7(S + 1)}$ .

That means $\sin \angle FAK = \sqrt{1 - \cos^2 \angle FAK} = \sqrt{1 - \cfrac{(7 - S)^2}{7^2(S + 1)^2}} = \cfrac{4\sqrt{S(3S + 7)}}{7(S + 1)}$ .

So $\cos \angle FAB = \cos (\angle FAK + \angle KAB) = \cos \angle FAK \cdot \cos \angle KAB - \sin \angle FAK \cdot \sin \angle KAB = \cfrac{7 - S}{7(S + 1)} \cdot \cfrac{5}{21} - \cfrac{4\sqrt{S(3S + 7)}}{7(S + 1)} \cdot \cfrac{4\sqrt{26}}{21} = \cfrac{5(7 - S) - 16\sqrt{26S(3S + 7)}}{147(S + 1)}$ .

By the law of cosines on $\triangle FAB$ , $\cos FAB = \cfrac{AF^2 + AB^2 - FB^2}{2 \cdot AF \cdot AB} = \cfrac{(S + 1)^2 + 2^2 - (3 - S)^2}{2 \cdot (S + 1) \cdot 2} = \cfrac{2S - 1}{S + 1}$ .

Therefore, $\cos \angle FAB = \cfrac{5(7 - S) - 16\sqrt{26S(3S + 7)}}{147(S + 1)} = \cfrac{2S - 1}{S + 1}$ , which solves to $S = \cfrac{26}{109}$ .

---

Let the larger circle have a radius of $T$ . By the law of cosines on $\triangle KEL$ , $\cos \angle KEL = \cfrac{EL^2 + KE^2 - KL^2}{2 \cdot EL \cdot KE} = \cfrac{(T + 2)^2 + (\frac{10}{3})^2 - (T + \frac{4}{3})^2}{2 \cdot (T + 2) \cdot \frac{10}{3}} = \cfrac{T + 10}{5(T + 2)}$ .

That means $\sin \angle KEL = \sqrt{1 - \cos^2 \angle KEL} = \sqrt{1 - \cfrac{(T + 10)^2}{5^2(T + 2)^2}} = \cfrac{2\sqrt{2T(3T + 10)}}{5(T + 2)}$ .

So $\cos \angle BEL = \cos (\angle KEA + \angle KEL) = \cos \angle KEA \cdot \cos \angle KEL - \sin \angle KEA \cdot \sin \angle KEL = \cfrac{11}{15} \cdot \cfrac{T + 10}{5(T + 2)} - \cfrac{2\sqrt{26}}{15} \cdot \cfrac{2\sqrt{2T(3T + 10)}}{5(T + 2)} = \cfrac{11(T + 10) - 8\sqrt{13T(3T + 10)}}{75(T + 2)}$ .

By the law of cosines on $\triangle BEL$ , $\cos BEL = \cfrac{EL^2 + BE^2 - BL^2}{2 \cdot EL \cdot BE} = \cfrac{(T + 2)^2 + 1^2 - (3 - T)^2}{2 \cdot (T + 2) \cdot 1} = \cfrac{5T - 2}{T + 2}$ .

Therefore, $\cos \angle BEL = \cfrac{11(T + 10) - 8\sqrt{13T(3T + 10)}}{75(T + 2)} = \cfrac{5T - 2}{T + 2}$ , which solves to $T = \cfrac{13}{25}$ .

---

Therefore, the ratio of the radius of the larger circle to the smaller circle is $\cfrac{T}{S} = \cfrac{\frac{13}{25}}{\frac{26}{109}} = \cfrac{109}{50}$ , so $p = 109$ , $q = 50$ , and $p + q = \boxed{159}$ .