Are they crazy?

Assuming that the distance between Addalia and Cyprus is equal to the length of arc of a circle (which is a cross-section of sphere ) we can calculate the angle : $\left ( \frac{\theta }{360^\circ} \right )\cdot 2\pi\cdot 6400km = 123.2 km\Rightarrow \theta = 1.102943756^\circ$ $\frac{R}{R+h}= cos\theta$ $\therefore h= 1.853098334\cdot R = 1185.982934 m$

I took 123.5km to be the straight line distance from Addalia to the Cyprus coast (shown yellow in your figure) and then used Pythagoras: the height we seek is $\sqrt{6400^2+123.2^2}-6400\approx{1185.7m}$ Interesting problem!

For an exact answer with a bit of trigonometry, assuming the distance $d=123'200\,$ m is along a straight line (going under water) from Addalia (0 meter above sea level) to Cyprus:

$\cos(\theta)=\frac{R}{R+h} \Rightarrow h=R·(\frac{1}{\cos(\theta)}-1)$ .

$\sin(\frac{\theta}{2})=\frac{\frac{d}{2}}{R}=\frac{d}{2R}$ .

From this, we have $\cos(\theta)=\cos(2·\frac{\theta}{2})=\cos^2(\frac{\theta}{2})-\sin^2(\frac{\theta}{2})=1-2\sin^2(\frac{\theta}{2})=1-2(\frac{d}{2R})^2$ Then, $\frac{1}{\cos(\theta)}=\frac{1}{1-2(\frac{d}{2R})^2}=\frac{2R^2}{2R^2-d^2}$ .

Finally, $h=R·(\frac{1}{\cos(\theta)}-1)=R·(\frac{2R^2}{2R^2-d^2}-1)=\frac{R·d^2}{2R^2-d^2}=1'186.02\,$ m.

Just a side note (is it possible to comment the problems itself on this site?): The question should have been "...required to see the Cyprus coastline from Addalia." Now, as the question was to determine the height needed to be able to see Cyprus, I'm a bit confused if I should look up the height and position of Mount Olympus, let alone - to be hatefully punctilious - the whole survey data of Cyprus. ;) And we haven't even thought about the refraction of light in the atmosphere!

h=(d^2)/2R simply by putting values we get h ...its a simple survey problem :)

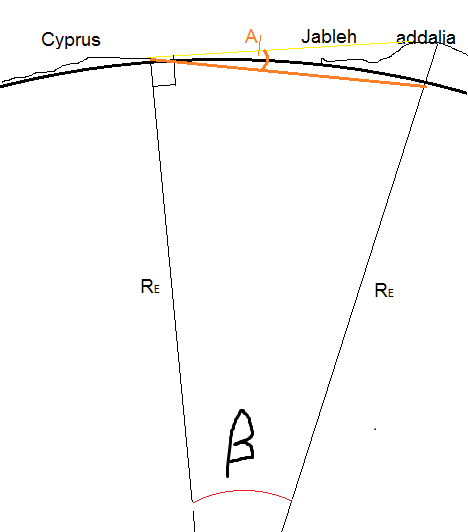
The central angle $β=\frac{D}{ R_E }$ , it's known that the circumferential angle in a circle is half of the central angle which limits the same arc, so $A=\frac{1}{2} β→A=\frac{D}{ 2 R_E }$ , we want the height the observer should be at so that he can see Cyprus, it is $D \tan A$ , since $A$ is a small angle we may consider its tangent the same as it,so $h=AD=\frac{D^2}{2R_E }=\frac{123.2^2}{2*6400}=1185.8$