Are you Smart in Simple Algebra?
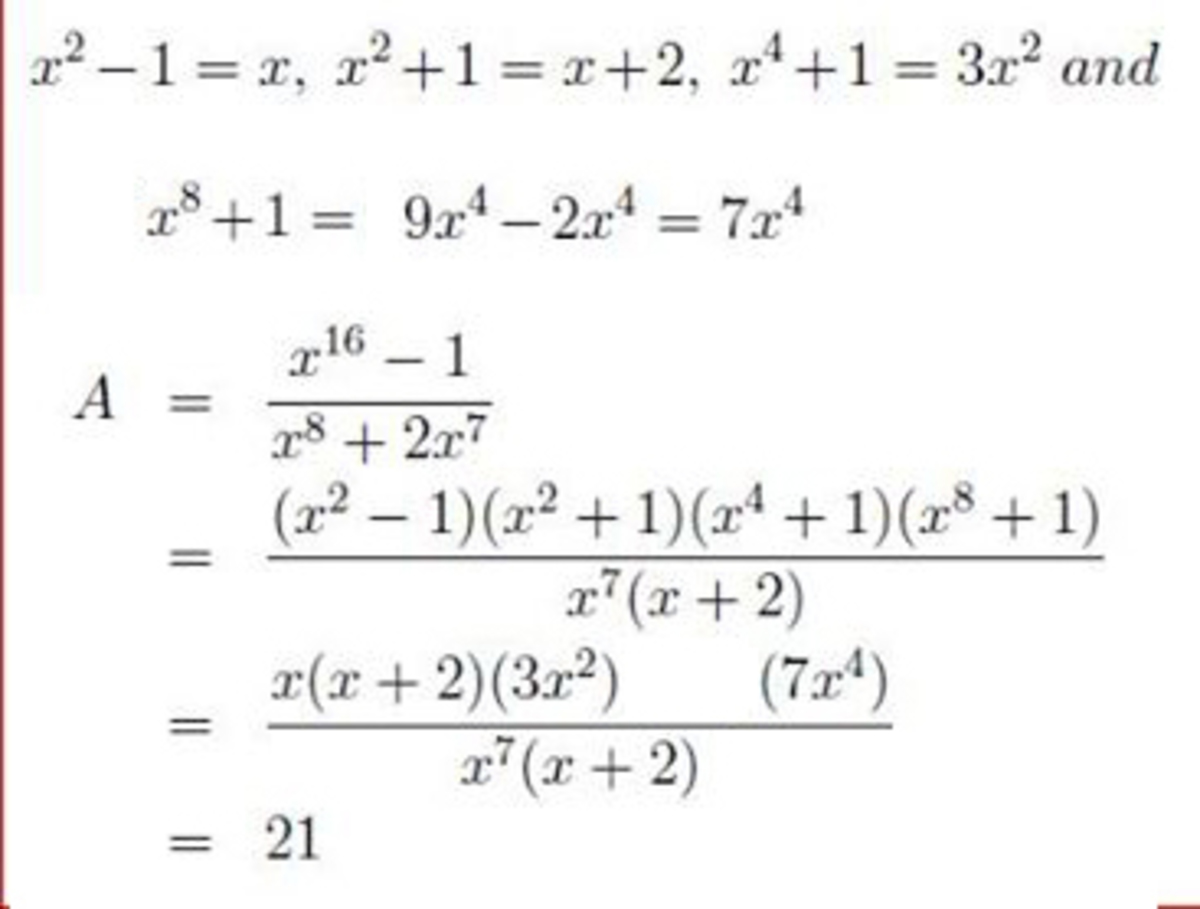
If x 2 − x − 1 = 0 , then find the value of the expression below
x 8 + 2 x 7 x 1 6 − 1 = ?
The answer is 21.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
Moderator note:
This is a solution that I consider "solve by magical observation". It doesn't offer us any good insight into why the statement is true, or how to deal with a similar problem like this in future.
Outstanding :)
Astonishing!!i can also be clever!same approach
Log in to reply
Hehe! Cheers!
This was one good solution!!
How did u get x^4+1=3x^2 and x^8+1=7x^4
Log in to reply
x'2-1=x...>square it on both sides to get x'4+1=3 x'2
Nice and simple way..
I did the same!! Look at my solution!
Suppose α is a root of x 2 − x − 1 = 0 Then α 2 = α + 1 α 4 = ( α + 1 ) 2 = α 2 + 2 α + 1 = ( α + 1 ) + 2 α + 1 = 3 α + 2 α 8 = ( α 4 ) 2 = ( 3 α + 2 ) 2 = 9 α 2 + 1 2 α + 4 = 9 ( α + 1 ) + 1 2 α + 4 = 2 1 α + 1 3 α 1 6 = ( 2 1 α + 1 3 ) 2 = 4 4 1 α 2 + 1 6 9 + 5 4 6 α = 4 4 1 ( α + 1 ) + 5 4 6 α + 1 6 9 = 9 8 7 α + 6 1 0 α 7 = α ⋅ α 2 ⋅ α 4 = α ( α + 1 ) ( 3 α + 2 ) = 1 3 α + 8
Putting in given expression we get the desired expression equals
α 7 ( α + 2 ) 9 8 7 α + 6 0 9 = ( 1 3 α + 8 ) ( α + 2 ) 9 8 7 α + 6 0 9 = ( 1 3 α 2 + 3 4 α + 1 6 ) 9 8 7 α + 6 0 9 = 4 7 α + 2 9 9 8 7 α + 6 0 9 = 4 7 α + 2 9 2 1 ( 4 7 α + 2 9 ) = 2 1
NOTE: A shortcut method We observe that α k = F k ⋅ α + F k − 1 where k is natural number and F k is k th Fibonacci number
So the higher powers of α can be obtained easily by this procedure than the above procedure and then put into the expression.
Moderator note:
The reason for the relationship to Fibonacci numbers is that the characteristic equation of F n is x 2 − x − 1 = 0 . This also offers us a way to deal with the generalized problem.
Well, without using Mathematical Induction, can you prove that α k = F k α + F k − 1 ?
⇒ x 8 + 2 x 7 x 1 6 − 1
⇒ x 7 ( x + 2 ) ( x 8 + 1 ) ( x 4 + 1 ) ( x 2 + 1 ) ( x + 1 ) ( x − 1 ) . . . . . ( A )
Now , we are given ,
⇒ x 2 − x − 1 = 0
We will make some observations ,
⇒ x 2 − 1 = x
⇒ ( x + 1 ) ( x − 1 ) = x ..... ( 1 )
Also ,
⇒ x 2 − 1 = x
Squaring it ,
⇒ ( x 2 − 1 ) 2 = x 2
⇒ ( x 4 + 1 ) = 3 x 2 ..... ( 2 )
Also ,
⇒ ( x 4 + 1 ) 2 = ( 3 x 2 ) 2
⇒ ( x 8 + 1 ) = 7 x 4 ..... ( 3 )
Substituting ( 1 ) , ( 2 ) and ( 3 ) in ( A ) ,
⇒ x 7 ( x + 2 ) 7 x 4 × 3 x 2 × ( x 2 + 1 ) × x
⇒ ( x + 2 ) 7 × 3 × ( x 2 + 1 ) . . . . . ( B )
Now again an observation by the given equation ,
⇒ x 2 − x − 1 = 0
⇒ x 2 − 1 = x
Adding 2 both sides ,
⇒ x 2 + 1 = x + 2 ..... ( 4 )
Placing ( 4 ) in ( B ) we will get ,
⇒ 7 × 3 × 1 = 2 1
This is not a solution.
Cheating! :P
x 2 − x − 1 x 2 x x + x 1 x 8 + 2 x 7 x 1 6 − 1 = 0 = x + 1 = 1 + x 1 . . . ( 1 ) = 1 + x 2 = 1 + x 2 x 8 − x 8 1 = x + x 1 x 8 − x 8 1 = ( x 4 + x 4 1 ) ( x 2 + x 2 1 ) ( x − x 1 )
From ( 1 )
x − x 1 x 2 + x 2 1 − 2 x 2 + x 2 1 x 4 + x 4 1 = 1 = 1 = 3 = 7
Substituting the values thus obtained,
x 8 + 2 x 7 x 1 6 − 1 = 7 . 3 . 1 = 2 1