Area

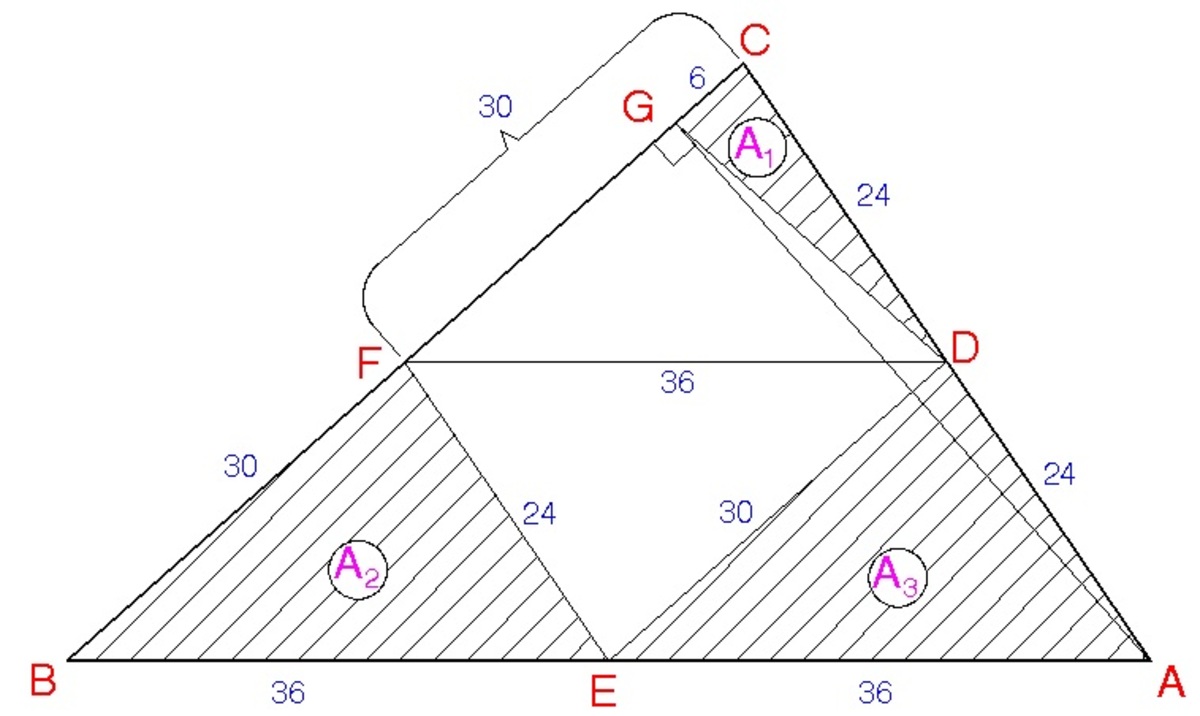
In △ A B C , E is the midpoint of A B , F is the midpoint of B C and D is the midpoint of A C . A G is an altitude of △ A B C . If A B = 7 2 , B C = 6 0 and A C = 4 8 , what is the area of E F G D . If your answer is of the form a b , where a and b are positive integers with b square-free, give your answer as a + b .
The answer is 250.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
 Draw
G
D
to form trapezoid
E
F
G
D
.
Draw
G
D
to form trapezoid
E
F
G
D
.
G D is the median to hypotenuse C A of right △ A G C . Therefore, G D = 2 1 A C = 2 1 ( 4 8 ) = 2 4 .
E F = 2 1 A C = 2 1 ( 4 8 ) = 2 4 ; E D = 2 1 B C = 2 1 ( 6 0 ) = 3 0 ; F D = 2 1 A B = 2 1 ( 7 2 ) = 3 6
Apply pythagorean theorem on △ A G C and △ A G B . A G 2 = 4 8 2 − C G 2 and A G 2 = 7 2 2 − ( 6 0 − C G ) 2 . We find G C = 6
Compute A 1 : ⟹ s = 2 2 4 + 2 4 + 6 = 2 7 ⟹ A 1 = 2 7 ( 2 7 − 2 4 ) ( 2 7 − 2 4 ) ( 2 7 − 6 ) = 5 1 0 3 = 2 7 7
Compute A 2 : ⟹ s = 2 3 0 + 2 4 + 3 6 = 4 5 ⟹ A 2 = 4 5 ( 4 5 − 3 0 ) ( 4 5 − 2 4 ) ( 4 5 − 3 6 ) = 1 2 7 5 7 5 = 1 3 5 7
Compute A 3 : ⟹ A 3 = A 2 = 1 3 5 7
Compute area of △ A B C : ⟹ s = 2 6 0 + 4 8 + 7 2 = 9 0 ⟹ A A B C = 9 0 ( 9 0 − 6 0 ) ( 9 0 − 4 8 ) ( 9 0 − 7 2 ) = 2 0 4 1 2 0 0 = 5 4 0 7
Finally,
A E F G D = A A B C − A 1 − A 2 − A 3 = 5 4 0 7 − 2 7 7 − 1 3 5 7 − 1 3 5 7 = 2 4 3 7
It follows that,
a + b = 2 4 3 + 7 = 2 5 0
A B = 6 0 = 1 2 ∗ 5 , B C = 7 2 = 1 2 ∗ 6 , A C = 4 8 = 1 2 ∗ 4 . A r e a o f A B C 4 1 ∗ 1 2 2 ∗ 1 5 ∗ 5 ∗ 7 ∗ 3 = 5 4 0 7 . ∴ A G = 2 ∗ 6 0 5 4 0 7 = 1 8 7 . S o i n A G C b y P y t h a g o r e a n T h e o r e m , C G = 6 . B u t C F = 2 1 ∗ 6 0 = 3 0 . ∴ A r e a C G D = 3 0 6 ∗ A r e a C F D = 5 1 ∗ A r e a C F D . E a s y t o s e e A r e a C F D = A r e a D F E = 4 1 ∗ A r e a A B C = 1 3 5 7 . A r e a E F G D = A r e a s C F D + D F E − C G D = ( 1 3 5 + 1 3 5 − 5 1 3 5 ) ∗ 7 = 2 4 3 7 = a b . S o A r e a E F G D = 2 5 0 .
Find c o s B using cosine rule which is c o s B = 4 3
Connect G and D then EFGD is a trapezium as G F ∣ ∣ E D .
As triangle ABG is right-angled so, using the value of cosB we can find BG=54 and AG= 1 8 7 .
As D and E are mid-points of AB and AC then D E ∣ ∣ B C and BF=DE=60/2=30
So, B G = D E + F G = 5 4
The height is also divided at midpoint because D E ∣ ∣ B C and D is the mid-point of AC.
So, height of trapezium is A G / 2 = 9 7
So, area of trapezium is 2 1 ( D E + F G ) × 9 7 = 2 1 × 5 4 × 9 7 = 2 4 3 7