Area enclosed by a string on a lattice
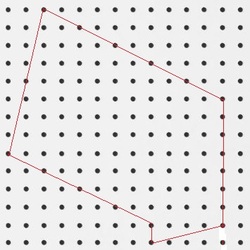 In the figure shown a lattice of dots has pegs which are connected by a red string.
In the figure shown a lattice of dots has pegs which are connected by a red string.
If the distance between the dots is 1 unit, what is the area enclosed by the string?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
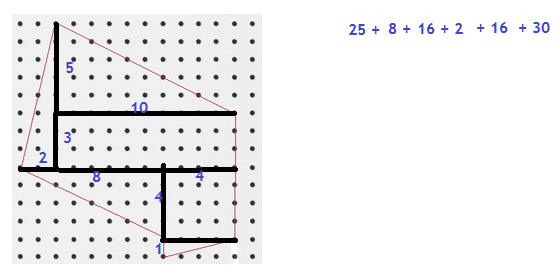
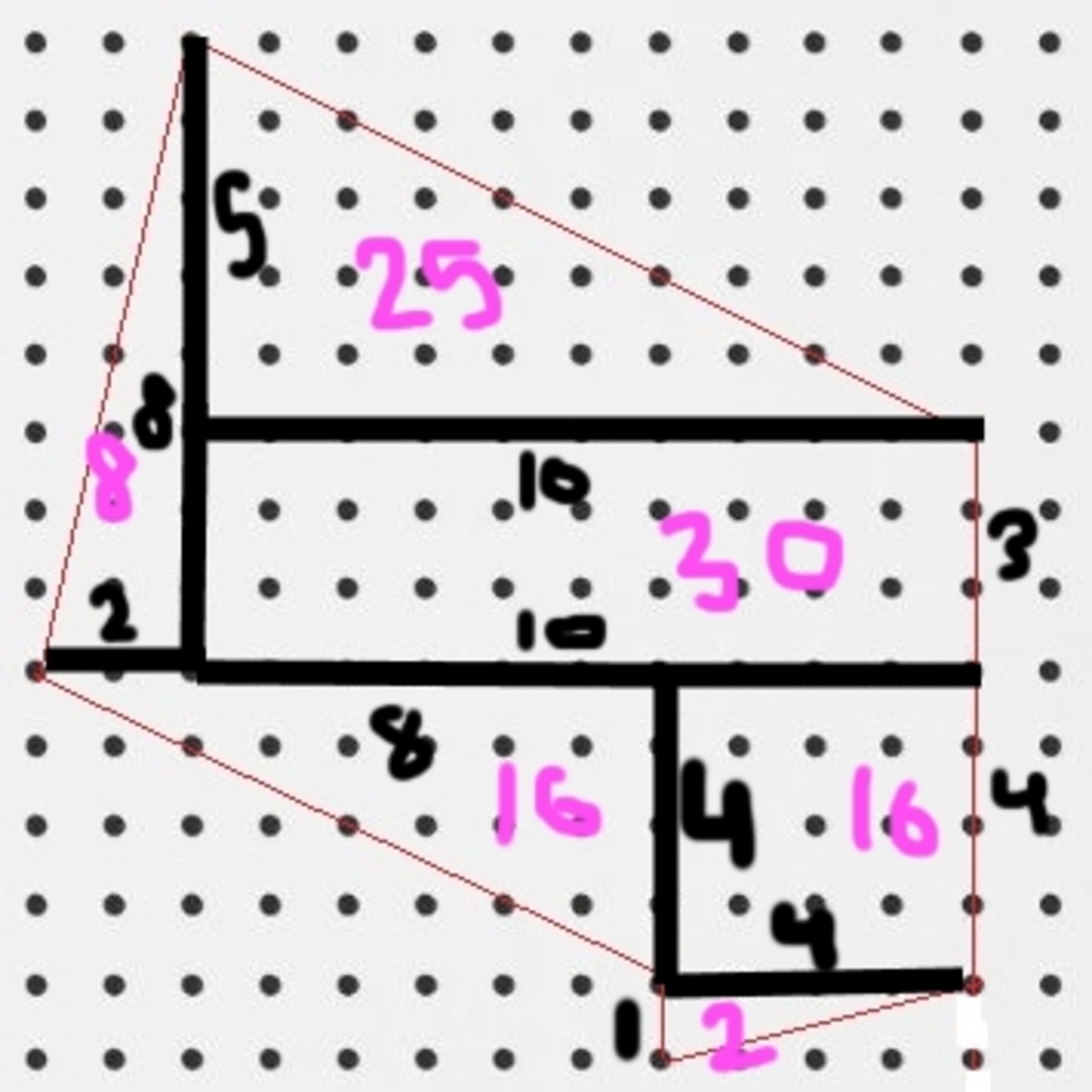
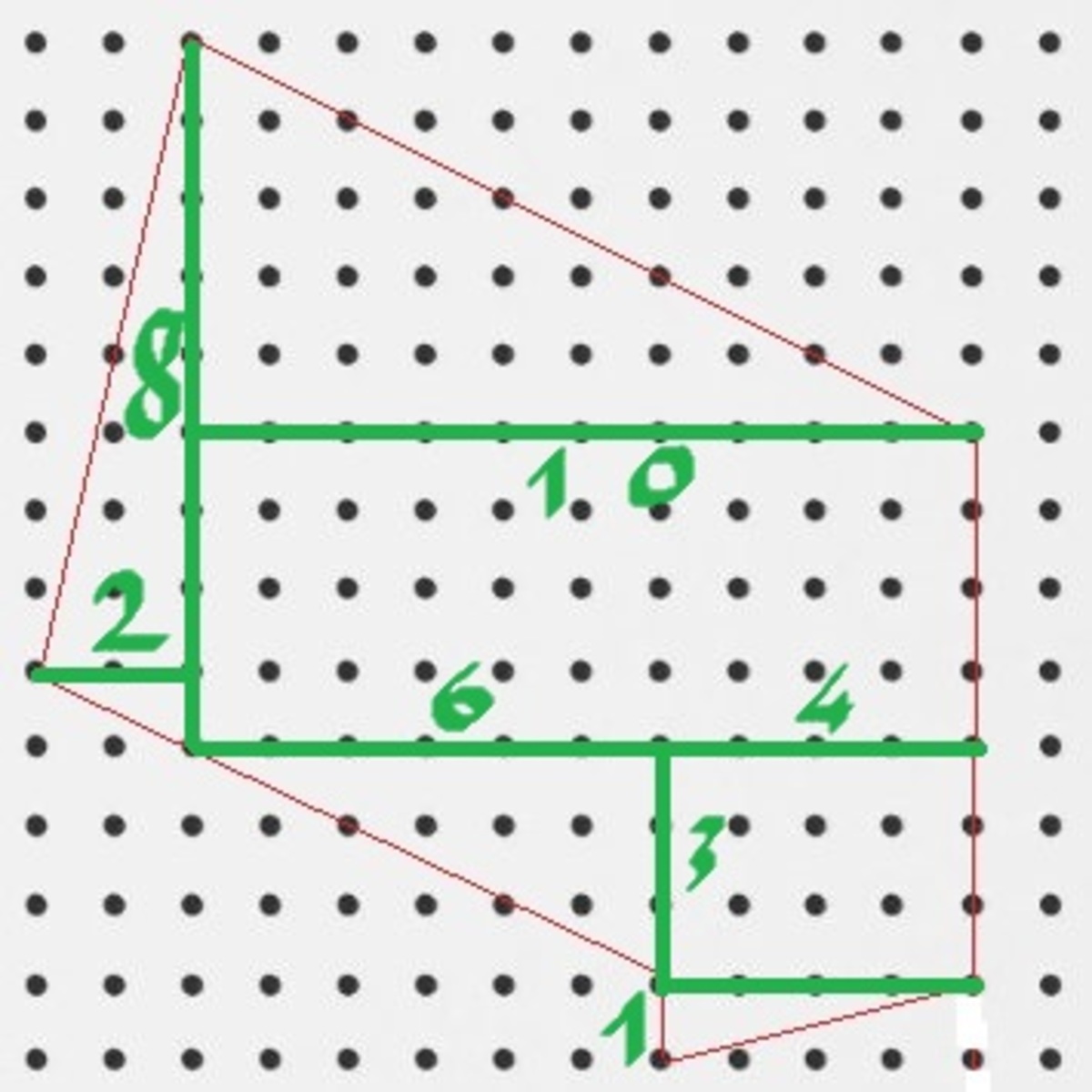
First find the area of the square with green boundary which is (13 * 12=156).
Now subtract the area of the 3 triangle and one trapezium which are labelled as 1,2,3 and 4 respectively . The areas are:
(1) 8 * 2/2=8
(2) 10 * 5/2=25
(3) (5+1) * 8/2=24
(4) 4 * 1/2=2
The area enclosed by the string is 156- 8 - 25 -24 - 2=97