Area enclosed by two curves, part 2
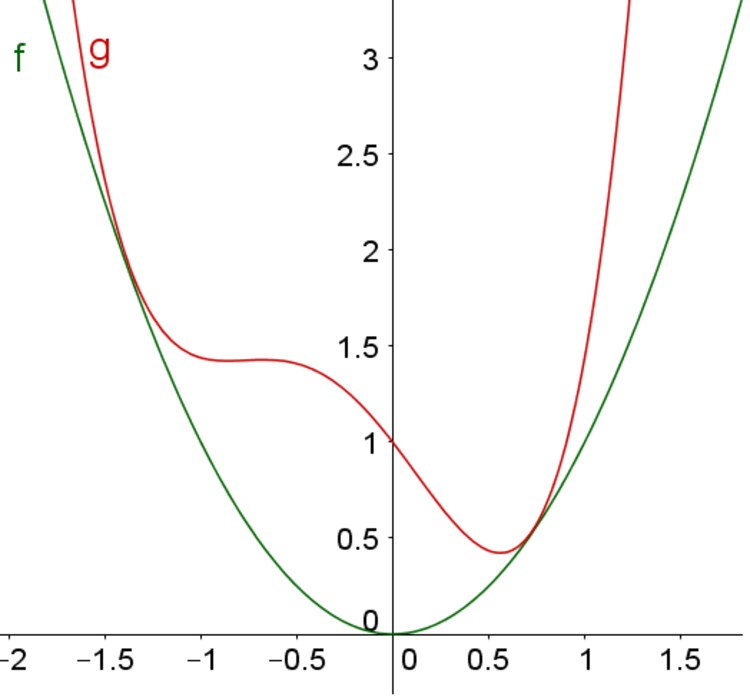 The parabola
is tangent to the graph of
at two distinct points. Given that the
area enclosed by these two curves is
, where
and
are coprime positive integers, find the value of
.
The parabola
is tangent to the graph of
at two distinct points. Given that the
area enclosed by these two curves is
, where
and
are coprime positive integers, find the value of
.
Remark : try the case for here
This problem is part of Curves... cut or touch?
The answer is 31.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
for the minimum area g(x) should be symmeyric about y axis as parabola so b= 0 =a now at point of inter section x^2=x^4+cx^2+1 as they touch this equation have pair of coinside root so c=-1 eqution reduce to x^4-2x^2+1=0 ie (x^2-1)^2=0 x=1,1,-1,-1 and requred area $x^4-x^2+1-x^2dx from x=-1 to x=1 = 16/15 p=16 ,q=15 so p+q= 15+16=31