area of a regular six pointed star
Geometry
Level
3
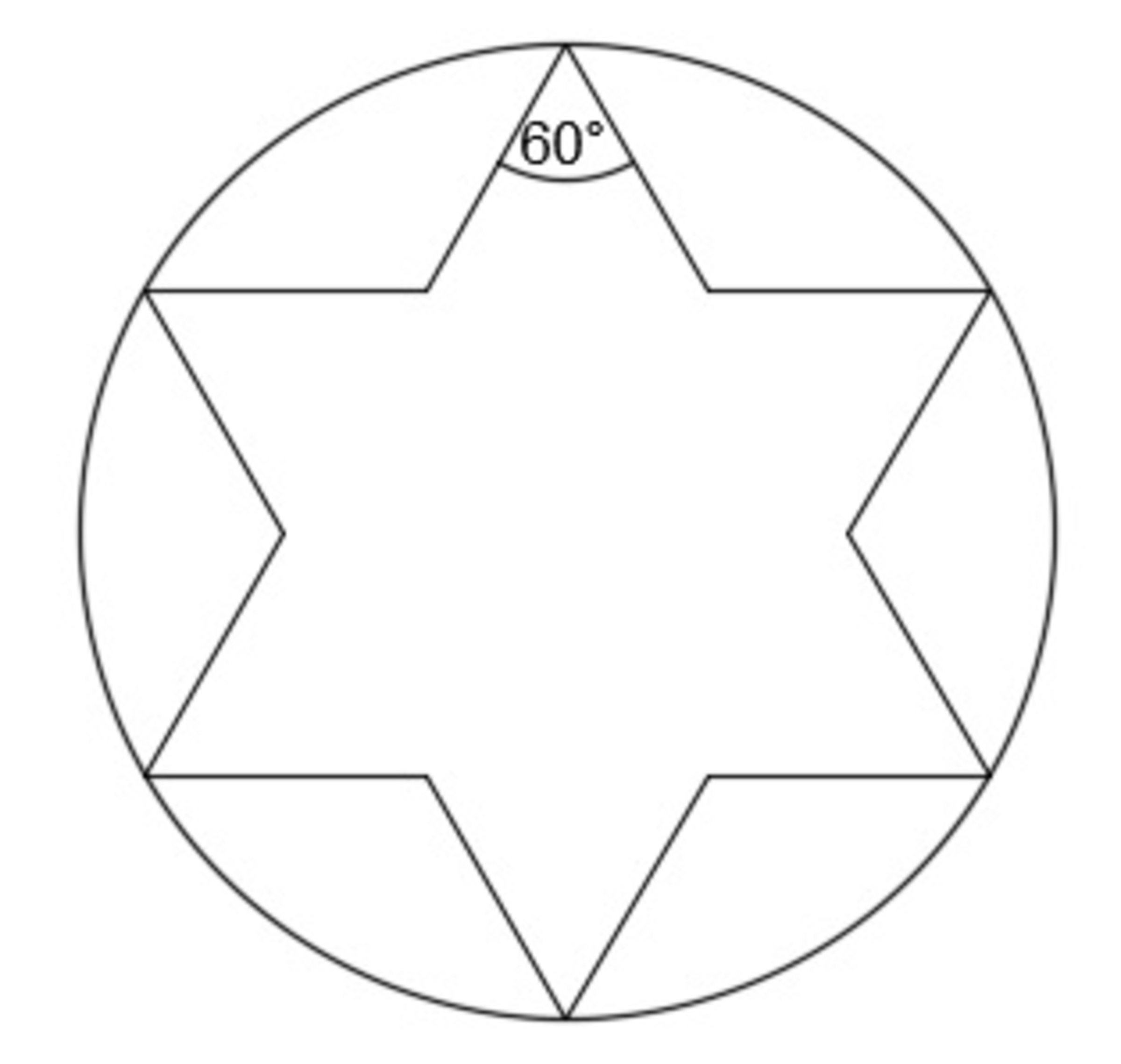 A regular six pointed star is inscribed in a circle of radius 4. Find the area of this regular six pointed star.
A regular six pointed star is inscribed in a circle of radius 4. Find the area of this regular six pointed star.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Firstly, find the area of the large equilateral triangle that is inscribed in the circle. Draw lines from the centre to each of the three vertices of the triangle. The area is then 3 ⋅ 2 1 ( 4 ) ( 4 ) sin 1 2 0 º = 1 2 3 using the formula A = 2 1 a b sin C .
Then subdivide the figure into 12 smaller equilateral triangles. The large equilateral triangle covers 5 + 3 + 1 = 9 of these smaller triangles. Therefore, the area of the figure is 1 2 3 ⋅ 9 1 2 = 1 6 3 .