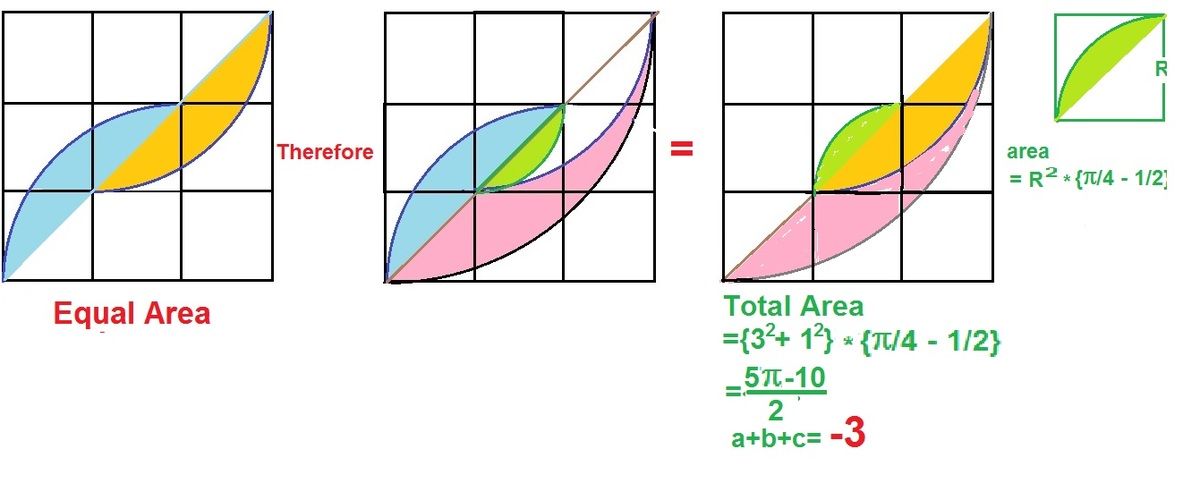
Area Of A Sickle
In the above diagram, each of the grid squares is a unit long. Also, all the arcs are quadrants. If the area of the shape enclosed by arcs D B , B J , J K , and K D can be represented as c a π + b , where a , b , c are integers with c positive, find the maximum value of a + b + c .
The answer is -3.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
Did you get this idea from "MIndYourDecisions" - youtube channel?
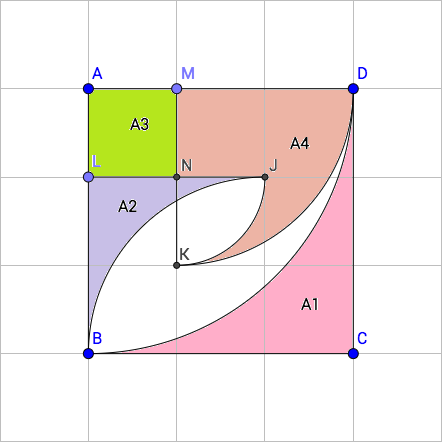
We have split this diagram into 4 areas to find the area of the exterior part. Note that the total square area is 9.
The area of A1 is 9 − 4 9 π , which can be shown by noticing the area of the quadrant D A B is 4 9 π .
Similarly, the area of A2 is 4 − π .
The area of A3 is 1.
The area of A4 can be determined by finding the area of the of the quadrant that defines it's largest arc ( D K ), and subtracting the area of the quadrant defines the smallest arc ( J K ). Thus, A4 is equal to π − 4 π = 4 3 π
Thus, the total exterior area is 9 − 4 9 π + 4 − π + 1 + 4 3 π = 1 4 − 2 5 π . Thus, the area of the sickle is 9 − ( 1 4 − 2 5 π ) = 2 5 π − 1 0 . Thus, a = 5 , b = − 1 0 and c = 2 , so a + b + c = − 3 .
I approached this the same way. I did attempt integration , especially to find the area between A4 and A1 but turned out to be too complex , at least for this problem.
The above seems indirectly related to fractals such as Seiperenski Gasket etc and associated Iterated Function Systems
We can use the integration rule to systematically find the area A as follow.
A = B → B ∫ f ( x ) d x = B → J ∫ f ( x ) d x − J → K ∫ f ( x ) d x + K → D ∫ f ( x ) d x − D → B ∫ f ( x ) d x = π − ( 2 − 4 π ) + ( 6 − π ) − ( 9 − 4 9 π ) = 2 5 π − 5 = 2 5 π − 1 0 − sign for reverse direction
⟹ a + b + c = 5 − 1 0 + 2 = − 3
Nice solver tremendous
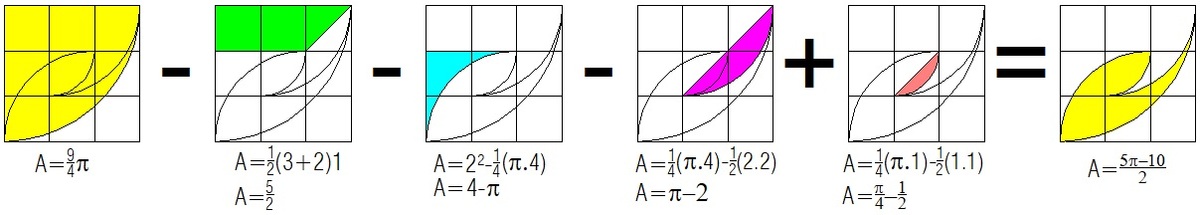 The desired answer is
5
−
1
0
+
2
=
−
3
The desired answer is
5
−
1
0
+
2
=
−
3
Define a crescent to be a quarter circle minus the triangle formed by its 3 vertices. For example, [ Crescent B D ] = [ Quadrant A B D ] − [ △ A B D ]
Remove crescent KJ and translate and rotate crescent BJ to crescent KD. Now we can see that the area is made up of crescent KJ and crescent BD. By similarity, [ crescent B D ] = 9 [ crescent K J ] . Area = [ crescent B D ] + [ crescent K J ] = 1 0 [ crescent K J ] = 1 0 [ 4 π − 2 1 ( 1 ) ( 1 ) ] = 2 5 π − 1 0