Area of a star inside a unit square

Label the figure as above. We note that $\triangle CBE$ and $\triangle BEF$ are similar. Therefore $\dfrac {EF}{BE} = \dfrac {BE}{BC} \implies EF = \dfrac {BE}{BC} \times = \dfrac {\frac 12}1 \times \dfrac 12 = \dfrac 14$ .

Then the area of $\triangle BEF$ , $A_{\triangle BEF} = \dfrac 12 \times \dfrac 12 \times \dfrac 14 = \dfrac 1{16}$ . The area of white region $A_{\text{white}} = 8 A_{\triangle BEF} = 8 \times \dfrac 1{16} = \dfrac 12$ . The area of the red region $A_{\red{\text{red}}} = A_{\text{square}} - A_{\text{white}} = 1 - \dfrac 12 = \boxed {\frac 12}$ .

Area of each white triangle is $\dfrac{1}{2}\times 1\times \dfrac{1}{4}=\dfrac{1}{8}$ . So total area of the four white triangles is $4\times \dfrac{1}{8}=\dfrac{1}{2}$ . Therefore area of the red region is $1-\dfrac{1}{2}=\boxed {\dfrac{1}{2}}$ .

To find the area of the red section, you must determine the area of the white triangles combined, subtract this from the total area of the square.

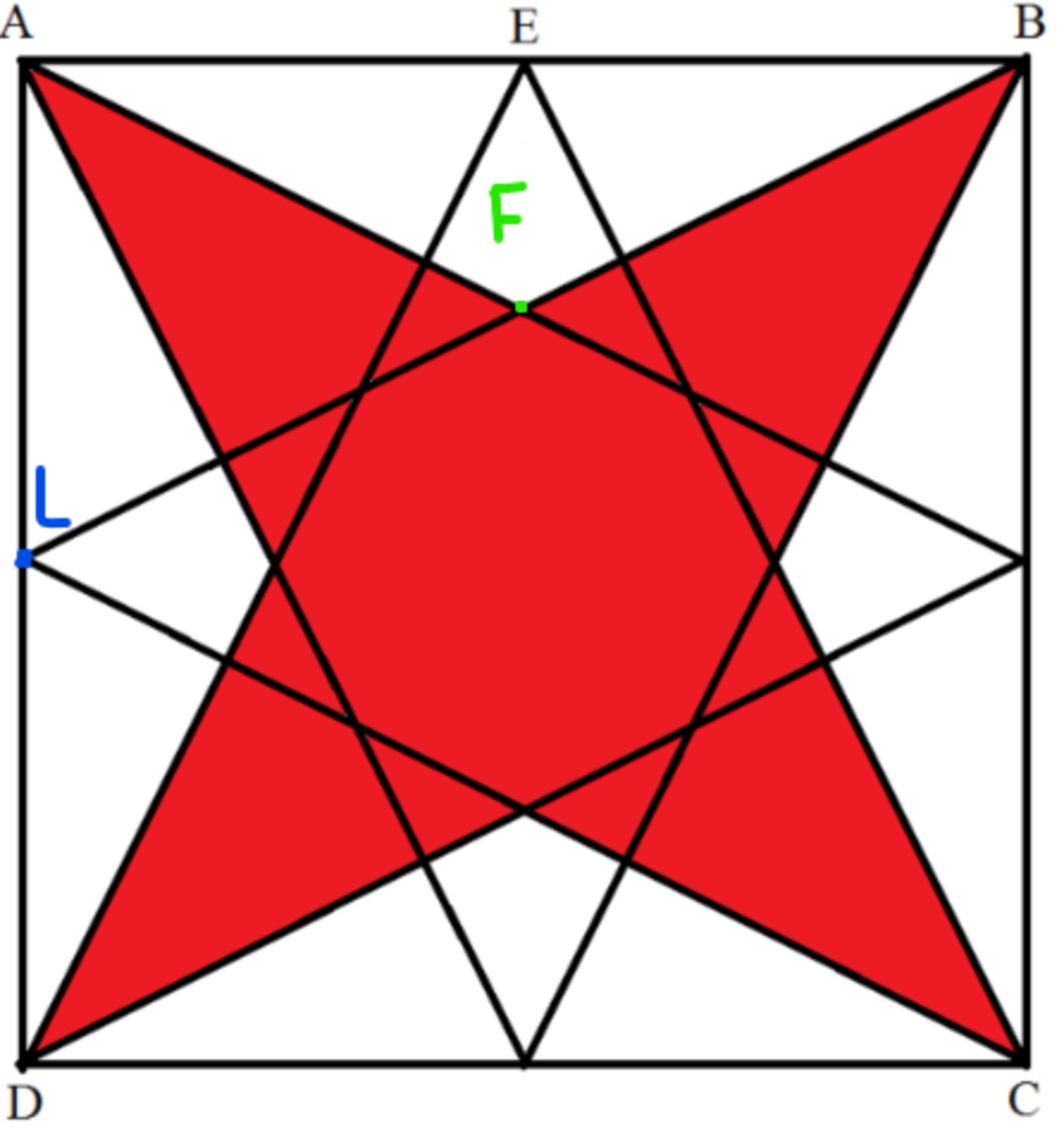
$AE$ = $\frac{1}{2}$ , meaning that $AB$ = 1. $AL$ = $\frac{1}{2}$ , meaning that $EF$ = $\frac{1}{2}$ of $AL$ , or $\frac{1}{4}$ .

We now have $AB$ and $EF$ , meaning that we know the $base$ and $height$ of the top white triangle. The formula for the area of a triangle is $\frac{base * height}{2}$ , so the area of the white triangle would be ( $\frac{1}{4}$ x 1) ÷ 2 = $\frac{1}{8}$ . All of the white triangles are congruent, and there are 4 of them, meaning $\frac{1}{8}$ x 4 = $\frac{1}{2}$ = the total white triangle area.

The total area of the square (1 x 1 = 1) minus the combined area of the white triangles = 1 - $\frac{1}{2}$ = $\frac{1}{2}$ = the area of the red figure.