Area of a triangle (hard)
Find the area of the triangle whose vertices are O(0,0), A(6,3) and B(1, -7).
The answer is 22.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
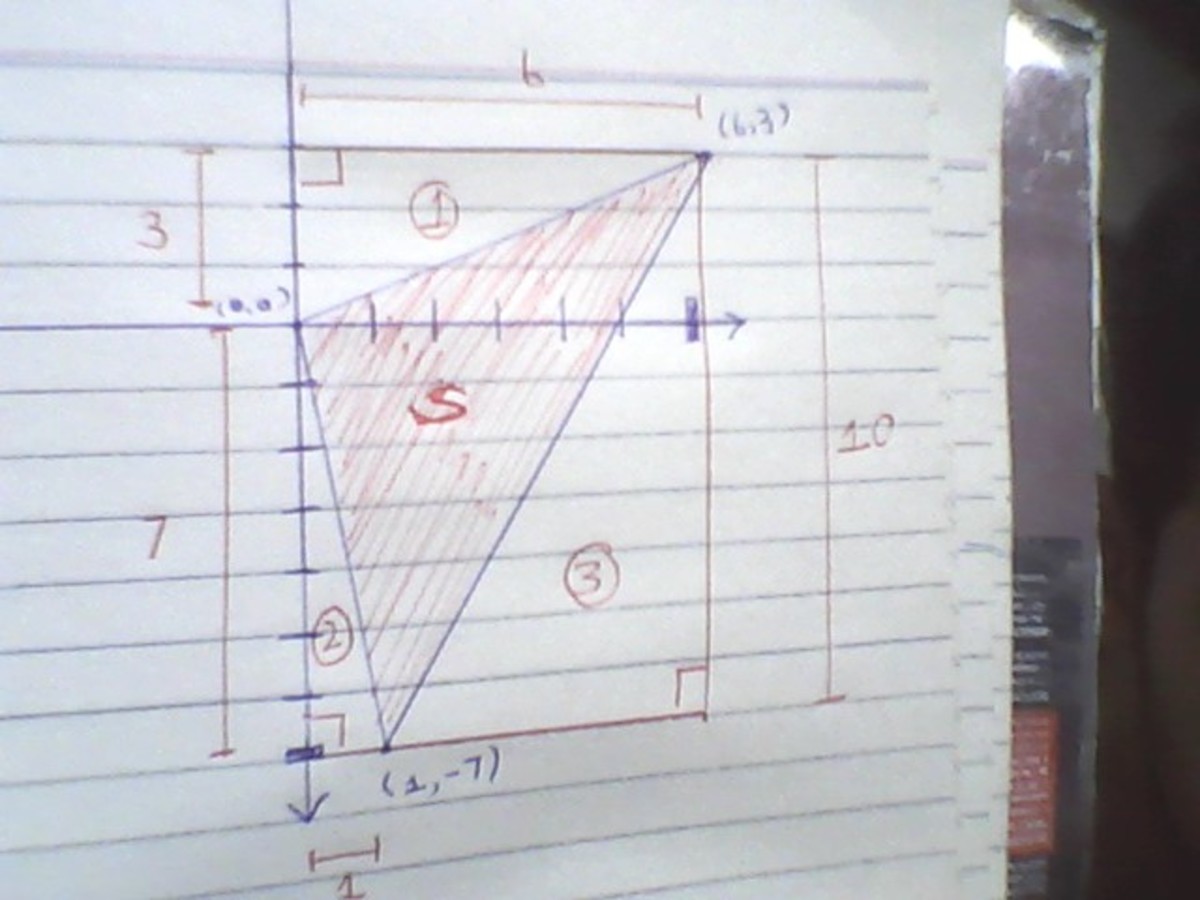 total area =
10 * 6 =>60
total area =
10 * 6 =>60
area(1)= 2 1 *3 * 6 => 9
area(2)= 2 1 *7 * 1 => 3.5
area(3)= 2 1 *5 * 10 => 25
=>s=60 - 25 - 3.5 - 9 => 22.5
± 2 1 ⎣ ⎢ ⎢ ⎡ 0 6 1 0 0 3 − 7 0 ⎦ ⎥ ⎥ ⎤ = 2 2 . 5
Let us consider OA as base.
then, length of base = 6 2 + 3 2 = 4 5 .
slope of base = 3/6 = 1/2.
As we have to find the length of perpendicular onto the base,
slope of BD = -1/(slope of base) = -1/(1/2) = -2.
using slope-point form,
Equation of BD, 2x + y = -5.
Equation of base ( using slope-point form), x - 2y = 0.
Their intersection is (-2,-1).
thus distance BD = 6 2 + 3 2 = 4 5 .
Thus area of the triangle is 45/2 = 2 2 . 5