Area of Lunar Moons (1)

Refer to the given diagram: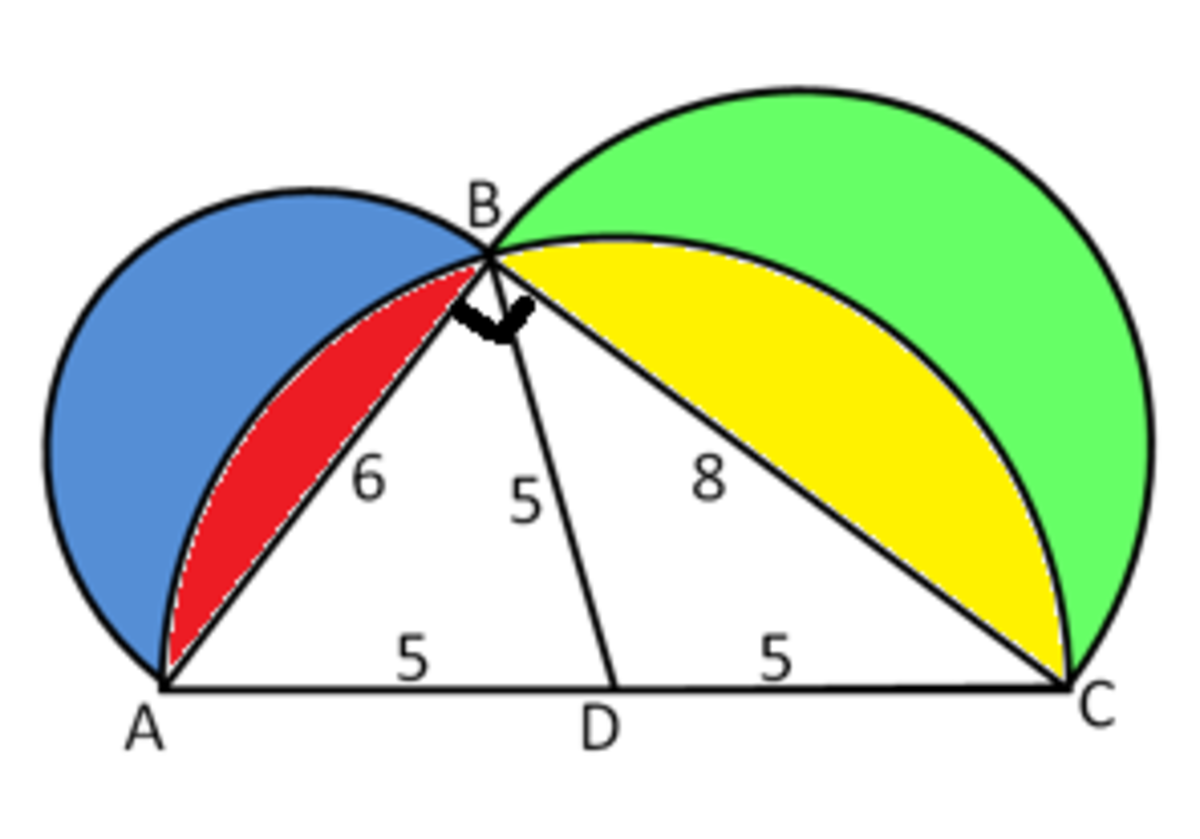

Area of the biggest semicircle $=\dfrac{\pi\text{R}^2}{2}=\dfrac{25\pi}{2}$

Area of $\Delta\space \text{ABC}=\dfrac{1}{2}\times\space 6\times\space 8=24$ (As it is right angled)

Area of Red region $+$ Area of yellow region $=$ $\dfrac{25\pi}{2}-24=\dfrac{25\pi-48}{2}$ $....(1)$

Now,

Area of $2^{nd}$ largest semicircle $=$ Area of Yellow region $+$ Area of Green region $=\dfrac{\pi\text{R}^2}{2}=\dfrac{16\pi}{2}.....(2)$

Similarly,

Area of $3^{rd}$ largest semicircle $=$ Area of Red region $+$ Area of Blue region $=\dfrac{\pi\text{R}^2}{2}=\dfrac{9\pi}{2}.....(3)$

Area of the lunar moons $=(2)+(3)-(1)=\dfrac{16\pi}{2}+\dfrac{9\pi}{2}-\dfrac{25\pi-48}{2}=\dfrac{25\pi}{2}-\dfrac{25\pi-48}{2}=\dfrac{48}{2}=\boxed{24}$