area of the shaded portion
Geometry
Level
1
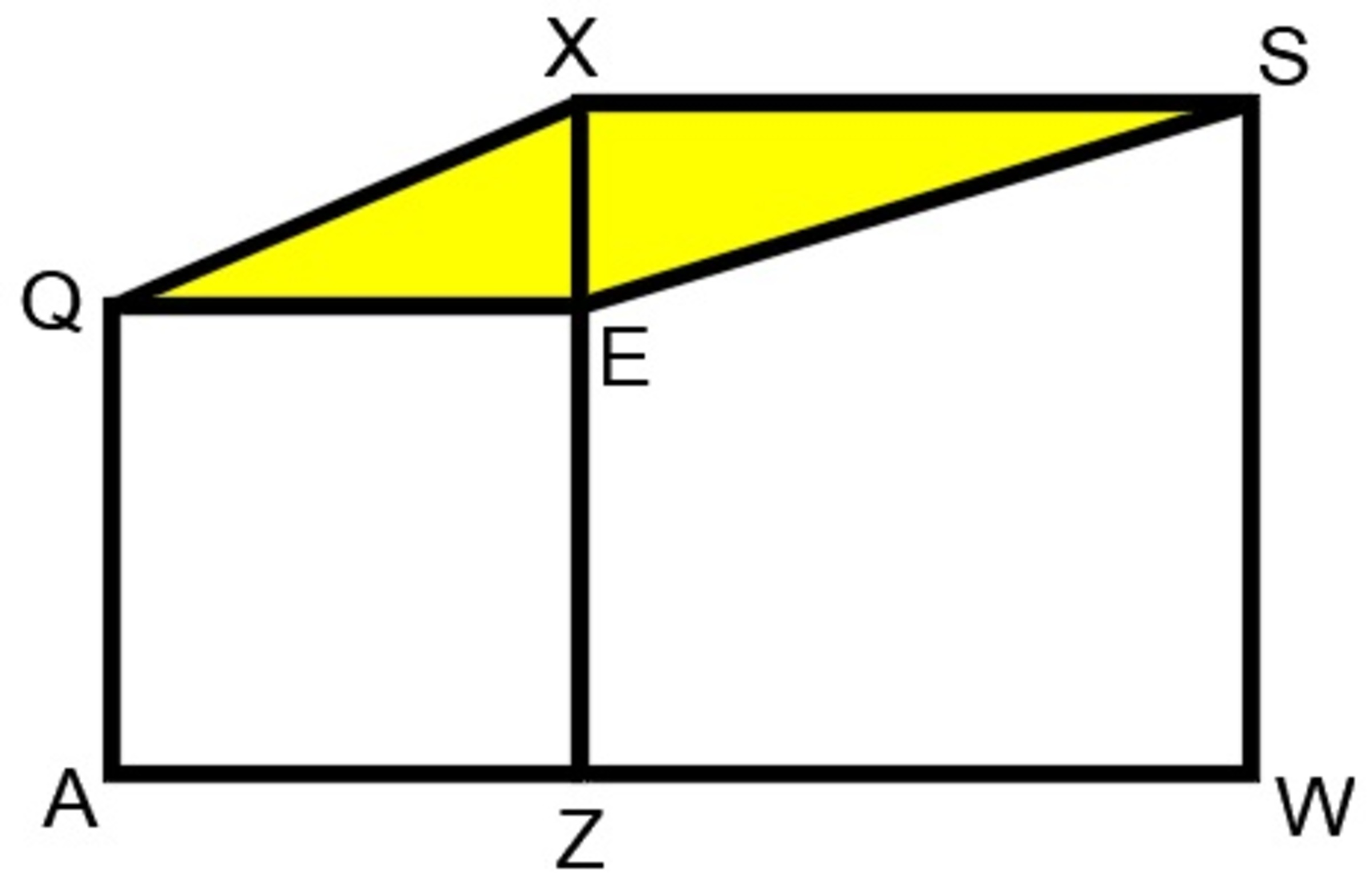
The difference of the areas of square XSWZ and square QEZA is 273 square units. Find the area of quadrilateral XSEQ (shaded yellow).
156.3 square units
165.3 square units
136.5 square units
135.6 square units
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let x = Z W , the side length of the larger square, and y = A Z , the side length of the smaller square.
We are given x 2 − y 2 = 2 7 3 .
Now consider the area of the yellow trapezoid X S E Q . (First, we must note that this is a trapezoid, since Q E ∥ X S .)
The area formula for a trapezoid is A = 2 ( b 1 + b 2 ) h , namely the height times the average of the two base lengths.
Here h = X E = ( x − y ) , b 1 = X S = x and b 2 = Q E = y .
Thus,
A = 2 ( x + y ) ( x − y ) = 2 x 2 − y 2 = 2 2 7 3 .