Area Of Triangles

Area of lighter blue equals area of lighter green, area of darker blue equals area of darker green.

Reasons: Both pairs have a height of 2. Both have a vertex in common and the same angle at that vertex. Both are pairs of right triangles. Therefore they are congruent (within a pair).

Relevant wiki: Triangles

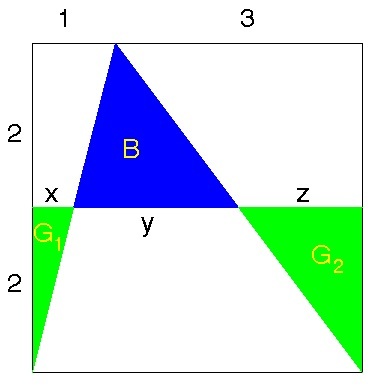let $B$ be the area of the blue region, $G_1$ be the area of the green region (leftmost) and $G_2$ be the area of the green region (rightmost)

By ratio and proportion (along the leftmost green region), we have

$\dfrac{x}{2}=\dfrac{1}{4}$

$x=\dfrac{1}{2}$

It follows that,

$G_1=\dfrac{1}{2}(2)(\dfrac{1}{2})=\dfrac{1}{2}$

By ratio and proportion again (along the right most green region), we have

$\dfrac{z}{2}=\dfrac{3}{4}$

$z=\dfrac{3}{2}$

It follows that,

$G_2=\dfrac{1}{2}(2)(\dfrac{3}{2})=\dfrac{3}{2}$

Therefore, $G_1+G_2=\dfrac{1}{2}+\dfrac{3}{2}=\dfrac{4}{2}=2$

By ratio and proportion again (along the blue region), we have

$\dfrac{z}{4}=\dfrac{2}{4}$

$z=2$

It follows that,

$B=\dfrac{1}{2}(2)(2)=2$

Compare:

$G_1+G_2=B$

$2=2$

We therefore conclude that the area of the blue region is equal to that of the green region.