Area Problem I

In △ A B C as shown above, ∠ C = 9 0 ∘ , A D and B E , which intersect at I , are bisectors of ∠ B A C and ∠ A B C respectively. If the area of △ I A B is 6 , find the area of quadrilateral A B D E .
The answer is 12.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
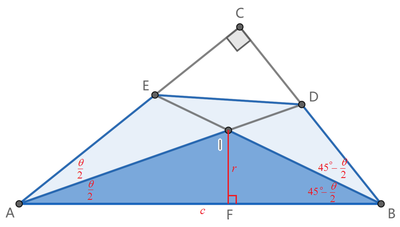
Let ∠ A = θ ; then ∠ B = 9 0 ∘ − θ , ∠ I A B = 2 θ and ∠ I B A = 4 5 ∘ − θ . Let A B = c and I F be perpendicular to A B with length r . Then
c ⟹ r r c = r cot 2 θ + r cot ( 4 5 ∘ − 2 θ ) = r ( t 1 + 1 − t 1 + t ) = 1 + t 2 c t ( 1 − t ) = 1 + t 2 c 2 t ( 1 − t ) = 1 2 Let t = tan 2 θ Since [ I A B ] = 2 r c = 6
Now the area of △ A B C ,
[ A B C ] = 2 B C × A C = 2 c 2 sin θ cos θ = 2 c 2 × 1 + t 2 2 t × 1 + t 2 1 − t 2 = ( 1 + t 2 ) ( 1 + t 2 ) c 2 t ( 1 − t ) ( 1 + t ) = 1 + t 2 1 2 ( 1 + t ) Recall 1 + t 2 c 2 t ( 1 − t ) = 1 2
And the area of △ C D E .
[ C D E ] = 2 C D × C E = 2 A C tan 2 θ × B C tan ( 4 5 ∘ − 2 θ ) = 2 c 2 sin θ cos θ tan 2 θ tan ( 4 5 ∘ − 2 θ ) = 1 + t 2 1 2 ( 1 + t ) × 1 + t t ( 1 − t ) Recall 2 c 2 sin θ cos θ = 1 + t 2 1 2 ( 1 + t )
Therefore, the area of quadrilateral A B D E ,
[ A B D E ] = [ A B C ] − [ C D E ] = 1 + t 2 1 2 ( 1 + t ) ( 1 − 1 + t t ( 1 − t ) ) = 1 + t 2 1 2 ( 1 + t ) ( 1 + t 1 + t 2 ) = 1 2
Let a = B C , b = A C , and c = A B . Since △ A B C is a right triangle, a 2 + b 2 = c 2 , and since I is at the intersection of the angle bisectors of △ A B C , it is the incenter of △ A B C with an incircle radius of r = a + b + c a b . The area of △ I A B is then A △ I A B = 2 1 c r = 2 ( a + b + c ) a b c .
Now place and rotate the diagram on a coordinate graph so that C is on the origin, A is on the x -axis, and B is on the y -axis.
Then the line through B ( 0 , a ) and I ( a + b + c a b , a + b + c a b ) has an equation of y = − a b a + c x + a , so that E is the x -intercept at E ( a + c a b , 0 ) , which means C E = a + c a b . By a similar argument, C D = b + c a b .
The area of quadrilateral A B D E is A A B D E = A △ A B C − A C D E = 2 1 a b − 2 1 a + c a b b + c a b , which simplifies to A A B D E = a + b + c a b c = 2 A △ I A B .
Since A △ I A B = 6 , A A B D E = 2 ⋅ 6 = 1 2 .