Area swept by a variable line segment
You have two circles. The green circle is centered at the origin and is of radius . The blue circle is centered at and is of radius . A point on the green circle starts at the top of the circle and a point on the blue circle starts at the right of the circle. A gray line segment connects the two points. The points rotate, in the counter clockwise direction, on their circles with the same angular velocity, that is, when the green circle point completes one revolution, the blue circle point completes one revolution as well.
Find the area of the region swept by the gray line segment in one cycle of the two circles points motion.
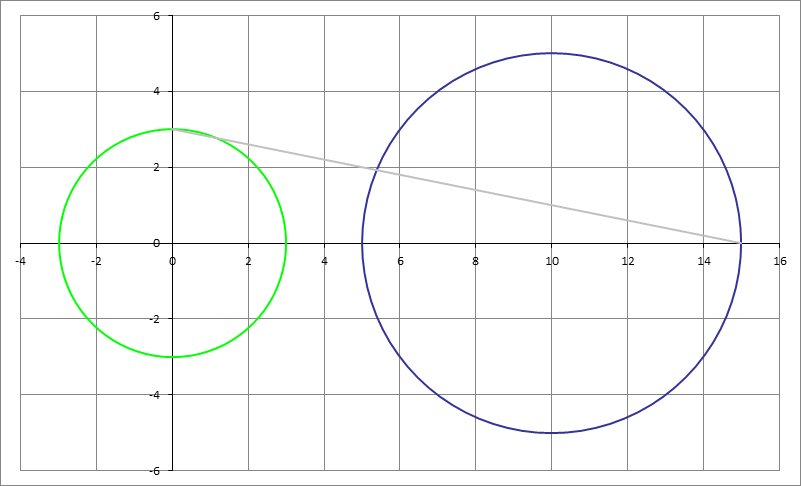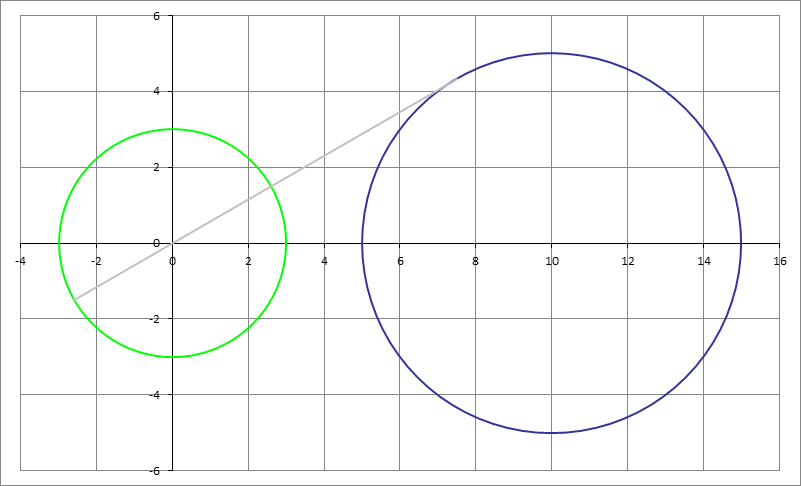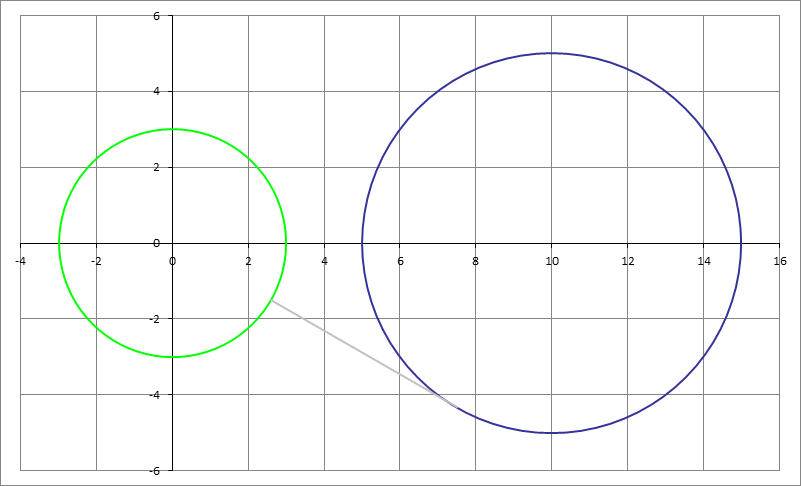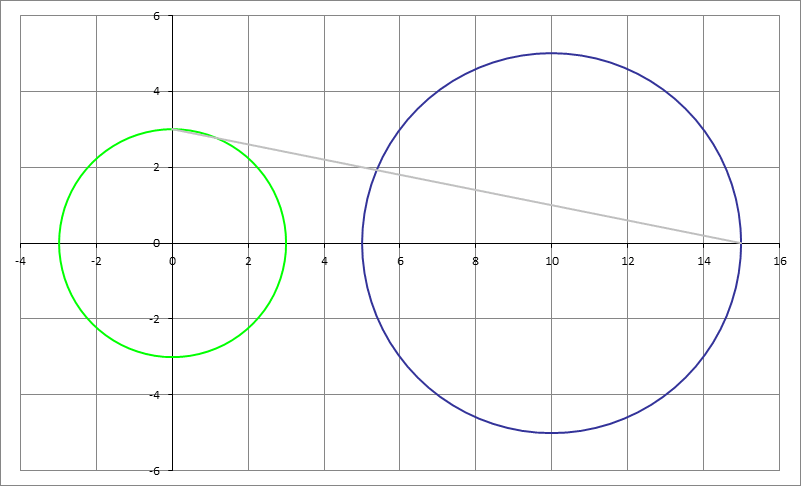
The answer is 123.23.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let C1 : (3cos(θ+π/2),3sin(θ+π/2)); C2 : (10+cos(θ),sin(θ)) in parametric form. Let L be the line joining a point on C1 to it's respective point in C2. The equation of L on simplifying will be, (5x-3y)sin(θ)+(3x+5y-30)cos(θ)+(15-10y)=0. Thus L represents a family of lines which are defined from the parameter θ. The curve which will be swept out from family L, is essentially the envelope of the family L(ie, the curve will touch every member of L at some point). Let curve S be the envelope, then eqn of S will be (5x-3y)²+(3x+5y-30)²=(15-10y)² => 66y²=34x²-180x+675. After this point, it is just calculation, S and C1 touch at x=0.9 and S and C2 touch at x=7.5 . Just find (area under C1 from -3 to 0.9)+(area under S from 0.9 to 7.5)+(area under C2 from 7.5 to 15) by integration. Final answer should be 123.2299 .