Astronaut Losing Her Oxygen
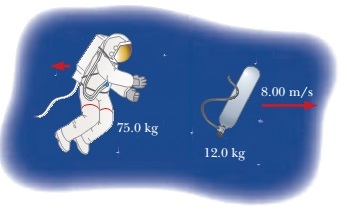An astronaut in her space suit has a total mass of 87.0 kg, including a suit and an oxygen tank. Her tether line loses its attachment to her spacecraft while she’s on a spacewalk. Initially at rest with respect to her spacecraft, she throws her 12.0 kg oxygen tank away directly opposite from the direction to the spacecraft with a speed of 8.00 m/s to propel herself back toward it.
Determine the maximum distance (in meters) she can be from the craft and still return within 2.00 min (the amount of time the air in her helmet remains breathable).
The answer is 154.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Its a question of conservation of momentum. The momentum of the gas cylinder is,
-12 * 8 (kg.m/s)
If we take the direction towards the space ship as positive.
Momentum of the astronaut,
75 * v (kg.m/s)
Since it is a isolated system hence the total momentum is conserved.
So,
The final momentum of the entire system = the initial momentum = 0. ( Wrt the space craft)
Hence,
-96 + 75*v = 0
Which gives,
v = 1.28 m/s
Using this and,
Distance= speed * time
We get,
Distance = 153.6 ..
Rounding off to nearest integer,
Distance = 154