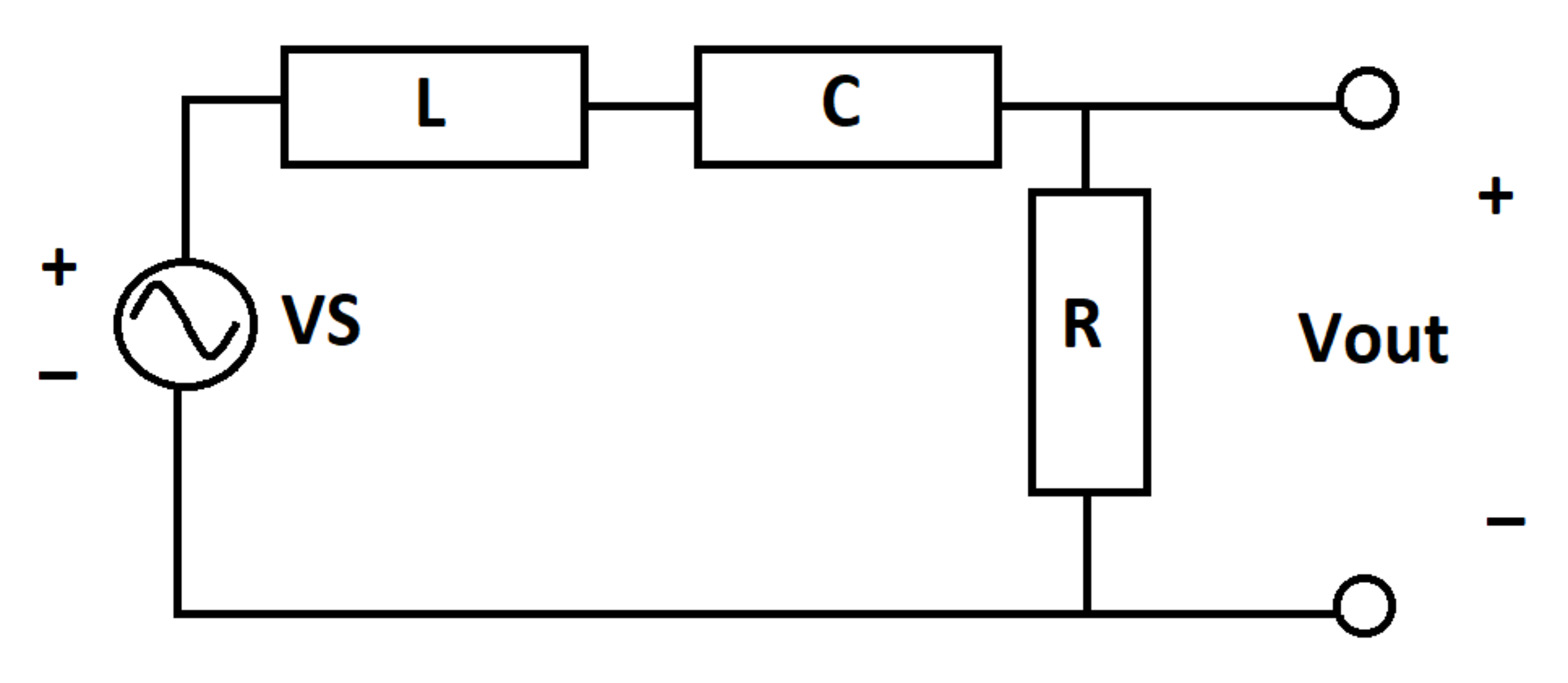
Band Pass Filter Charging Transient

Electricity and Magnetism Level pending

An $RLC$ band pass filter takes a sinusoidal voltage input $V_S(t)$ and produces an output voltage $V_{out} (t)$ .

$V_S(t)$ is as follows:

$V_S(t) = 10 \sin(t)$

At time $t = 0$ , the inductor and capacitor are de-energized. Determine the following integral:

$\int_0^{40 \pi} | V_S(t) - V_{out} (t) | \, dt$

Details and Assumptions:
1) $R = 0.1$
2) $L = 1$
3) $C = 1$
4) In the integral, $|\cdot|$ denotes the absolute value of a scalar

The answer is 127.2.

1 solution

Karan Chatrath
Dec 13, 2019

Let $Q$ be the charge on the capacitor and $V$ be the output voltage. The equations for this circuit are:

$\red{L\dot{I} + \frac{Q}{C} + IR = V_S} \ ; \ \blue{\dot{Q}= I} \ ; \ \green {V = IR}$

By manipulating and rearranging the above equations in order to obtain a dynamic mapping between $V_S$ and $V$ , one gets:

$10\ddot{V} + \dot{V} + 10V = 10\cos{t}$

Subject to: $V(0) = \dot{V}(0) = 0$ . This equation can be solved using any standard techniques and has a closed form solution:

$V = 10\sin{t} -\frac{200}{\sqrt{399}}e^{-\frac{t}{20}}\sin\left(\frac{t\sqrt{399}}{20}\right)$

From here, the required integral to be computed is shown below. This evaluation is done numerically.

$\boxed{I = \int_{0}^{40\pi} \left\lvert \frac{200}{\sqrt{399}}e^{-\frac{t}{20}}\sin\left(\frac{t\sqrt{399}}{20}\right) \right\rvert dt \approx 127.2}$

Hello. I just solved your latest problem on the band stop filter. I attempted to post my solution but I deleted it by mistake and now cannot post one. I am tempted to share my work as a comment but am not sure if I should. I do not mind posting it but given that the method and answer becomes visible, it might be unfair for future solvers.

Karan Chatrath - 1 year, 5 months ago

I could post it in the report section, even though it is not a report. Would that be okay?

Karan Chatrath - 1 year, 5 months ago

Band Pass Filter Charging Transient

The answer is 127.2.

1 solution

0 pending reports