Bangle in a triangle
Geometry
Level
2
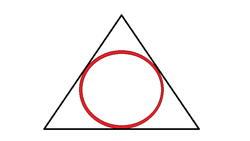 The inradius of an equilateral triangle is
, then what is the perimeter of the triangle?
The inradius of an equilateral triangle is
, then what is the perimeter of the triangle?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
There is a simplified formula for the area of any triangle, A = r s where r is inradius and s is semiperimeter. Let the side of equilateral trianglebe a then, area of triangle = 4 3 a 2 From the above formula we have 3 × 2 3 a = 4 3 a 2 on simplifying we get a = 6 so 3 a = 1 8