Basic 4-Resistor, 1-Capacitor Circuit

Electricity and Magnetism Level 2

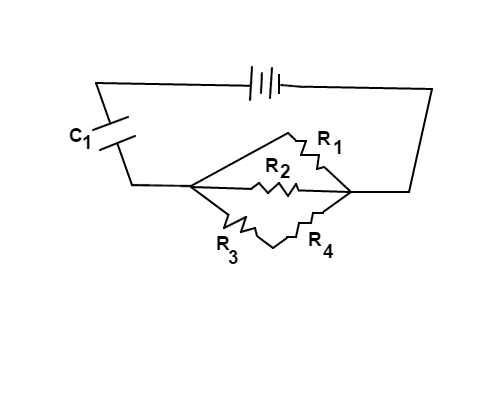
A simple RC circuit was prepared with one capacitor ( C C ) and four resistors ( R R ), as depicted in the figure shown above. All resistors have resistances of 2 Ω 2 \text{Ω} ; the capacitor's capacitance is 9 F 9 \text{F} .

Determine the time constant τ \tau in seconds.

H i n t : τ = R C Hint: \tau = R C

David's Electricity Set


The answer is 7.2.

David Hontz by David Hontz , 26, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

David Hontz
Jan 10, 2017

Resistors in Series Simplication: R a = R 3 + R 4 = 2 + 2 = 4 Ω R_a = R_3 + R_4 = 2+2 = 4 \text{Ω} Resistors in Parallel Simplication: R b = ( 1 R 1 + 1 R 2 + 1 R a ) − 1 = ( 1 2 + 1 2 + 1 4 ) − 1 = 0.8 Ω R_b = \Big( \frac{1}{R_1} + \frac{1}{R_2} + \frac{1}{R_a} \Big) ^{-1} = \Big( \frac{1}{2} + \frac{1}{2} + \frac{1}{4} \Big) ^{-1} = 0.8 \text{Ω}

Time Constant Calculation: τ = R b C = 0.8 Ω × 9 F = 7.2 s \tau = R_bC= 0.8 \text{Ω} \times 9\text{F} = \boxed{7.2 \text{s}}

3 Helpful 0 Interesting 0 Brilliant 0 Confused

It's pretty hard to imagine a capacitor of that capacitance!

Akshat Sharda - 3 years, 9 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...