Basics Of Rolling
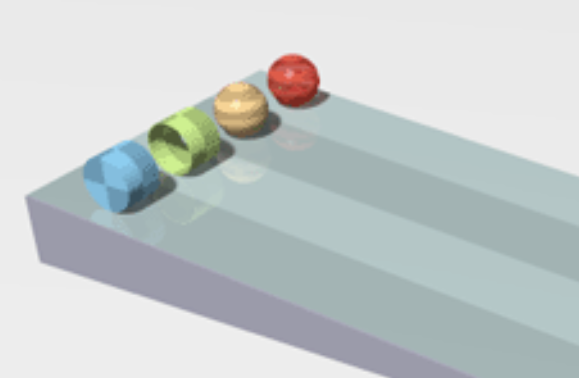
If a hollow sphere, a solid sphere, a hollow cylinder and a solid cylinder are released from the same height as shown in the above figure then which will reach the ground first.
Assume that the inclination is perfectly rough and all are doing pure rolling motion.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
For any body of mass M and radius R , we can write it's moment of inertia as M K 2 , where K is the radius of gyration for that body. This argument is enough for the bodies we will be considering.
Now, for any body, the force that accelerates it is the component of it's weight along the inclined, which can be written as M g sin θ where θ is the angle, the incline makes with the horizontal. Since, the body rolls perfectly, hence, there must be an opposite frictional force. Let that force be f . This frictional force decelerates the body linearly, but provides it an accelerating torque.
Writing down the equations of motion along the plane:
M g sin θ − f = M a
where a is the linear acceleration of the body.
Secondly, for rotational motion:
f × R = M K 2 α
where α is the angular acceleration of the body.
Now, using the relation α = R a in the second equation, we get
f = M a R 2 K 2
Using this in the first equation, we can easily get:
a = 1 + R 2 K 2 g sin θ
Hence, the body with more radius of gyration will have a lower acceleration, and hence will take more time to roll down the incline.
Comparing the radii of gyration of all the given bodies, we can easily make out that
K S o l i d S p h e r e = 5 2 < K H o l l o w S p h e r e = 3 2 < K S o l i d C y l i n d e r = 2 1 < K H o l l o w C y l i n d e r = 1
And so,
t i m e S o l i d S p h e r e < t i m e H o l l o w S p h e r e < t i m e S o l i d C y l i n d e r < t i m e H o l l o w C y l i n d e r