Bead on a wavy wire
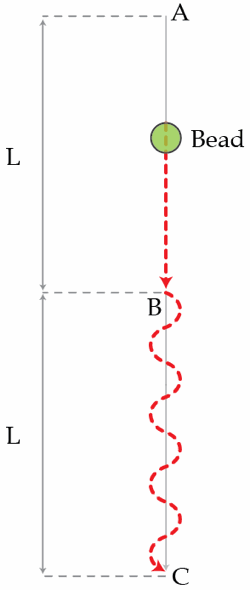
A bead slides down on a frictionless wire, starting from rest at point A. The section from A to B is straight while the section from B to C is wavy (with wavelength λ ≪ L ). The total length of the stretched wire is 3 L . If L = 5 0 cm , determine the time in seconds it takes for the bead to reach point C. Assume that the bead slides smoothly at all times.
Details and assumptions
g = 9 . 8 m / s 2
The answer is 0.584.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
6 solutions
I was thinking more about this problem and felt a little uncomfortable with the level of analysis I gave to my claim about path lengths and accelerations.
Consider two cases, one where we have a constant acceleration over a given path length, and another where we have a varying acceleration over the same overall path length with the same average acceleration as in the constant case.
Let's call the average acceleration a ˉ , then, the path length l for a given time t is found by 2 1 a ˉ t 2 = l .
Let's call the time it takes to travel this path t ∗ .
Then t ∗ = 2 l ∗ / a ˉ .
Now, let's treat the case with a ( t ) constantly varying. It is sufficient to show that if we travel for the same amount of time, we traverse the same path length. The distance traveled is given by
l = 0 ∫ t ∗ d t 1 0 ∫ t 1 d t 2 a ( t 2 ) .
Now, the integral over t 2 is just the sample average of the acceleration multiplied by the duration of the sample interval, i.e.
a ˉ ( T ) = T 1 0 ∫ T d t 2 a ( t 2 )
which very quickly becomes equal to the true average acceleration. For the early T , the average is converging, but after a few cycles the average tends to its true value. Therefore, the difference between
0 ∫ t 1 d t 2 a ( t 2 ) and t 1 a ˉ ( t 1 ) disappears quite rapidly so that the two can be interconverted after a few wavelengths. As λ ≪ L we can safely interconvert in our problem.
Therefore,
l = 0 ∫ t ∗ d t 1 0 ∫ t 1 d t 2 a ( t 2 ) ≈ 0 ∫ t ∗ d t 1 t 1 a ˉ ( t 1 ) = 2 1 a ˉ ( t ∗ ) t ∗ 2 = l ∗
Showing that two paths of the same path length, one of which has a constant acceleration, the second of which has a fluctuating acceleration whose average quickly converges to that of the constant case, are equivalent up to small differences.
I'd like to back off the claim that the path can be interconverted with any path of the same length and drop, but this does provides justification for the remapping onto the inclined plane.
You inverted the explanations up there, into the parentheses.
Log in to reply
...I didn't realize that til you pointed it out. Thanks for keeping me honest!
do we assume normal force b/w wire and bead
The speeds of the bead at B and C respectively are: v 1 = 2 g L and v 2 = 4 g L .
The time it takes for the bead to reach B is: t 1 = g 2 L .
The wavy section BC is totally similar to a straight line with length 2L and height L, so the acceleration of the bead in this section is a = 2 L g L = 2 g .
The time it takes in section BC is: t 2 = a v 2 − v 1 = g L ( 4 − 2 2 ) .
The total times are: t = t 1 + t 2 = g L ( 4 − 2 ) = 0 . 5 8 4 ( s ) .
why is the wavy section totally similar to a triangle? if the acceleration turns out to average around g/2, two trajectories can have the same avg acceleration and yet have different kinematics. also, if this claim is true, why is the whole path (from A to C) not equivalent to a straight line with length 3L and height 2L? we should be able to calculate the whole thing using this analogy?
I didn not get this part: a = gL/2L
We need to treat the two portions of the wire separately, since the accelerations of the bead in each section will be different.
Conservation of energy tells us when the bead is at the end of the straight section, it has speed v 1 = 2 g L . Since it started at rest, and has constant acceleration, its average speed for the straight portion must be half of that value, v a v g = 2 g L and the time to get to that point must be Δ t 1 = v a v g L = g 2 L .
Similarly, conservation of energy tells us when the bead is at the bottom of the wire, it has speed v 2 = 4 g L . Since it began this portion with a speed of v 1 = 2 g L , and has constant acceleration, its average speed over that range must be v a v g = 2 v 1 + v 2 = ( 1 + 2 2 ) g L .
Since the bead travels a distance 2 L with that average speed, the time to cover that length must be Δ t 2 = v a v g 2 L = ( 2 + 2 ) g L 4 L .
The total time is just Δ t 1 + Δ t 2 , which simplifies to ( 2 + 2 4 + 2 ) g L ≈ 0 . 5 8 4 seconds.
I don't think it is correct to say "Since it bagan this portion [wavy portion] with a speed of v 1 ans has constant acceleration ...". It clearly doesn't have a constant acceleration in the wavy portion. However, it might be correct to say that it has a constant average acceleration. Just a subtle thing I wanted to point out. Btw, I like this solution more than others because you are not changing the path of the bead at all, something which I feel is not properly justified.
First, compute the time it takes for the bead to go from A to B : Δ x = − 2 1 g t A B 2 = L ⇒ t A B = g 2 L We know that by the conservation of energy, the velocity ( v ) is related to the height ( h ) of the bead above point C by 2 1 m v 2 + m g h = 2 m L From here we can solve for v and a function of h : v = − 2 g ( 2 L − h ) ( 1 ) Now if the wire were not wavy, then the time ( t 0 ) it would take for the bead to go from B to C can be found by writing ( 1 ) as a differential equation, then separating and integrating: d t d h = − 2 g ( 2 L − h ) ⇒ t 0 = ∫ 0 L 2 g ( 2 L − h ) d h ( 2 ) For the problem at hand, we can still use ( 1 ) but v is not d t d h , it is d t d s where s is the length along the wavy curve that the wire makes. But d h and d s are related: if h changes by a small amount, s changes by twice that amount since the segment of the wire from B to C is compacted to half its normal size. So we have d s = 2 d h Using this result and ( 1 ) , we see that t B C = 2 ∫ 0 L 2 g ( 2 L − h ) d h = 2 t 0 Now we just need to find t 0 . We could evaluate ( 2 ) but I chose to use the kinematic equation: v f = v B + g t 0 We know v f and v B from the conservation of energy. After finding t 0 , doubling it and adding t A B , we get t B C = 2 t 0 + t A B = . 5 8 4 s
Error: There is a missing factor of g in the right hand side of the 3rd equation down. Also in case it's not clear, v f is the velocity of the bead at C and v B is the velocity of the bead at B.
Imagine the race of two balls, ball 1 dropped vertically from rest at A and arrive at B below A height h, and ball 2 dropped from rest at A, roll on a slope of angle θ and arrive at B also below A height h.
t 1 = g 2 h and t 2 = g s i n θ 2 s i n θ h = s i n θ 1 g 2 h
⇒ t 2 = s i n θ t 1 = t 1 l 1 l 2 which l 1 and l 2 are lengths of the path which each balls travelled.
Apply to the problem, we got t ′ = g 2 × 0 . 5 + 2 × ( g 2 × 1 − g 2 × 0 . 5 ) = 0 . 5 8 4 ( s )
Imagine the race of two balls, ball 1 dropped vertically from rest at A and arrive at B below A height h, and ball 2 dropped from rest at A, roll on a slope of angle and arrive at B also below A height h. and which and are lengths of the path which each balls travelled. Apply to the problem, we got Reply Subscribe to comments
Straight section
In the first segment of the wire, the bead is in free fall under the influence of gravity. It takes the bead time
t 1 = 2 L / g (by conservation of energy: 2 1 m v 2 = m g h )
to fall this distance and exits the section with the speed
v 1 = 2 g L (by kinematics: 2 1 g t 2 = L ).
Wavy section
In the wavy section, the key is to recognize that the detailed kinematics of the bead at any moment is irrelevant to the time taken, t 2 .
As the bead moves along the curve of the wire, it is subject to a changing acceleration which varies from g whenever the wire turns around, to g cos θ c where θ c is the angle between the wire and the vertical at the inflection points of the wire's curve.
This, however, does not matter. What does matter is the average acceleration that the bead experiences along the path. Regardless of the path that is actually taken by the bead, it must achieve a vertical drop of length L and travel a path distance of length 2 L which means it must have the same average acceleration as every other path which drops a vertical distance L while traveling a path length 2 L . We can therefore calculate using a simpler path with the same average acceleration.
In particular, we can map the path of the wavy wire onto an inclined plane of height L with hypotenuse of length 2 L .
Now we simply have a particle which enters an inclined plane with some initial velocity v 1 and accelerates along the inclined plane with the acceleration a = g sin θ , with the angle θ given by sin θ = 2 1 → θ = 6 π .
Therefore, we have
v 1 t 2 + 2 1 g sin θ t 2 2 = 2 L
which gives t 2 = g sin θ 4 g L sin θ + v 1 2 − v 1 = g sin θ 4 g L sin θ + 2 g L − 2 g L = 1 6 L / g − 8 L / g
Giving an overall time of
t 1 + t 2 = L / g ( 2 + 1 6 − 8 ) ≈ 0 . 5 8 4 seconds