Beauty of Geometry - 1 (Easier version)
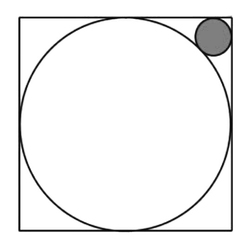 There is a circle inscribed in a square. Inside the square, where the inscribed circle isn't present, we inscribe another small circle (shaded) touching both of the square and the bigger circle.
There is a circle inscribed in a square. Inside the square, where the inscribed circle isn't present, we inscribe another small circle (shaded) touching both of the square and the bigger circle.
If the radius of the bigger circle is 15 cm, then what will be the radius of the smaller circle (in cm)? Round your answer to 3 decimal digit.
You can find the original problem here .
The answer is 2.574.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the radius of the bigger and smaller circle be R & r respectively.
Now, draw a line from the center of the bigger circle to that particular corner of the square which will go through the center of the smaller circle . Then draw 2 more squares as shown below:
As the diagonal of a square is equal to 2 times it's edge, we get, O A + A B + B C = O C ⇒ R + r + 2 r = 2 R ⇒ r = 2 + 1 R ( 2 − 1 ) ⇒ r = R ( 3 − 2 2 )
So the radius of the smaller circle will be, r = 1 5 ( 3 − 2 2 ) = 2 . 5 7 4