Averaging Out Their Differences

Algebra Level 1

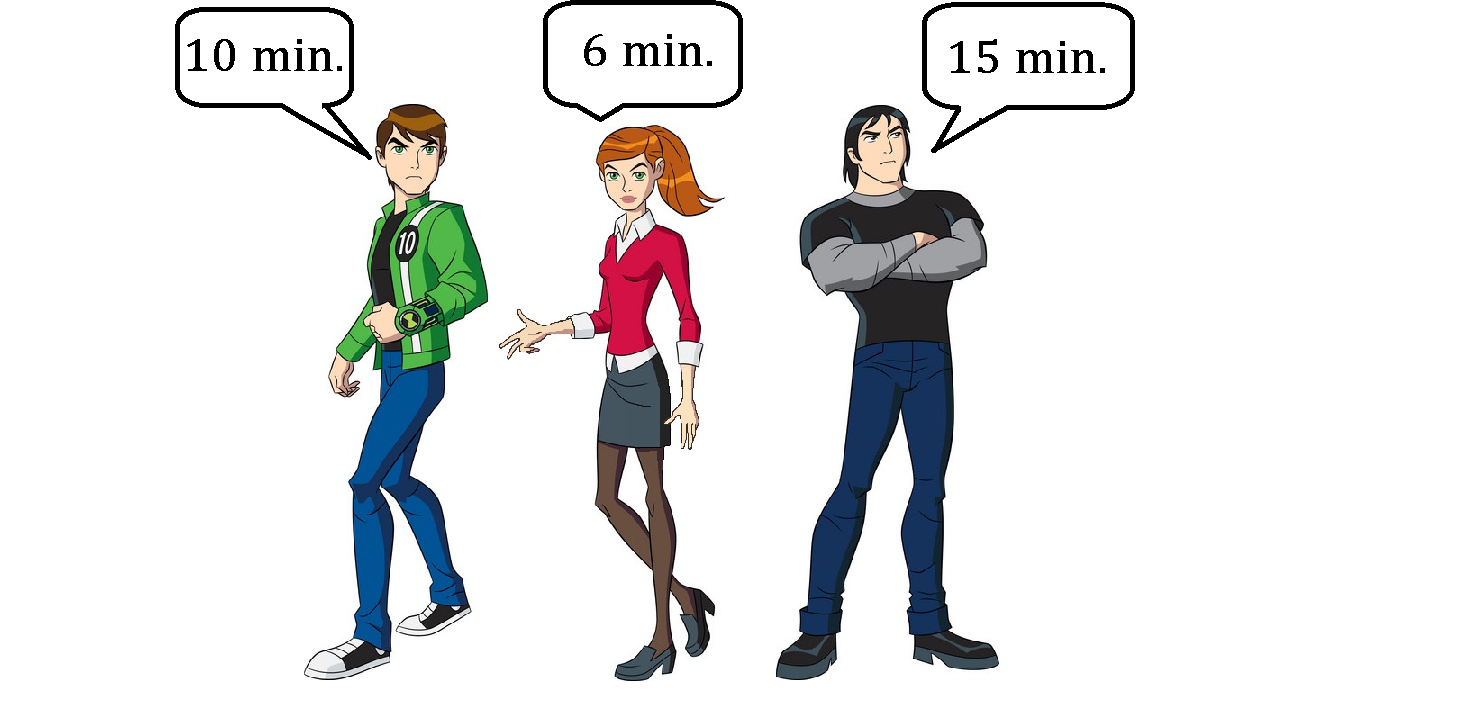
Ben, the ultimate hero, spends 10 minutes to solve a math problem.
The pretty and smarter Gwen only needs 6 minutes to come up with an answer.
Kevin, on the other hand, doesn't attend any school, so he takes 15 minutes for the same task.

What is the average time per person required for this team to solve a math problem?

The answer is 9.

2 solutions

Worranat Pakornrat
Aug 28, 2016

Relevant wiki: Harmonic Mean

To solve this problem, we need to find the harmonic mean within this team:

$H.M. = \dfrac{N}{\sum \dfrac{1}{x}} = \dfrac{3}{\dfrac{1}{6}+\dfrac{1}{10}+\dfrac{1}{15}} = \dfrac{3}{\dfrac{2+3+5}{30}} = \boxed{9}$

Practically speaking, suppose we let the three teenagers $30$ minutes to do their homework. Ben would solve $3$ problems; Gwen $5$ problems; and Kevin only $2$ problems. Overall, the three of them could solve $10$ problems in $30$ minutes; in other words, proportionally they could solve $1$ problem in $3$ minutes.

Clearly, since $\dfrac{1}{3} = \dfrac{1}{9} + \dfrac{1}{9} + \dfrac{1}{9}$ , for the same capacity, each person would need $9$ minutes to solve a math problem, as calculated above.

Why can't we just do like, (6+10+15)÷3=10.33 What is wrong with this thought???

Prayas Rautray - 4 years, 1 month ago

It's not an arithmetic mean, in which time is constant. For example, if you change the question to be something like "Ben did 10 questions in an hour, Gwen 15 in an hour, and Kevin 6 in an hour," then an average will be calculated as you did. However, in this case, time is variable; only the work done is constant. So we have to evaluate the amount of work done in a constant time (half an hour in my solution) and average them out as explained in my solution above.

Hope it's clarified now.

Worranat Pakornrat - 4 years, 1 month ago

Ahhhhhhh my stupid brain. I had the exact same thoughts of the 30 minute scenario thing and interpreted the amount of problems solved in 30 minutes. Next I even added 3+5+2 but then I didn't know where to go next (starting from 10 problems in 30 minutes) mainly because of confusion (for some reason unexplainable). I knew what to do, Ahhh I'm so angry. Haha

Rico Lee - 4 years, 9 months ago

Well, I'm sure you'll figure out next time. ;)

Worranat Pakornrat - 4 years, 9 months ago

Rodrigo Carvalho
Jul 19, 2018

Actually, I didnt get the why in its use. I mean, about the reasoning. Are there any other way o thinking instead of just jumping into the formulae?!

A little late, but imagine you're driving a car and you want to find the average velocity. The first half you travel at a speed v, and the second half you travel at half that speed, v / 2. You can't just find the arithmetic mean, because you spent twice as much time at the speed of v / 2 than v. Imagine, you drive 1 mile in 10 min, and 1 mile in 20 min. If you find the arithmetic mean, it would give you the wrong value because you can imagine that you spent twice as long driving at the second velocity than the first. You can imagine that the 'weight' of the second part is greater than the first, in fact twice as big. If you plug the velocity for x in the harmonic mean formula, it gives you the correct answer. Actually, if you can still solve it using arithmetic mean. Split the 1 mile into 2 1/2 miles. Now you go 1 mile in 10, 1/2 mile in 10 and another 1/2 mile in 10. They are all of the same weight because the time taken is equal. Now we have three terms so we must divide by three instead of two. I'll leave the calculations to you.

A Former Brilliant Member - 2 years, 5 months ago

I didn't understand the usage here either.

Krish Shah - 1 year, 2 months ago

Averaging Out Their Differences

The answer is 9.

2 solutions

0 pending reports