Bigger than a circle
The diagram above shows both a unit circle and a circle-like shape S --slightly larger than the unit circle--defined by the equation 1 − ∣ x ∣ + 1 − ∣ y ∣ = 1 . What is the percentage by which the area of S is bigger than the area of the unit circle?
Give your answer to 3 decimal places.
The answer is 6.1032954.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The larger region closely resembles a superellipse . If you are interested, you can read more about it there. :)
Log in to reply
It is not a superellipse, but gets very close indeed. This inspired a new problem .
This is strange, is there a reason why this curve looks so much like a circle?
By just looking at the first quadrant, the gradient at each point ( X , Y ) can be found (via implicit differentiation ) to be − Y X and − 1 − X 1 − Y for the circle and the non-circle, respectively. But I don't see how that helps....
Log in to reply
The non-circle runs through ( 0 , 1 ) , ( 1 , 0 ) , and ( 0 . 7 5 , 0 . 7 5 ) . The circle runs through ( 0 , 1 ) , ( 1 , 0 ) , and ( 2 1 , 2 1 ) ≈ ( 0 . 7 1 , 0 . 7 1 ) . That suggest some of the closeness.
Moreover, if we consider the polar representation r ( θ ) , we see that r = 1 for the circle, and r varies between 1 and 4 3 2 ≈ 1 . 0 6 1 . This shows how close the two are.
Conjecture. For the non-circle, r ( θ ) is strictly increasing on [ 0 ∘ , 4 5 ∘ ] , and strictly decreasing on [ 4 5 ∘ , 9 0 ∘ ] .
Here is a start of a possible proof: first, from implicit differentiation we have 1 − y d x + 1 − x d y = 0 . From x = r cos θ , y = r sin θ we find d x = r x d r − y d θ ; d y = r y d r + x d θ . Substitute these in the first equation to eliminate d x and d y : ( y 1 − x + x 1 − y ) r d r = ( y 1 − y − x 1 − x ) d θ . If only we can prove that, for points on the non-circle in the first quadrant, y 1 − y > x 1 − x iff 0 < y < x < 1 we have proof.that d r / d θ > 0 iff x < y .
1-|x|=sin⁴(t),1-|y|=cos⁴(t) |x|=1-sin⁴(t), |y|=1-cos⁴(t)
x²+y²=(1-sin⁴(t))²+(1-cos⁴(t))²= 2-2(sin⁴(t)+cos⁴(t))+ sin^8(t)+cos^8(t)= 2-2(1-2sin²(t)cos²(t))+ (1-2sin²(t)cos²(t))²-2sin⁴tcos⁴t= 1+2sin⁴tcos⁴t= 1+sin⁴(2t)/8 So1≤x²+y²≤9/8 so we see it is very near x²+y²=1
Ah parametrization! This is probably the last thing that I would be thinking when solving this problem! Very creative.
Note that you can (slightly) simplify your integral 4 ∫ 0 1 ( 1 − t 2 ) ( 1 − t 4 ) t d t by making the substitution u = t 2 .
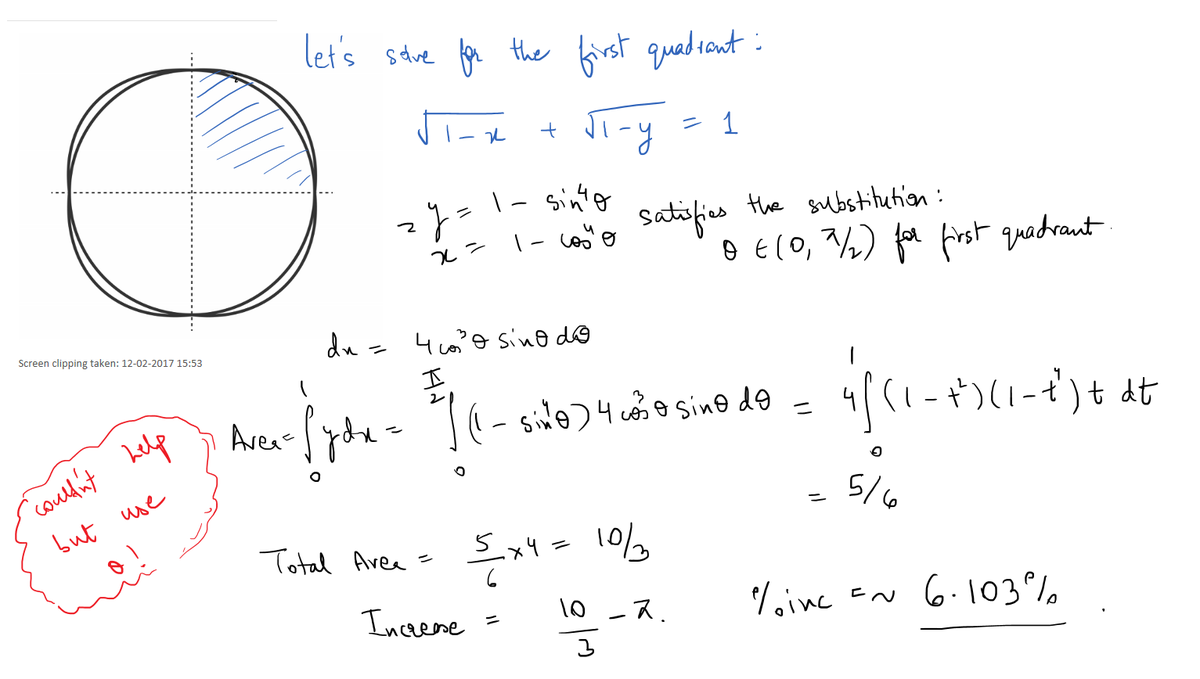
Let's focus on the part of the shape in the first quadrant. We find the area by integration. First express y in terms of x : y = 1 − ( 1 − 1 − x ) 2 = 2 1 − x − 1 + x . The area is ∫ 0 1 y d x = ∫ 0 1 ( 2 1 − x − 1 + x ) d x = [ − 3 4 ( 1 − x ) 3 / 2 − x + 2 1 x 2 ] 0 1 = 3 4 − 1 + 2 1 = 6 5 . This is one-quarter of the entire shape; the total area of shape S is therefore A S = 4 × 6 5 = 3 3 1 . Compare this to the area of the unit circle, A U = π : A U A S = π 3 3 1 = 1 . 0 6 1 0 3 ⟹ 6 . 1 0 3 % .