Bisectors
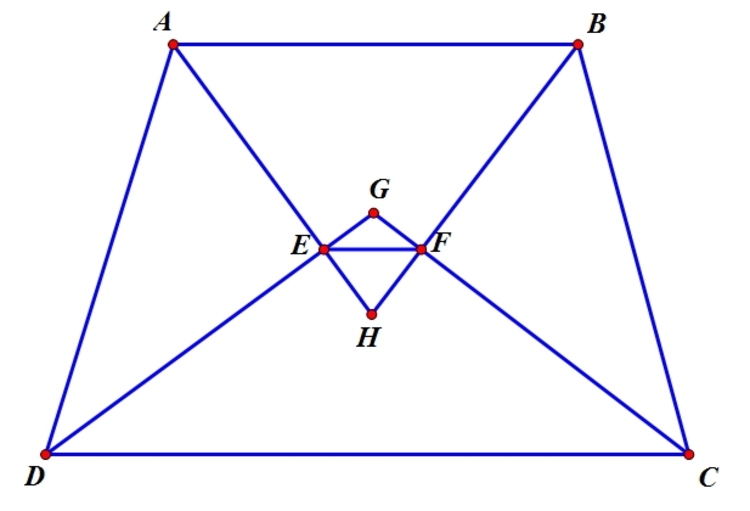 Let
A
B
C
D
be a trapezoid with
A
B
/
/
C
D
. The bisectors of
∠
C
D
A
and
∠
D
A
B
meet at
E
, the bisectors of
∠
A
B
C
and
∠
B
C
D
meet at
F
, the bisectors of
∠
B
C
D
and
∠
C
D
A
meet at G, and the bisectors of
∠
D
A
B
and
∠
A
B
C
meet at
H
. Quadrilaterals
E
A
B
F
and
E
D
C
F
have areas
2
4
and
3
6
, respectively, and triangle
A
B
H
has area
2
5
.
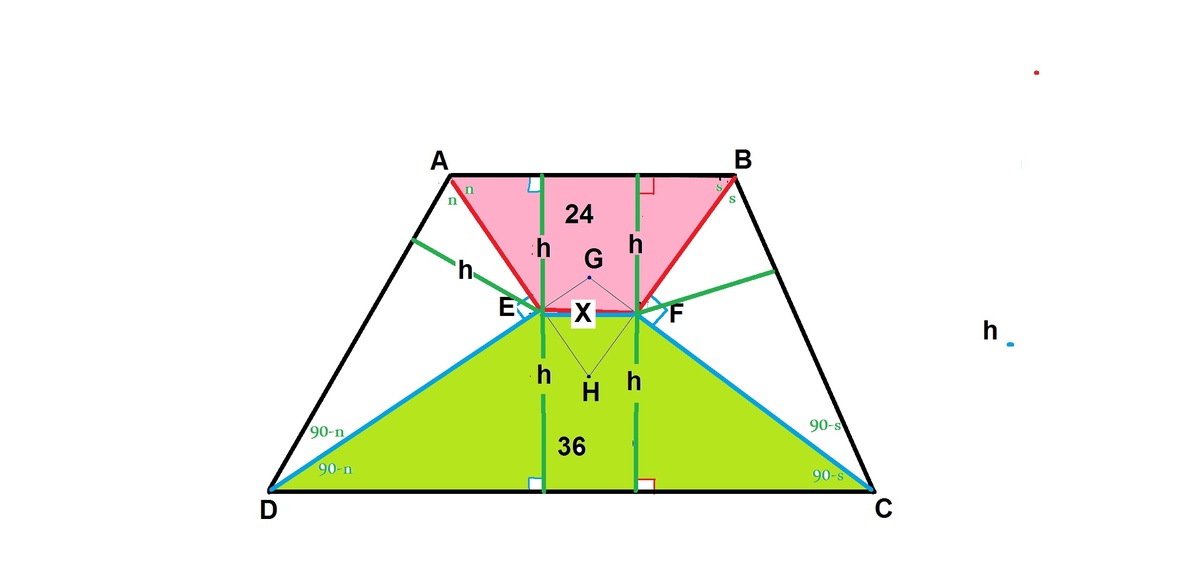
Let
A
B
C
D
be a trapezoid with
A
B
/
/
C
D
. The bisectors of
∠
C
D
A
and
∠
D
A
B
meet at
E
, the bisectors of
∠
A
B
C
and
∠
B
C
D
meet at
F
, the bisectors of
∠
B
C
D
and
∠
C
D
A
meet at G, and the bisectors of
∠
D
A
B
and
∠
A
B
C
meet at
H
. Quadrilaterals
E
A
B
F
and
E
D
C
F
have areas
2
4
and
3
6
, respectively, and triangle
A
B
H
has area
2
5
.
The area of triangle C D G can be expressed as n m , where m , n are coprime positive integers.
Find m + n .
The answer is 263.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
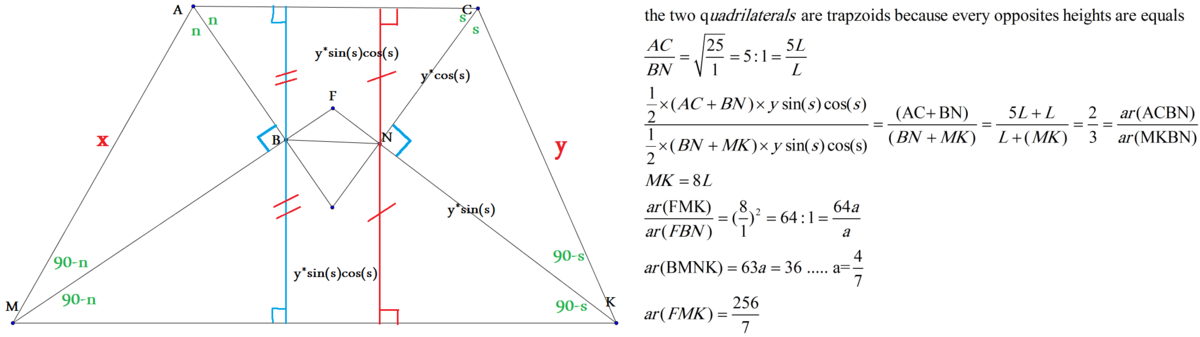
 Because of the four angle bisectors, perpendiculars dropped on each side
are equal to h.
∴
x
=
E
F
=
a
n
d
∣
∣
A
B
a
n
d
C
D
.
⟹
A
E
F
B
a
n
d
E
D
C
F
a
r
e
t
r
a
p
e
z
o
i
d
s
.
A
r
e
a
Δ
E
H
F
=
2
5
−
2
4
=
1
A
r
e
a
s
Δ
E
H
F
Δ
A
H
B
=
1
2
5
=
(
x
A
B
)
2
.
∴
A
B
=
5
x
.
.
.
.
.
.
.
.
.
.
.
.
(
∗
)
A
r
e
a
s
A
E
F
B
E
D
C
F
=
2
4
3
6
=
2
3
=
2
1
∗
h
∗
(
x
+
5
x
)
2
1
∗
h
∗
(
x
+
D
C
)
.
.
.
.
(
∗
∗
)
∴
D
C
=
8
x
.
.
.
.
(
∗
∗
∗
)
Δ
s
G
D
C
=
E
D
C
F
+
G
E
F
.
∴
A
r
e
a
s
G
E
F
G
D
C
=
G
E
F
3
6
+
G
F
F
=
(
x
2
(
8
x
)
2
)
S
o
l
v
i
n
g
a
r
e
a
G
E
F
=
7
4
.
⟹
A
r
e
a
G
E
C
=
3
6
+
7
4
.
=
7
2
5
6
=
n
m
.
m
+
n
=
.
.
.
.
.
.
.
2
6
3
Because of the four angle bisectors, perpendiculars dropped on each side
are equal to h.
∴
x
=
E
F
=
a
n
d
∣
∣
A
B
a
n
d
C
D
.
⟹
A
E
F
B
a
n
d
E
D
C
F
a
r
e
t
r
a
p
e
z
o
i
d
s
.
A
r
e
a
Δ
E
H
F
=
2
5
−
2
4
=
1
A
r
e
a
s
Δ
E
H
F
Δ
A
H
B
=
1
2
5
=
(
x
A
B
)
2
.
∴
A
B
=
5
x
.
.
.
.
.
.
.
.
.
.
.
.
(
∗
)
A
r
e
a
s
A
E
F
B
E
D
C
F
=
2
4
3
6
=
2
3
=
2
1
∗
h
∗
(
x
+
5
x
)
2
1
∗
h
∗
(
x
+
D
C
)
.
.
.
.
(
∗
∗
)
∴
D
C
=
8
x
.
.
.
.
(
∗
∗
∗
)
Δ
s
G
D
C
=
E
D
C
F
+
G
E
F
.
∴
A
r
e
a
s
G
E
F
G
D
C
=
G
E
F
3
6
+
G
F
F
=
(
x
2
(
8
x
)
2
)
S
o
l
v
i
n
g
a
r
e
a
G
E
F
=
7
4
.
⟹
A
r
e
a
G
E
C
=
3
6
+
7
4
.
=
7
2
5
6
=
n
m
.
m
+
n
=
.
.
.
.
.
.
.
2
6
3