Breaking The Rod!!
 A wooden rod is 6 m long. 2 points A and B on the rod are uniformly and independently chosen. The rod is then cut at both points to obtain a smaller rod AB.
A wooden rod is 6 m long. 2 points A and B on the rod are uniformly and independently chosen. The rod is then cut at both points to obtain a smaller rod AB.
Find the chance that the rod AB will be at least 1m in length. If the chance can be written as b a , where a , b are positive coprime integers, find a + b .
Image credit: Adapated from Wikipedia ArnoldReinhold .
The answer is 61.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
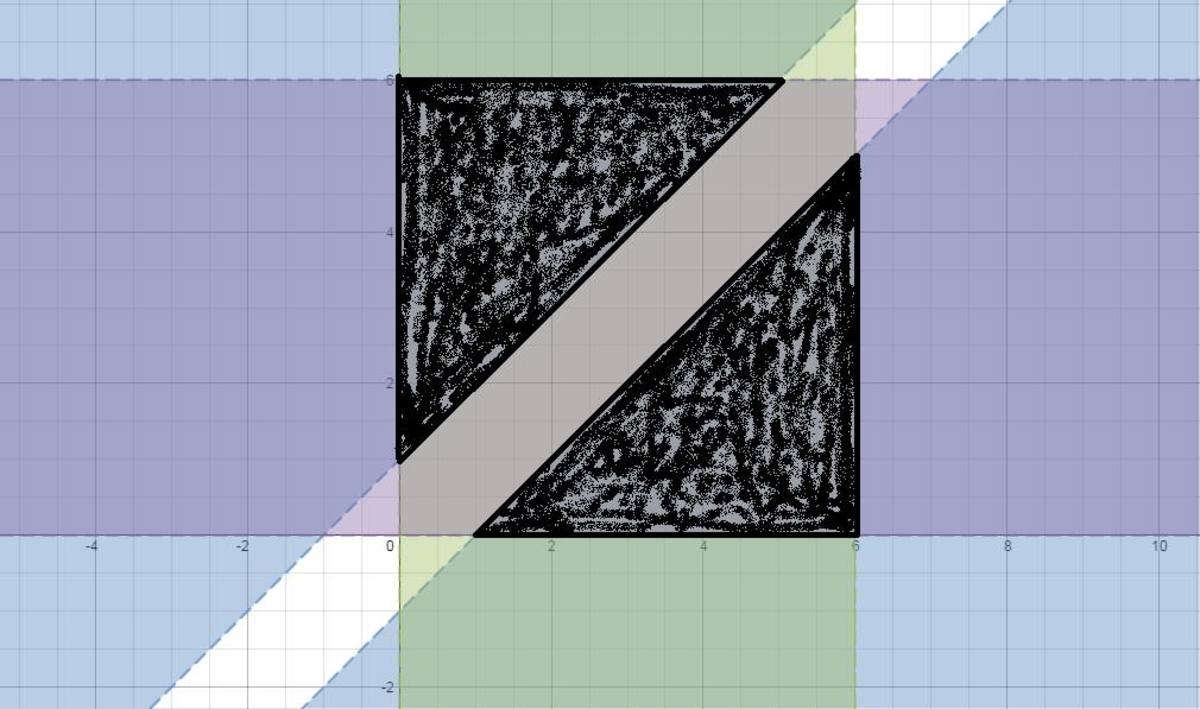
This Is the Graph Of ∣ x − y ∣ ≤ 1 Where x , y ϵ [ 0 , 6 ]
And The Shaded region Divided by the total region is the Probability.
Log in to reply
I used the same method. Desmos is so helpful.
I did the same, but I think question is not correctly phrased. For example, if you actually imagine the procedure of cutting, then imagine that we first on the left side at point A . Now not all the points from 0 to 6 are accessible for B and hence not both triangles exist in reality, only one of them does. Am I missing something?
Log in to reply
@VrajMehta you should mention that the cut at a random point A is made first, and then a second cut at another random point B is made, or vice versa. I mean that you need the two cuts to be distinguishable.
Log in to reply
Calvin edited the problem now. So everything is fine.
Let us call the first point A , and its distance from left end of the rod x .
First case - 0 < x < 1
In this case the required probability is ∫ 0 1 3 6 5 − x d x = 8 1 .
Second case - 1 < x < 5
In this case the required probability is ∫ 1 5 3 6 4 d x = 9 4 .
Third case - 5 < x < 6
In this case the required probability is ∫ 5 6 3 6 x − 1 d x = 8 1 .
Therefore, our final probability is the sum of the probabilities in all the three cases, IE,
8 1 + 9 4 + 8 1 = 3 6 2 5 .
Can you explain your method?
Classic solution man.....
Imagine a square of side length 6 . Each point ( x , y ) in the square corresponds to the distance of A and B from the left of the rod (i.e. A is x metres away and B is y metres away). The set of points for which AB is at least 1 m corresponds to two right angle isosceles triangles at the bottom right and top left of the square, of side length 6 − 1 = 5 . The probability is thus 6 × 6 2 ( 0 . 5 ) ( 5 ) ( 5 ) = 3 6 2 5 . The answer is thus 2 5 + 3 6 = 6 1