Brilliant Gamees - Quarter Quell 4
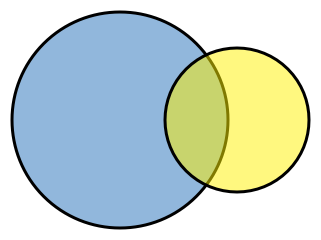In the above figure of 2 overlapping circles, the area of the overlapped region is
of the small circle's area, and
of the large circle's area.
What is the ratio of the area of the large circle to the small circle?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Assume that the overlapped region had an area of x . Then, the large circle has an area of 5 x and the small circle has an area of 4 x . The ratio of their areas would be 5 : 4 .