Brilliant Geometry puzzle 》》 Rectangle puzzle
 brilliant geometry puzzle
brilliant geometry puzzle
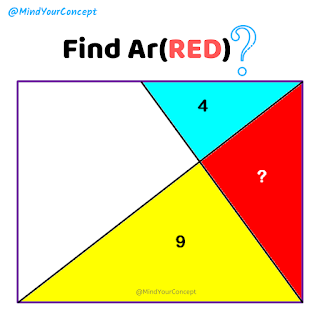
In the rectangle shown above, the areaa of the yellow and blue regions are given as 9 and 4 respectively. Find the area of red region.
You can use Swaroop's theorem to solve 》 bit.ly/Swaroop-theorem
The answer is 6.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
We note that the blue and yellow triangles are similar. Therefore that areas of the two triangles are proportional to the square of their linear dimensions. That is if the blue triangle has a base of 2 a and height of 2 h , the yellow triangle will have a base of 3 a and height of 3 h . Since 2 4 a h = 4 and 2 9 a h = 9 , a h = 2 .
Let the area of the red region be A . Then A + 9 = 2 1 ( 3 a ) ( 5 h ) = 2 1 5 a h = 1 5 ⟹ A = 1 5 − 9 = 6 .