Brothers of Medians

Geometry Level 5

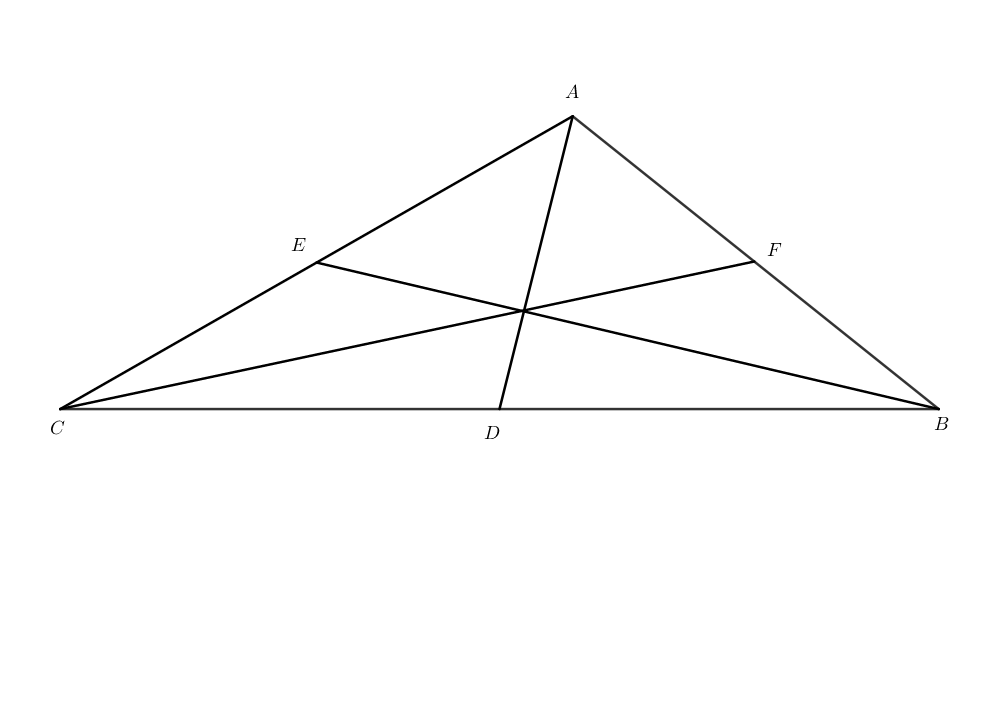
In $\Delta ABC$ ─

$D$ , $E$ and $F$ are interior points on side segments $BC$ , $AC$ and $AB$ .

$\frac{AE}{CE} = \frac{CD}{BD} = \frac{BF}{AF} = r$ , where $r$ is a real number.

$AD$ , $BE$ and $CF$ are concurrent at point $G$ with $\frac{AG}{DG} = \frac{BG}{EG} = \frac{CG}{FG}$ .

The sum of all possible values of $r$ can be expressed as $\frac{a\sqrt{c}}{b}$ , where $c$ is a square-free positive integer, $a$ and $b$ are two coprime positive integers.

Find $(a+b+c)$ .

The answer is 3.

1 solution

Digi Verse
Jan 1, 2018

Using Ceva's Theorem, $\frac{AE}{CE} * \frac{CD}{BD} * \frac{BF}{AF} = 1$ . As all the terms are equal to r, we then get: $r^3=1$ . Therefore, r must be 1. Thus, $a=b=c=1$ , and $a+b+c=3$

I didn't know this nice theorem. Thanks!

I have two solutions completely based on more elementary ideas, and yes, they are much longer too. One is with Vector Approach, another is with Coordinate Geometry.

Muhammad Rasel Parvej - 3 years, 5 months ago

Brothers of Medians

The answer is 3.

1 solution

0 pending reports