Calculate perimeter of a circle

Geometry Level 2

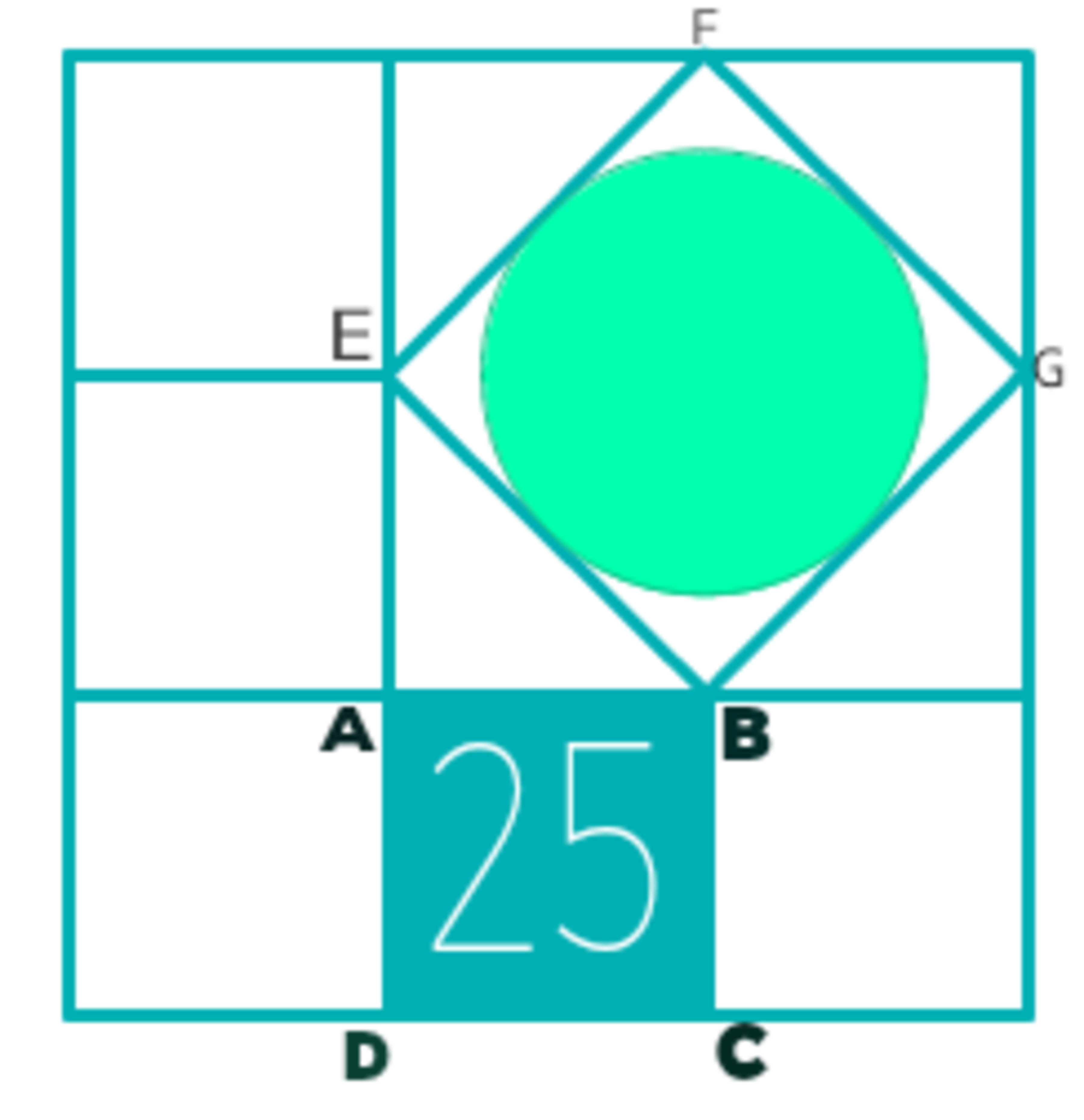
The above shows a figure that has a 2 squares ABCD and BGFE inside of it. A circle is also inscribed inside the square BGFE.

Given that the square ABCD has area 25, and that AB and AE are equal in distance and perpendicular to each other.

What is the circumference of the circle to the nearest integer?

Note: Use $\frac{22}7$ as an approximation for $\pi$ .

39 22 28 20

1 solution

Jahangir Hossain
Dec 29, 2016

given that square ABCD has the area of 25 cm^2

so, AB=√(25)

       =5 cm

AB=AE=5 now EG=5+5 =10

so, We got a diagonal of big square is 10. if EF is a one arms of this bigger square then, √2EF=10 so EF = 10/(√2) =7.071

that is Diameter of the circle is, 2r=7.071 when Redius is r. now the perimeter of the circle is=2πr =2r $\frac{22}{7}$ =7.071 $\frac{22}{7}$ =22.22 so, Answer is 22.22

From your problem, it must be "square", not squire. ................ which has an area of 25 cm^2. From your problem again, we do not know that AB is equal to EA. EB = EF = √(5^2 + 5^2) = √50 = √(25*2) = 5√2 so P = (22/7)(5√2) = 22.22 cm^2

A Former Brilliant Member - 4 years, 5 months ago

Problem has been edited. Thanks a lot.

Jahangir Hossain - 4 years, 5 months ago

show my solution. Answer is same. There's a little logic to understand the the diagonal of bigger square. But both you and mine answer is same.

Jahangir Hossain - 4 years, 5 months ago

You should remove the non-integer from the solutions as you ask for the closest integer in the question.

Kevin Hinson - 4 years, 5 months ago

Calculate perimeter of a circle

1 solution

0 pending reports