Calculative or not?
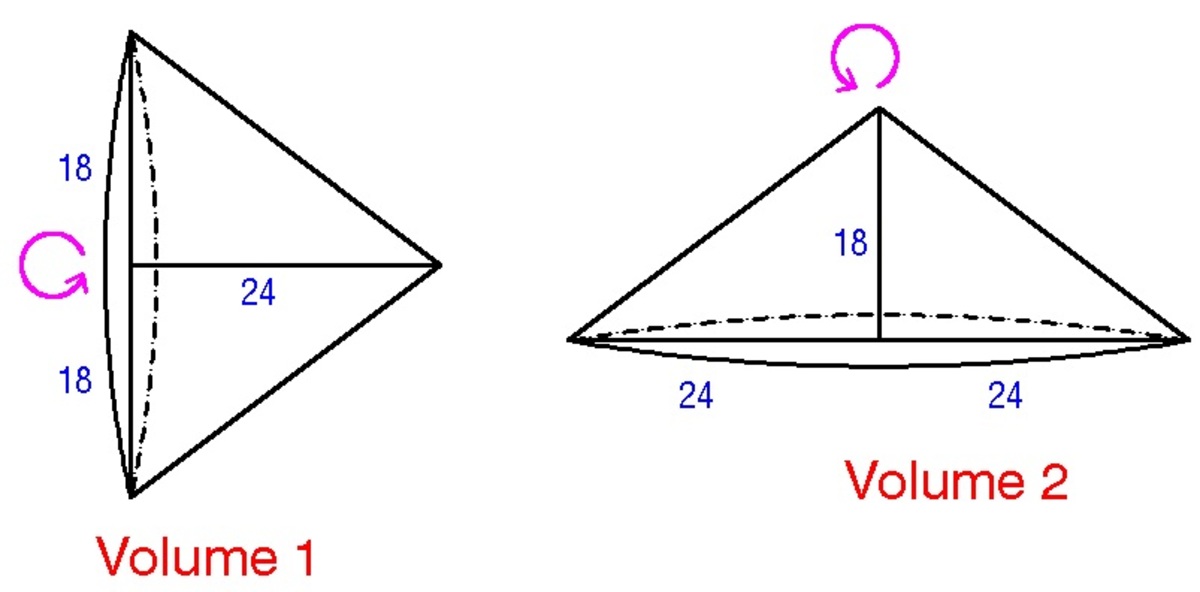
A right triangle, whose base is 24 and height is 18, is made to revolve along its base and height respectively. Find the ratio of volumes of the two solids so obtained.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
After revolving, it forms two regular cones.
 Solving for their volumes
Solving for their volumes
V 1 = 3 1 π ( 1 8 ) 2 ( 2 4 ) = 3 1 π ( 7 7 6 6 )
V 2 = 3 1 π ( 2 4 ) 2 ( 1 8 ) = 3 1 π ( 1 0 3 6 8 )
Solving for the ratio of their volumes
V 2 V 1 = 1 0 3 6 8 7 7 7 6 = 4 3
 G
i
v
e
n
a
r
i
g
h
t
△
w
i
t
h
b
a
s
e
=
2
4
a
n
d
h
e
i
g
h
t
=
1
8
G
i
v
e
n
a
r
i
g
h
t
△
w
i
t
h
b
a
s
e
=
2
4
a
n
d
h
e
i
g
h
t
=
1
8
A f t e r r e v o l v i n g t h e △ a l o n g e i t h e r o f i t s a r m s , w e w i l l o b t a i n a C O N E
C a s e 1 :
R a d i u s o f c o n e = h e i g h t o f △ = 1 8
H e i g h t o f c o n e = b a s e o f △ = 2 4
∴ V o l u m e ( V 1 ) = 3 1 π ( 1 8 ) 2 × 2 4
C a s e 2 :
R a d i u s o f c o n e = b a s e o f △ = 2 4
H e i g h t o f c o n e = h e i g h t o f △ = 1 8
∴ V o l u m e ( V 2 ) = 3 1 π ( 2 4 ) 2 × 1 8
⇒ V o l u m e R a t i o = V 2 V 1 = 3 1 π ( 2 4 ) 2 × 1 8 3 1 π ( 1 8 ) 2 × 2 4 = 4 3
∴ V o l u m e R a t i o = 3 : 4
If the base and height of the right triangle be b and h respectively then, The volume when the triangle is rotated about its base will be = 1/3πh^2b. And the volume when the triangle is rotated about its height will be = 1/3πb^2h. Hence the ratio of the volumes will be = h/b. In this case, the ratio of volumes will be = 18/24 = 3/4. So it is not calculative.