Can It Be Balanced?

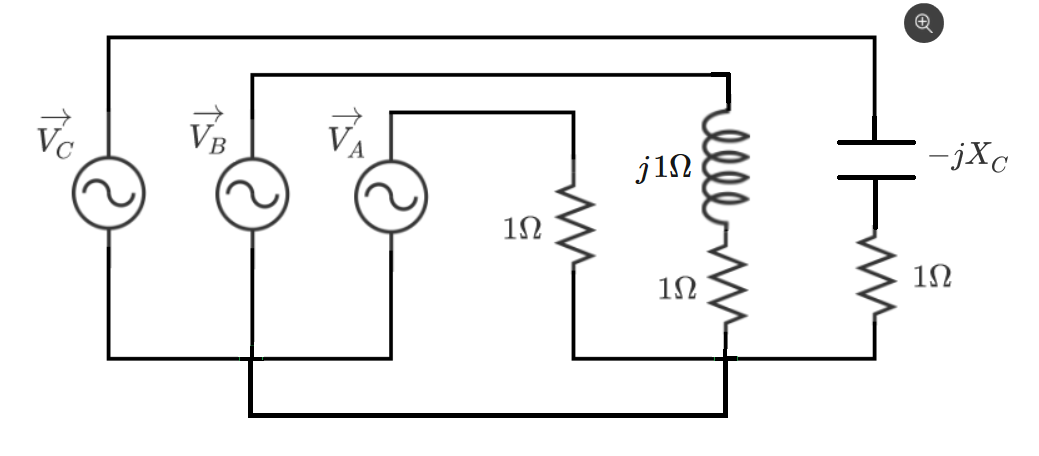
Electricity and Magnetism Level pending

In this circuit the voltages are a balanced three phase set.

V A ⃗ = 1 ∠ 0 ∘ V B ⃗ = 1 ∠ − 120 ∘ V C ⃗ = 1 ∠ 12 0 ∘ \vec{V_A} = 1 \angle 0^\circ \\ \vec{V_B} = 1 \angle {-120}^\circ \\ \vec{V_C} = 1 \angle 120^\circ

Is there any value of capacitive reactance ( X C ) (X_C) that can cause the current flowing in the neutral conductor to be zero?

Yes No

Steven Chase by Steven Chase , 37, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Steven Chase
May 16, 2018

Zero neutral current is equivalent to the following:

V A ⃗ 1 + V B ⃗ 1 + j + V C ⃗ Z C ⃗ = 0 1 ∠ 0 ∘ 1 + 1 ∠ − 120 ∘ 1 + j + 1 ∠ 12 0 ∘ Z C ⃗ = 0 \large{\frac{\vec{V_A}}{1} + \frac{\vec{V_B}}{1 + j} + \frac{\vec{V_C}}{\vec{Z_C}} = 0 \\ \frac{1 \angle 0^\circ}{1} + \frac{1 \angle {-120}^\circ}{1 + j} + \frac{1 \angle 120^\circ}{\vec{Z_C}} = 0 }

Solving for Z C ⃗ \vec{Z_C} yields:

Z C ⃗ ≈ 2.732 ∠ − 3 0 ∘ \large{\vec{Z_C} \approx 2.732 \angle -30^\circ}

Since the resistive portion of the required Z C ⃗ \vec{Z_C} is not equal to 1 1 , no value of capacitive reactance can make the neutral current zero.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...