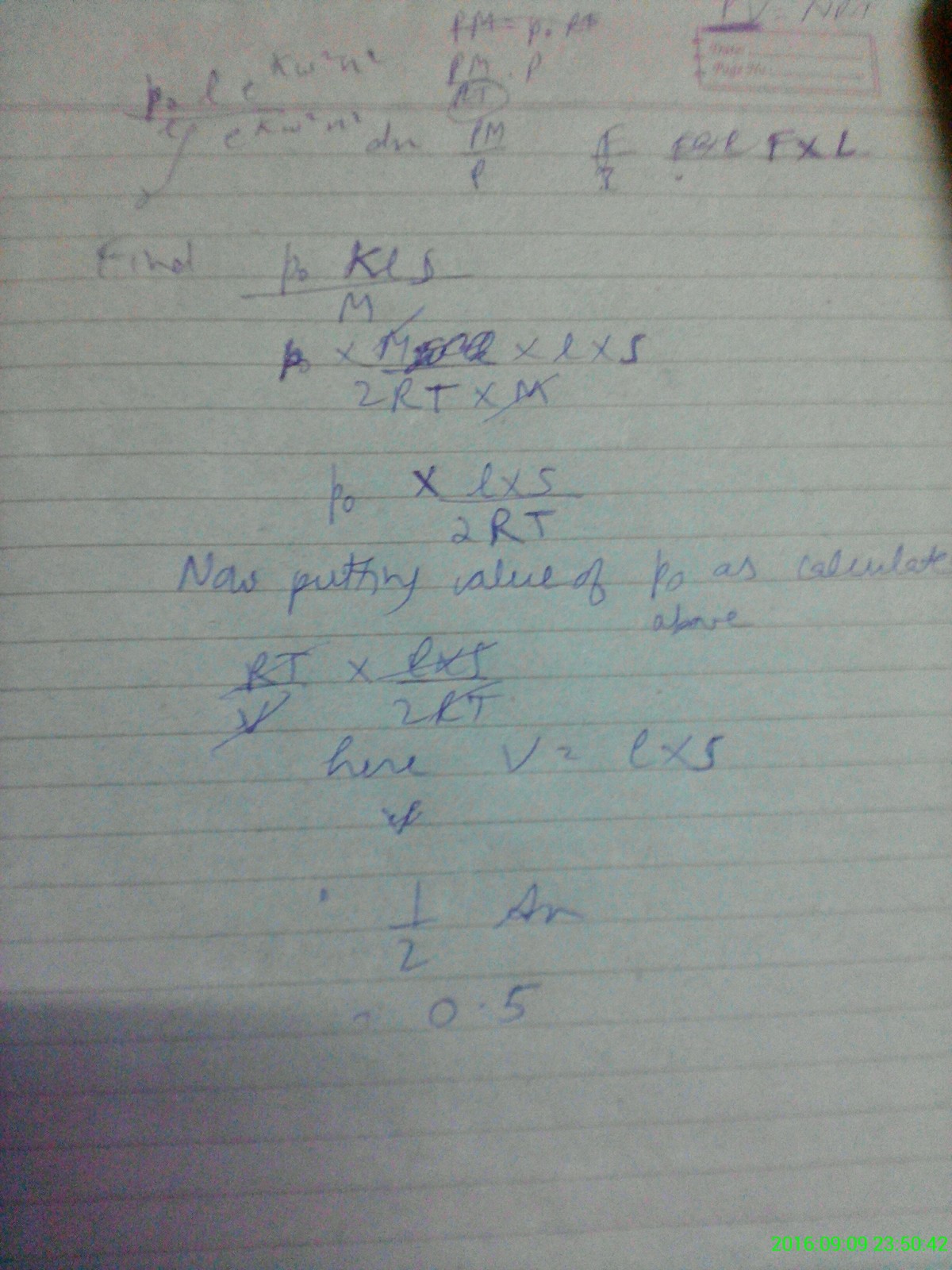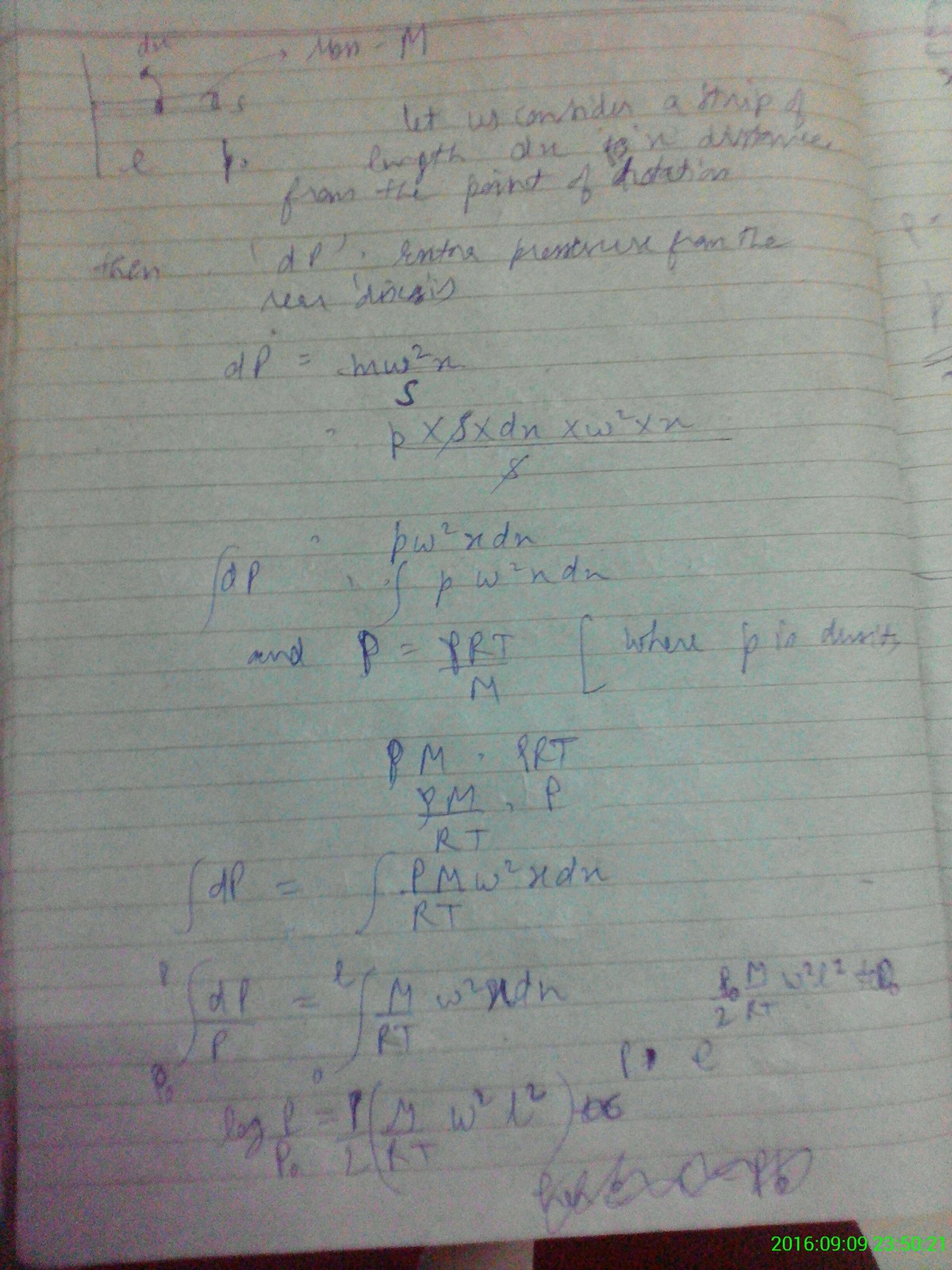Can it be practically evaluated?

A narrow horizontal cylindrical tube is filled with a gas. The tube rotates in a horizontal plane with an angular velocity $w$ about a perpendicular axis through one of its ends A. The length of the tube is $l$ and cross sectional area is $S$ . Total mass of contained gas is $M$ . When the tube was at rest initially, it had a pressure ${ p }_{ o }$ and a temperature $T$ .

Assuming that the temperature remained constant, find the Pressure in the tube as a function of distance x from the end A.

If $\large p=\dfrac { { { p }_{ o }l{ e }^{ k{ w }^{ 2 }{ x }^{ 2 } } } }{ \int _{ 0 }^{ l }{ { e }^{ k{ w }^{ 2 }{ x }^{ 2 } } \, dx } }$ for some constant $k$ , find $\dfrac { { p }_{ o }klS }{ M }$

We've got more for you at the set

Mayank and Akul