Can you help Mr Einstein V. Vector solve his Vector Problem.
 Mr Einstein V. Vector has a problem that he could not solve can you help him solve it.
Mr Einstein V. Vector has a problem that he could not solve can you help him solve it.
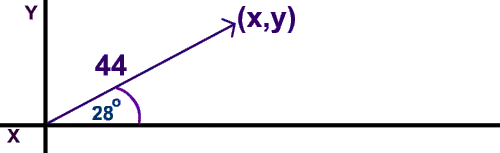
Problem : The magnitude of the resultant of two vectors of the same magnitude is equal to the magnitude of either vector. Find the angle between the two vectors.
If your answer is 65.7555 degrees select 65.7.
The answer is 120.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
R = A 2 + B 2 + 2 A B c o s θ A s B = A a n d w e c a n a s s u m e t h a t R = A ⇒ A = A 2 + A 2 + 2 A 2 c o s θ = 2 A 2 ( 1 + c o s θ ) ⇒ A 2 = 2 A 2 ( 1 + c o s θ ) ⇒ c o s θ = 2 − 1 ⇒ θ = 1 2 0 o