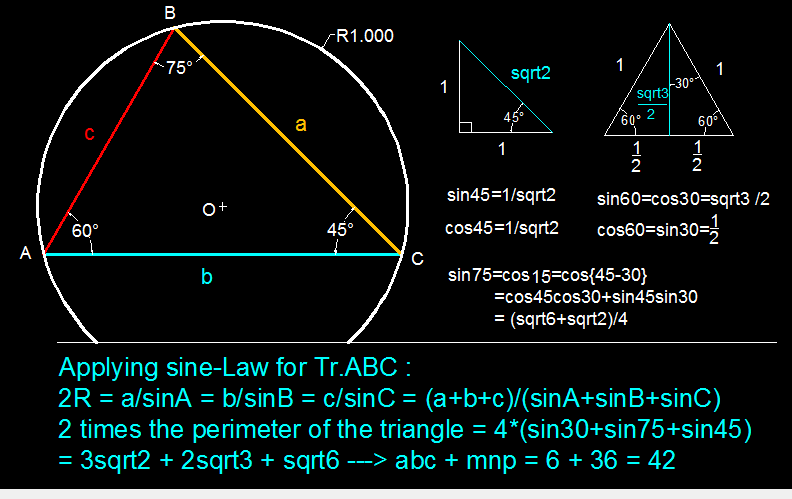
Can you solve without trig?

Triangle $ABC$ inscribed a circle $(O;1)$ . If $\angle A = 60 ^\circ$ and $\angle C = 45 ^\circ$ , 2 times the perimeter of the triangle can be written as $a \sqrt{m} + b\sqrt{n} + c\sqrt{p}$ , where $a,b,c,m,n,p$ are positive integers and $m,n,p$ are distinct squarefrees. Find $abc+mnp$ .

Note: 'Cause I don't like trigonometry, please post solutions using only trigonometric functions of these angles: $30 ^\circ,45 ^\circ,60 ^\circ$

Inspired by my math exercise.