Warm up problem: Cartesian Right Triangle 2 (harder)

Geometry Level 4

here is a link to the easier one

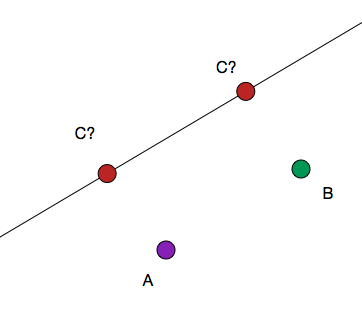
Given point A=(1,6) and B=(4,12) and line D=3x+7, a point C is placed on line D such that $\triangle ABC$ is a right triangle. If the sum of all possible x-coordinates of c can be represented by $\dfrac{p}{q}$ . Find p+q.

Tip: the right angle doesn't necessarily occur at A or B.

The answer is 319.

1 solution

Brian Charlesworth
Oct 3, 2014

Using vectors and scalar dot products is probably the easiest way to approach this problem.

We will have $4$ possible scenarios for point $C(x, 3x + 7)$ , (noting that by, for example, $<AC>$ I mean the vector from $A$ to $C$ and by " $\cdot$ " I mean the dot product operation):

(i), (ii): $<AC>\cdot<BC> = 0$

$\Longrightarrow <x - 1, 3x + 1>\cdot<x - 4, 3x - 5> = 0$

$\Longrightarrow (x - 1)(x - 4) + (3x + 1)(3x - 5) = 0$

$\Longrightarrow 10x^{2} - 17x - 1 = 0$ ,

the two roots of which add to $\frac{17}{10}$ .

(iii) $<AC> \cdot <AB> = 0$

$\Longrightarrow <x - 1, 3x + 1> \cdot <3,6> = 0$

$\Longrightarrow 3x - 3 + 18x + 6 = 0 \Longrightarrow 21x = -3 \Longrightarrow x = -\frac{1}{7}$ .

(iv) $<BC> \cdot <AB> = 0$

$\Longrightarrow <x - 4, 3x - 5> \cdot <3,6> = 0$

$\Longrightarrow 3x - 12 + 18x - 30 = 0 \Longrightarrow x = 2$ .

So the sum of all possible $x$ -coordinates will be

$\frac{17}{10} - \frac{1}{7} + 2 = \frac{249}{70}$ .

Thus $p = 249, q = 70$ and $p + q = \boxed{319}$ .

You're too smart... I just used simple analytical geom. But great solution nonetheless.

Trevor Arashiro - 6 years, 8 months ago

Thanks. Yes, there are a few ways to approach this problem, but since I hadn't used vectors for a while I just went with this method. Good question; thanks for posting it. :)

Brian Charlesworth - 6 years, 8 months ago

Warm up problem: Cartesian Right Triangle 2 (harder)

here is a link to the easier one

The answer is 319.

1 solution

0 pending reports